What is the square of 14?
196
198
200
202
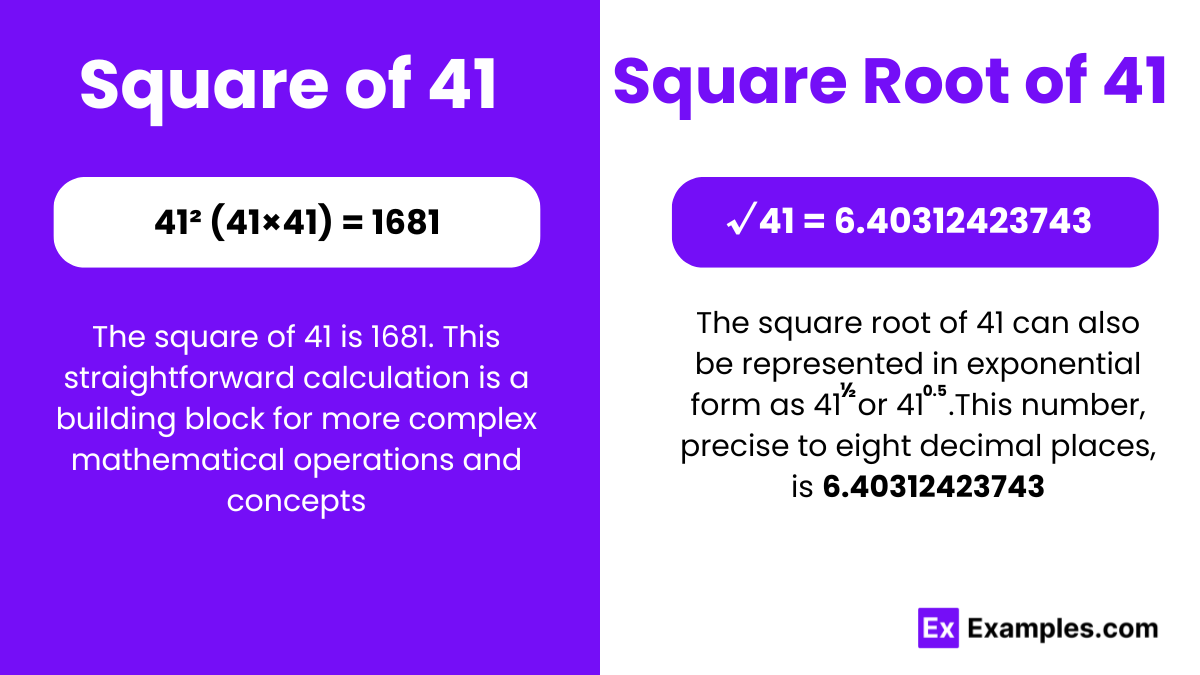
So, the square of 41 is 1681.
Geometrically, if you have a square with each side measuring 41 units, the total area enclosed by the square will be 1681 square units.
Understanding the square of 41 is important in various mathematical contexts, including geometry, algebra, and arithmetic. It finds applications in calculating areas, volumes, distances, and solving mathematical problems.
or
√41=6.403 up to three places of decimal
The square root of 41 (√41) is an irrational number. It cannot be represented as a fraction of two integers, and its decimal representation goes on infinitely without repeating.
Approximately, √41 is around 6.40312423743.
In mathematical terms, √41 represents a number that, when multiplied by itself, equals 41.
Since 41 is not a perfect square (the square of an integer), its square root cannot be expressed as a simple fraction or terminating decimal. Therefore, it is classified as an irrational number.
Exponential Form: 41^½ or 41^0.5
Radical Form: √41
The square root of 41 is an irrational number.
Examples of rational numbers include 1/2, -3, and 5.
Since 41 is not a perfect square, the square root of 41 cannot be expressed as a rational number. Additionally, the decimal representation of √41 is non-repeating and non-terminating. Therefore, √41 is classified as an irrational number.
Examples of irrational numbers include √2, π (pi), and √3.
The square root of 41 (√41) is an irrational number. An irrational number is a real number that cannot be expressed as a simple fraction, and its decimal representation goes on infinitely without repeating. Since 41 is not a perfect square, its square root cannot be expressed as a fraction of two integers. Additionally, the decimal representation of √41 continues infinitely without repeating, indicating its irrational nature. Therefore, the square root of 41 (√41) is classified as an irrational number.
There are several methods to approximate the value of the square root of 41 (√41). Here are some common ones:
Estimation Method:
Long Division Method:
Newton’s Method:
Using a Calculator or Software:
A perfect square is a number that can be expressed as the square of an integer. In other words, it is the product of an integer multiplied by itself. For example, 25 is a perfect square because it equals 5×5.
However, 41 cannot be expressed as the product of an integer multiplied by itself. Therefore, it is not a perfect square.
The square root of 41 (√41) is an irrational number, approximately equal to 6.40312423743. It cannot be expressed as a fraction and its decimal representation is non-terminating and non-repeating.
Various methods can be used, such as estimation, long division, or iterative algorithms like Newton’s method. These methods involve iteratively refining an initial guess to approximate the value of √41.
No, the decimal representation of the square root of 41 does not follow a repeating or terminating pattern. The digits continue infinitely without a discernible repetition.

41² (41×41) = 6.40312423743
So, the square of 41 is 1681.
Geometrically, if you have a square with each side measuring 41 units, the total area enclosed by the square will be 1681 square units.
Understanding the square of 41 is important in various mathematical contexts, including geometry, algebra, and arithmetic. It finds applications in calculating areas, volumes, distances, and solving mathematical problems.
√41 = 6.40312423743
or
√41=6.403 up to three places of decimal
The square root of 41 (√41) is an irrational number. It cannot be represented as a fraction of two integers, and its decimal representation goes on infinitely without repeating.
Approximately, √41 is around 6.40312423743.
In mathematical terms, √41 represents a number that, when multiplied by itself, equals 41.
Since 41 is not a perfect square (the square of an integer), its square root cannot be expressed as a simple fraction or terminating decimal. Therefore, it is classified as an irrational number.
Square Root of √41= 6.40312423743
Exponential Form: 41^½ or 41^0.5
Radical Form: √41
The square root of 41 is an irrational number.
Rational numbers are those that can be expressed as a fraction of two integers, where the denominator is not zero. In other words, they can be written in the form a/b, where a and b are integers and b is not equal to zero.
Examples of rational numbers include 1/2, -3, and 5.
An irrational number is a real number that cannot be expressed as a simple fraction, and its decimal representation goes on infinitely without repeating.
Since 41 is not a perfect square, the square root of 41 cannot be expressed as a rational number. Additionally, the decimal representation of √41 is non-repeating and non-terminating. Therefore, √41 is classified as an irrational number.
Examples of irrational numbers include √2, π (pi), and √3.
The square root of 41 (√41) is an irrational number. An irrational number is a real number that cannot be expressed as a simple fraction, and its decimal representation goes on infinitely without repeating. Since 41 is not a perfect square, its square root cannot be expressed as a fraction of two integers. Additionally, the decimal representation of √41 continues infinitely without repeating, indicating its irrational nature. Therefore, the square root of 41 (√41) is classified as an irrational number.
There are several methods to approximate the value of the square root of 41 (√41). Here are some common ones:
Estimation Method:
Start by finding the nearest perfect squares around 41. In this case, the nearest perfect squares are √36 = 6 and √49 = 7.
Since 41 is closer to 49, start with an initial estimate of √41 ≈ 6.5.
Refine the estimate iteratively using trial and error until you reach a satisfactory approximation.
Long Division Method:
Use the long division method to approximate the square root of 41 manually.
Start with an initial guess, such as 6, and proceed with division and adjustment until you achieve the desired accuracy.
This method involves a series of steps of trial and error to converge on an approximation of √41.
Newton’s Method:
Apply Newton’s method, an iterative algorithm, to approximate the square root of 41.
This method involves refining an initial guess through successive iterations until reaching a sufficiently accurate approximation.
Newton’s method is more efficient but requires a deeper understanding of calculus and iterative algorithms.
Using a Calculator or Software:
Utilize a scientific calculator or mathematical software to directly calculate the square root of 41.
Input 41 into the calculator or software, and the result will provide the accurate value of √41.
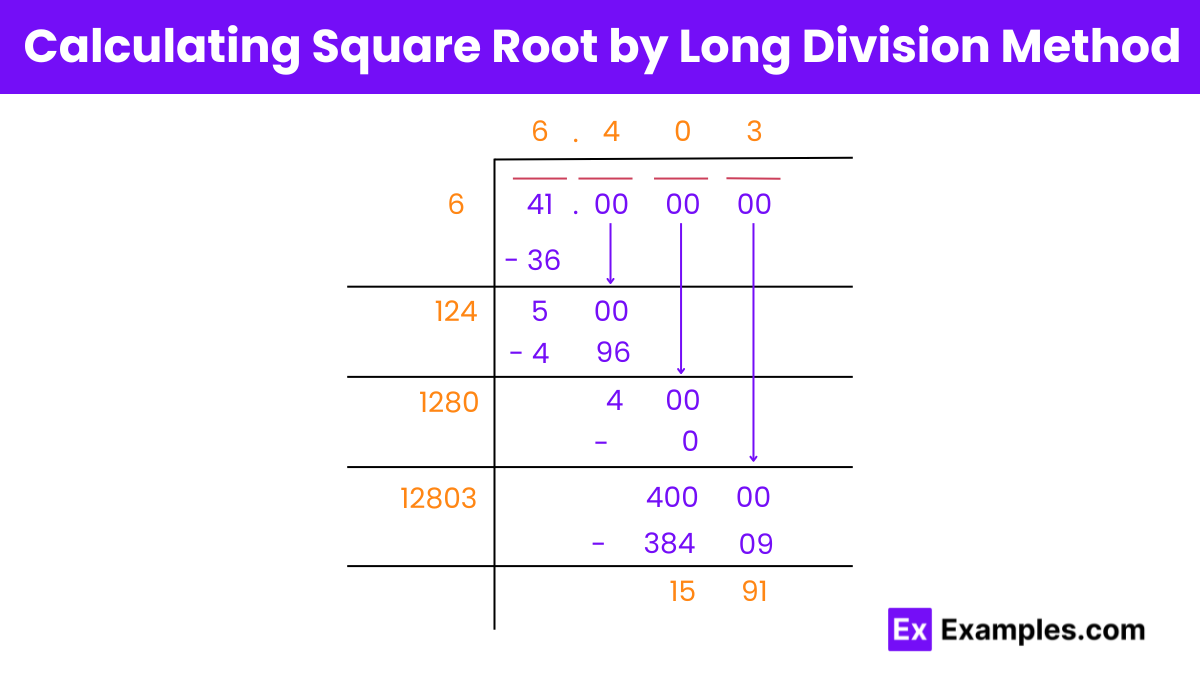
Step 1: Group the digits into pairs, with a bar over them, representing the number 41.
Step 2: Start by finding the largest number whose square is less than or equal to 41. Since 6×6=36, we try 6 as our initial quotient.
Step 3: Place a decimal point and pairs of zeros to continue the division. Multiply the quotient (6) by 2 to get 12, the starting digits of our next divisor.
Step 4: Choose a number in the unit’s place for the new divisor such that its product with a number is less than or equal to 500. We find that 124×4=496 is the closest multiplication.
Step 5: Bring down the next pair of zeros and multiply the current quotient (64) by 2, resulting in 128, forming the starting digits of the new divisor.
Step 6: Choose the largest digit in the unit’s place for the new divisor such that its product with the digit at one’s place is less than or equal to 400. We find that 1281, when multiplied by 1, gives 1281, which is greater than 400. Therefore, we take 1280×0=0, which is less than 400.
Step 7: Repeat the process by adding more pairs of zeros and finding the new divisor and product until the desired level of accuracy is achieved.
This method breaks down the process of finding the square root of 41 into manageable steps, making it easier to understand and follow.
41 Is Not a Perfect Square Root
A perfect square is a number that can be expressed as the square of an integer. In other words, it is the product of an integer multiplied by itself. For example, 25 is a perfect square because it equals 5×5.
However, 41 cannot be expressed as the product of an integer multiplied by itself. Therefore, it is not a perfect square.
The square root of 41 (√41) is an irrational number, approximately equal to 6.40312423743. It cannot be expressed as a fraction and its decimal representation is non-terminating and non-repeating.
Various methods can be used, such as estimation, long division, or iterative algorithms like Newton’s method. These methods involve iteratively refining an initial guess to approximate the value of √41.
No, the decimal representation of the square root of 41 does not follow a repeating or terminating pattern. The digits continue infinitely without a discernible repetition.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What is the square of 14?
196
198
200
202
Which of the following is closest to the square root of 14?
3.7
3.8
3.9
4
If x² = 14, what is x?
3.4
3.6
3.8
4.0
Simplify √14 to its decimal form.
3.73
3.74
3.75
3.76
What is (14)²?
192
194
196
200
Find the approximate value of √14 to two decimal places.
3.73
3.74
3.75
3.76
Which number is closest to 14 but not a perfect square?
12
15
16
18
What is 14 raised to the power of 0.5?
3.7
3.8
4.0
4.1
Calculate 14 × 14.
192
194
196
198
What is the square root of 14 rounded to the nearest integer?
2
4
6
8
Before you leave, take our quick quiz to enhance your learning!

