Square Root 1 to 10
Square Root 1 to 10
Exploring square roots from 1 to 10 reveals the fundamental relationship between numbers and their roots, encompassing rational and irrational numbers. Understanding square and square roots is essential in algebra and number theory, providing insights into quadratic equations and the least square method in statistics. These roots represent the inverse operation of squaring, revealing the original integer from its square. Mastery of square roots enhances numerical literacy, facilitating deeper comprehension of mathematical principles and applications.
The square root of numbers from 1 to 10 refers to the value that, when multiplied by itself, yields the original number. It encompasses both rational and irrational numbers and is a fundamental concept in algebra, number theory, and mathematical analysis. Understanding square roots is essential for solving equations, evaluating expressions, and understanding the relationship between squares and their roots.
Square Roots 1 to 10
In exponential form: (x)¹/²
Where x is any number between 1 to 10.
Square Root from 1 to 10 Chart
Download Square Root 1 to 10 in PDF
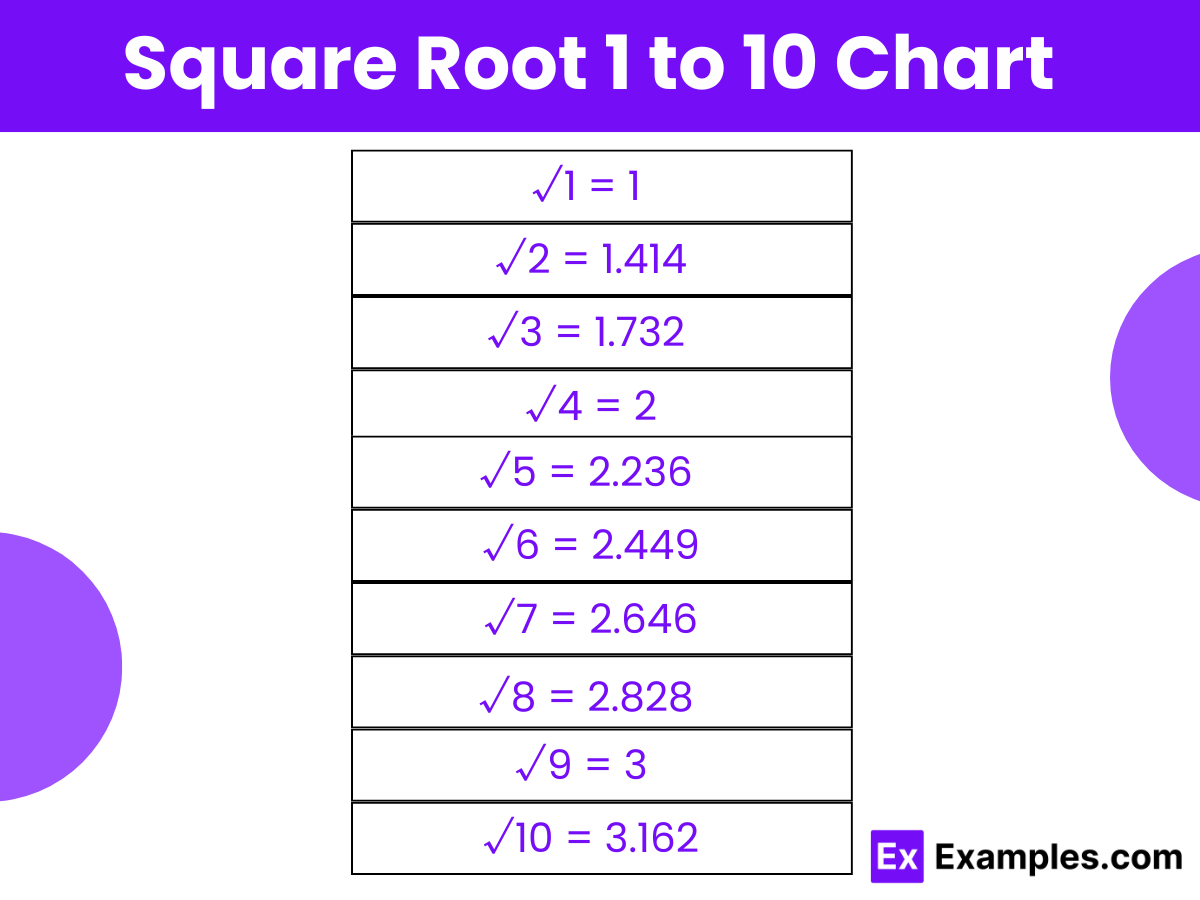
| Square Root from 1 to 10 |
| √1 = 1 |
| √2 = 1.414 |
| √3 = 1.732 |
| √4 = 2 |
| √5 = 2.236 |
| √6 = 2.449 |
| √7 = 2.646 |
| √8 = 2.828 |
| √9 = 3 |
| √10 = 3.162 |
The given content provides the square roots of numbers from 1 to 10, both exact values and approximate values. Square roots represent the number which, when multiplied by itself, yields the original number. For example, the square root of 9 is 3 because 3 multiplied by itself equals 9. The approximate values are rounded to three decimal places for convenience. Understanding square roots is crucial in various mathematical applications, including geometry, algebra, and calculus.
Square Root 1 to 10 for Perfect Squares
| √1 = 1 |
| √4 = 2 |
| √9 = 3 |
This content lists the square roots of numbers from 1 to 10, focusing on perfect square values where the square root is an integer. Understanding these square roots helps grasp fundamental mathematical relationships and aids in solving equations and evaluating expressions.
More About Square Root 1 to 10
| Square Root of 1 | Square Rootof 2 | Square Rootof 3 | Square Rootof 4 | Square Root of 5 |
| Square Rootof 6 | Square Rootof 7 | Square Rootof 8 | Square Rootof 9 | Square Rootof 10 |
Square Root 1 to 10 for Non-Perfect Squares
| √2 = 1.414 |
| √3 = 1.732 |
| √5 = 2.236 |
| √6 = 2.449 |
| √7 = 2.646 |
| √8 = 2.828 |
| √10 = 3.162 |
This content provides the square roots of numbers from 1 to 10, including non-perfect squares, offering both exact and approximate values. Understanding these square roots is essential for numerical calculations and mathematical applications where precise values are required.
How to Calculate Square Root from 1 to 10?
To calculate the square roots of numbers from 1 to 10:
- Use a Calculator: The easiest way to find square roots is to use a calculator or a mathematical software program. Simply input the number and press the square root button.
- Estimate with Approximations: For mental calculations, you can approximate square roots using known perfect squares. For example, to estimate √7, you might know that √4 = 2 and √9 = 3, so √7 is between 2 and 3, closer to 3.
- Use a Square Root Table: You can also refer to a square root table, which provides approximate values for square roots up to a certain precision. Locate the number you’re interested in and read off its square root.
- Long Division Method: For those who want to calculate square roots manually, the long division method can be used. This involves a step-by-step process of finding the square root through successive approximation and division.
- Newton’s Method: Another method for finding square roots manually is Newton’s method, which is an iterative algorithm for approximating roots. While more complex, it can provide accurate results with successive iterations.
FAQs
What is the long division method for calculating square roots?
The long division method involves repeatedly dividing the number whose square root is being calculated by an approximation of its square root, adjusting the approximation until the desired level of accuracy is achieved.
Why is it useful to know how to calculate square roots manually?
While calculators are convenient, manual calculation methods can improve mental math skills and provide a deeper understanding of mathematical concepts. Additionally, in certain situations where calculators are unavailable, manual methods may be necessary.
What are some practical applications of knowing how to calculate square roots?
Knowing how to calculate square roots is valuable in various fields, including engineering, physics, and finance. It is used in tasks such as estimating distances, calculating areas, and solving quadratic equations.
Share :
