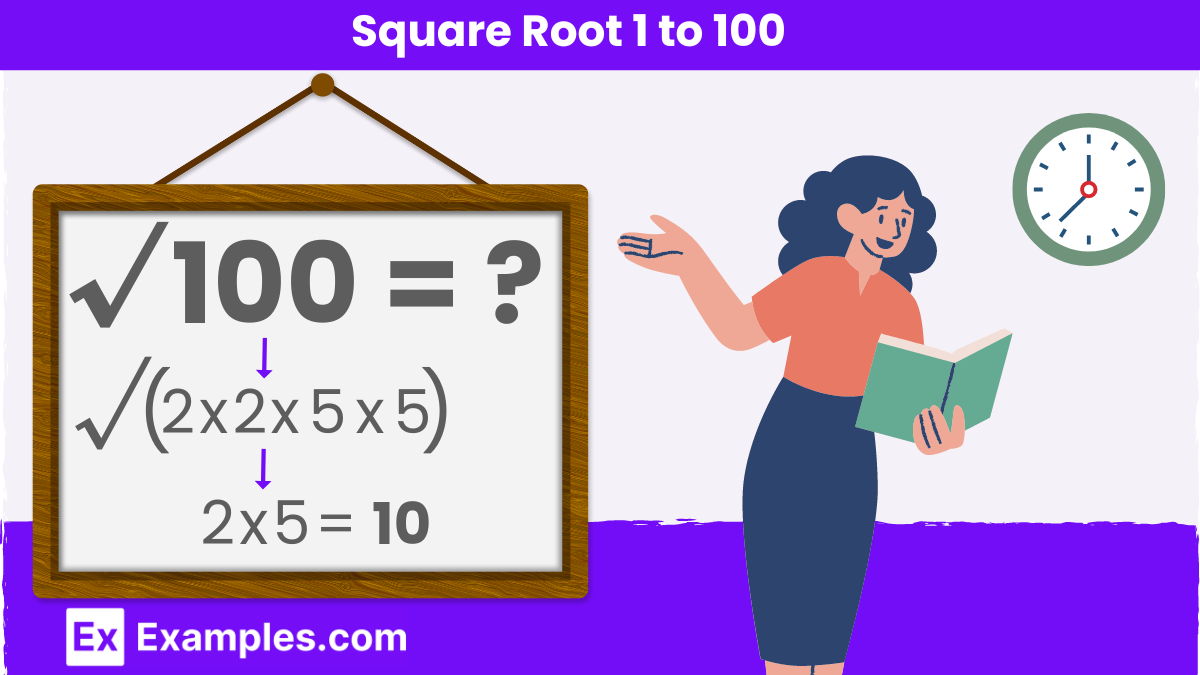
Square Root 1 to 100

Team Maths - Examples.com
Created by: Team Maths - Examples.com, Last Updated: June 10, 2024

Square Root 1 to 100

Exploring squares from 1 to 40 delves into the realm of rational and irrational numbers, a fundamental concept in mathematics. Through algebraic principles, this range highlights both perfect squares, where the Square and square root yields rational results, and non-perfect squares, producing irrational outcomes. Understanding squares within this integer range extends beyond basic arithmetic, offering insights into geometry, statistics, and the least square method, crucial for data analysis and modeling in various fields

Download Square Root 1 to 100 in PDF

Squares from 1 to 40 represent the set of numbers obtained by multiplying each integer from 1 to 40 by itself. These squares include both perfect squares, where the result is an integer, and non-perfect squares, resulting in irrational numbers. Understanding squares within this range is fundamental in mathematics, providing insights into algebraic patterns, geometry, and statistical analysis.

Square Root 1 to 100

In radical form: √x

In exponential form: (x)¹/²

Largest Square Root: √100 = 10.

Where x is any number between 1 to 20

Square Root 1 to 100 Chart

Square-Root-1-to-100-Chart

Download Square Root 1 to 100 in PDF

Square RootValue
√11
√21.414
√31.732
√42
√52.236
√62.449
√72.646
√82.828
√93
√103.162
√113.317
√123.464
√133.606
√143.742
√153.873
√164
√174.123
√184.243
√194.359
√204.472
√214.583
√224.69
√234.796
√244.899
√255
√265.099
√275.196
√285.292
√295.385
√305.477
√315.568
√325.657
√335.745
√345.831
√355.916
√366
√376.083
√386.164
√396.245
√406.325
√416.403
√426.481
√436.557
√446.633
√456.708
√466.782
√476.855
√486.928
√497
√507.071
√517.141
√527.211
√537.28
√547.348
√557.416
√567.483
√577.55
√587.616
√597.681
√607.746
√617.81
√627.874
√637.937
√648
√658.062
√668.124
√678.185
√688.246
√698.307
√708.367
√718.426
√728.485
√738.544
√748.602
√758.66
√768.718
√778.775
√788.832
√798.888
√808.944
√819
√829.055
√839.11
√849.165
√859.22
√869.274
√879.327
√889.38
√899.434
√909.487
√919.539
√929.592
√939.644
√949.695
√959.747
√969.798
√979.848
√989.899
√999.95
√10010

This table displays the square roots of numbers from 1 to 100 in a systematic format. Each entry presents the square root value alongside its corresponding number.

More About Square Root 1 to 100

Square Root of 1Square Root of 2Square Root of 3Square Root of 4Square Root of 5
Square Root of 6Square Root of 7Square Root of 8Square Root of 9Square Root of 10
Square Root of 11Square Root of 12Square Root of 13Square Root of 14Square Root of 15
Square Root of 16Square Root of 17Square Root of 18Square Root of 19Square Root of 20
Square Root of 21Square Root of 22Square Root of 23Square Root of 24Square Root of 25
Square Root of 26Square Root of 27Square Root of 28Square Root of 29Square Root of 30
Square Root of 31Square Root of 32Square Root of 33Square Root of 34Square Root of 35
Square Root of 36Square Root of 37Square Root of 38Square Root of 39Square Root of 40
Square Root of 41Square Root of 42Square Root of 43Square Root of 44Square Root of 45
Square Root of 46Square Root of 47Square Root of 48Square Root of 49Square Root of 50
Square Root of 51Square Root of 52Square Root of 53Square Root of 54Square Root of 55
Square Root of 56Square Root of 57Square Root of 58Square Root of 59Square Root of 60
Square Root of 61Square Root of 62Square Root of 63Square Root of 64Square Root of 65
Square Root of 66Square Root of 67Square Root of 68Square Root of 69Square Root of 70
Square Root of 71Square Root of 72Square Root of 73Square Root of 74Square Root of 75
Square Root of 76Square Root of 77Square Root of 78Square Root of 79Square Root of 80
Square Root of 81Square Root of 82Square Root of 83Square Root of 84Square Root of 85
Square Root of 86Square Root of 87Square Root of 88Square Root of 89Square Root of 90
Square Root of 91Square Root of 92Square Root of 93Square Root of 94Square Root of 95
Square Root of 96Square Root of 97Square Root of 98Square Root of 99Square Root of 100

Square Root 1 to 100 for Perfect Squares

NumberSquare Root
√11
√42
√93
√164
√255
√366
√497
√648
√819
√10010

This table lists the perfect square numbers from 1 to 100 alongside their respective square roots. Perfect squares are numbers that result from multiplying an integer by itself, thus their square roots are integers.

Square Root 1 to 100 for Non-Perfect Squares

NumberSquare Root
√21.414
√31.732
√52.236
√62.449
√72.646
√82.828
√103.162
√113.317
√123.464
√133.606
√143.742
√153.873
√174.123
√184.243
√194.359
√204.472
√214.583
√224.69
√234.796
√244.899
√265.099
√275.196
√285.292
√295.385
√305.477
√315.568
√325.657
√335.745
√345.831
√355.916
√376.083
√386.164
√396.245
√406.325
√416.403
√426.481
√436.557
√446.633
√456.708
√466.782
√476.855
√486.928
√507.071
√517.141
√527.211
√537.28
√547.348
√557.416
√567.483
√577.549
√587.616
√597.681
√607.746
√617.81
√627.874
√637.937
√658.062
√668.124
√678.185
√688.246
√698.307
√708.367
√718.426
√728.485
√738.544
√748.602
√758.66
√778.774
√788.832
√798.888
√808.944
√829.055
√839.11
√849.165
√859.22
√869.274
√879.327
√889.38
√899.434
√909.487
√919.539
√929.591
√939.643
√949.695
√959.746
√979.849
√989.899
√999.95

This table provides the square roots of non-perfect squares from 1 to 100. Each entry shows a number and its corresponding square root value.

How to Find Square Root from 1 to 100?

To find the square root of numbers from 1 to 100, you can use various methods such as:

  • Prime Factorization Method: Express the number as a product of its prime factors and then group the factors into pairs. The square root will be the product of the square roots of each pair.
  • Estimation Method: Find the nearest perfect squares to the given number and then estimate the square root based on those perfect squares.
  • Long Division Method: Use the long division method to find the square root by iterative approximation.
  • Calculator: Utilize a calculator with a square root function to directly compute the square roots of the numbers from 1 to 100.

FAQs

Can I estimate the square root of a number without knowing its exact value?

Yes, you can use estimation techniques to approximate the square root, especially useful for non-perfect square numbers.

Is there a pattern in the square roots of consecutive numbers?

Yes, generally, as the numbers increase, their square roots also increase, but not necessarily in a linear fashion.

Why are square roots important in mathematics?

Square roots are fundamental in various mathematical concepts and real-world applications, including geometry, physics, and engineering.

AI Generator

Text prompt

Add Tone

Select a Tone

10 Examples of Public speaking

20 Examples of Gas lighting