Square Root 1 to 20
Square Root 1 to 20
Square roots from 1 to 20 encompass a fundamental aspect of mathematics, bridging algebra, numbers, and geometry. They represent the inverse operation of squaring, finding the number which, when multiplied by itself, equals a given number. In this range, we encounter both rational and irrational numbers, where rational roots yield integer results and irrational roots produce non-integer values. Understanding square and square roots is crucial in various mathematical domains, including statistics, Least square where they play a role in calculating measures of central tendency and dispersion.
Download Square Root 1 to 20 in PDF
Square Root 1 to 20 refers to the list of square roots for numbers ranging from 1 to 20. Square roots can be positive or negative, but in this range, only the positive values are considered, ranging from 1 to approximately 4.47214.
In this sequence, the numbers 1, 4, 9, and 16 are perfect squares, resulting in rational square roots, while the rest are non-perfect squares, yielding irrational square roots. The square root of each number from 1 to 20 can be represented in radical form as √x or in exponential form as (x)½.
Square Root 1 to 20
In exponential form: (x)½
Largest Square Root: √20 = 4.4721
Square Root from 1 to 20 Chart
Download Square Root 1 to 20 in PDF
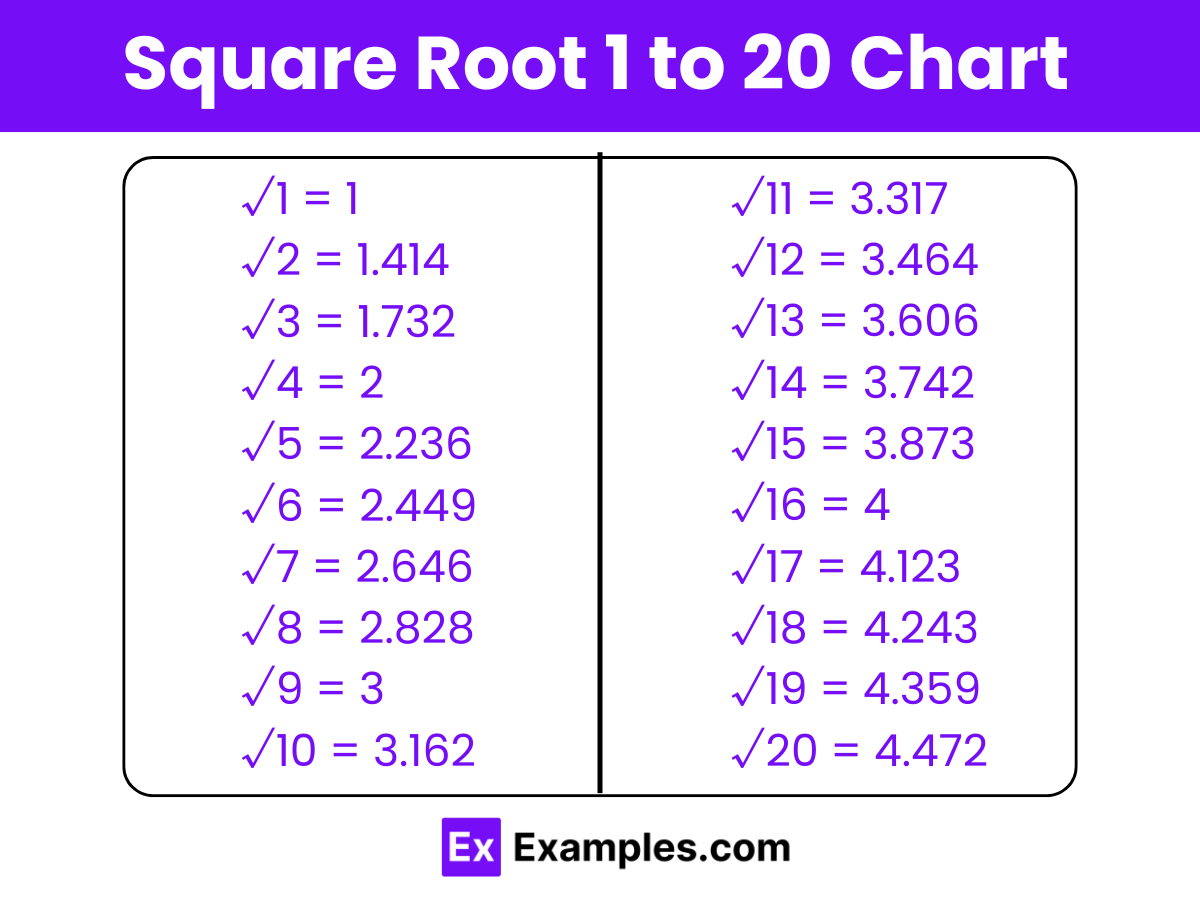
| Square Root from 1 to 20 | |
| √1 = 1 | √11 = 3.317 |
| √2 = 1.414 | √12 = 3.464 |
| √3 = 1.732 | √13 = 3.606 |
| √4 = 2√ | √14 = 3.742 |
| √5 = 2.236 | √15 = 3.873 |
| √6 = 2.449 | √16 = 4 |
| √7 = 2.646 | √17 = 4.123 |
| √8 = 2.828 | √18 = 4.243 |
| √9 = 3 | √19 = 4.359 |
| √10 = 3.162 | √20 = 4.472 |
The square root from 1 to 20 chart displays the square roots of numbers ranging from 1 to 20. It highlights both the rational and irrational nature of square roots within this range.
More About Square Root 1 to 20
Square Root 1 to 20 for Perfect Squares
| √1 = 1 |
| √4 = 2 |
| √9 = 3 |
| √16 = 4 |
In the square root of 1 to 20 for perfect squares, the square roots of numbers 1, 4, 9, and 16 are rational, resulting in whole numbers. This subset highlights the clear and easily identifiable square roots within the range.
Square Root 1 to 20 for Non-Perfect Squares
| √2 = 1.414 |
| √3 = 1.732 |
| √5 = 2.236 |
| √6 = 2.449 |
| √7 = 2.646 |
| √8 = 2.828 |
| √10 = 3.162 |
| √11 = 3.317 |
| √12 = 3.464 |
| √13 = 3.606 |
| √14 = 3.742 |
| √15 = 3.873 |
| √17 = 4.123 |
| √18 = 4.243 |
| √19 = 4.359 |
| √20 = 4.472 |
For non-perfect squares in the range from 1 to 20, their square roots result in irrational numbers, which cannot be expressed as fractions and have non-repeating decimal expansions. These square roots are important in mathematics and various real-world applications, representing values such as lengths, areas, and volumes.
How to Calculate Square Root from 1 to 20?
To calculate the square root of numbers from 1 to 20, you can use various methods such as:
- Calculator: Use a scientific calculator with a square root function to find the square root of each number individually.
- Long Division Method: Apply the long division method to find the square root of each number manually. This involves a step-by-step process of approximation and division.
- Prime Factorization: Decompose each number into its prime factors and then simplify to find the square root.
- Estimation: Make an educated guess of the square root based on the nearest perfect squares and refine the estimate through repeated approximations.
FAQs
How do you estimate square roots?’
Estimating square roots involves finding the nearest perfect squares above and below the given number, then making an educated guess between them. For example, to estimate √15, we find that 3² = 9 and 4² = 16, so √15 is between 3 and 4.
What are the practical applications of square roots?
Square roots are used in various fields such as mathematics, engineering, finance, and physics. They are essential for calculating distances, areas, volumes, and in algorithms for data analysis.
Why are some square roots irrational?
Square roots of numbers that are not perfect squares result in irrational numbers, meaning they cannot be expressed as fractions. For example, √2 is irrational because it cannot be written as a simple fraction.
Share :
