What is the square root of 16?
3
4
5
6
of 10
Square Root from 1 to 30 – Value of Square Root from 1 to 100 [PDF]
Square Root from 1 to 30 – Value of Square Root from 1 to 100 [PDF]
Square roots, a fundamental concept in mathematics, represent the inverse operation of squaring a number. From 1 to 30, square and square roots encompass both rational and irrational numbers, with perfect squares yielding rational roots while non-perfect squares result in irrational roots. In algebraic terms, the square root of a number ‘x’ is denoted as √x. Understanding square roots is crucial in various mathematical disciplines, including algebra, where they play a pivotal role in solving equations and finding roots. Moreover, square roots are integral to the study of integers, offering insights into the properties and relationships between numbers. Employing techniques like the least square method, mathematicians and scientists utilize square roots extensively in statistical analysis and data modeling, contributing to advancements in diverse fields.
Download Square Root 1 to 30 in PDF
The square root of a number ‘x’ is a value that, when multiplied by itself, equals ‘x’. It is denoted by the symbol √x. For example, the square root of 9 is 3 because 3 × 3 = 9. In mathematical terms, if y = √x, then y² = x. Square roots can be both rational and irrational, depending on whether the number being rooted is a perfect square or not.
Square Roots 1 to 30
In exponential form: (x)¹/²
Where x is any number between 1 to 30.
Square Root from 1 to 30 Chart
Download Square Root 1 to 30 in PDF
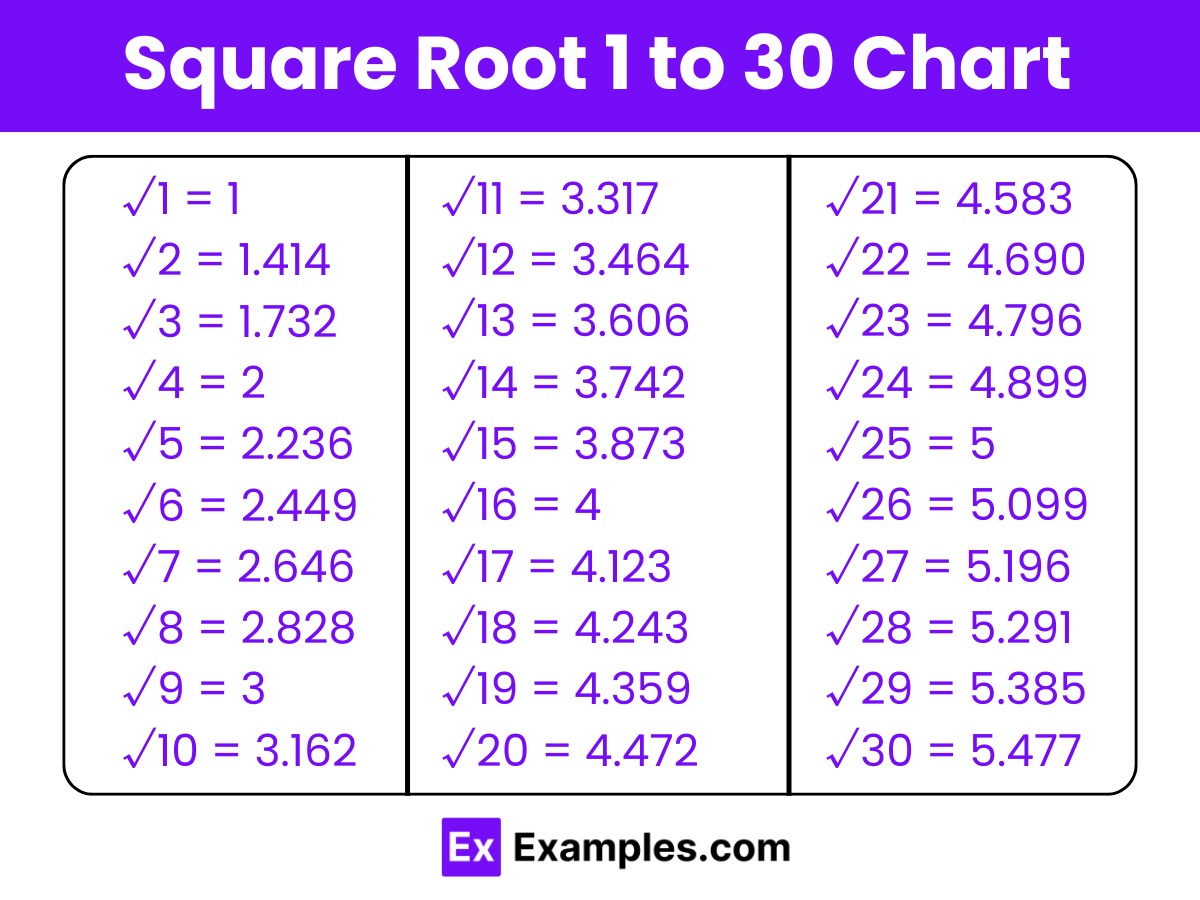
| Square Root from 1 to 30 | |
| √1 = 1 | √16 = 4 |
| √2 = 1.414 | √17 = 4.123 |
| √3 = 1.732 | √18 = 4.243 |
| √4 = 2 | √19 = 4.359 |
| √5 = 2.236 | √20 = 4.472 |
| √6 = 2.449 | √21 = 4.583 |
| √7 = 2.646 | √22 = 4.690 |
| √8 = 2.828 | √23 = 4.796 |
| √9 = 3 | √24 = 4.899 |
| √10 = 3.162 | √25 = 5 |
| √11 = 3.317 | √26 = 5.099 |
| √12 = 3.464 | √27 = 5.196 |
| √13 = 3.606 | √28 = 5.292 |
| √14 = 3.742 | √29 = 5.385 |
| √15 = 3.873 | √30 = 5.477 |
Square roots from 1 to 30 include rational roots for perfect squares and irrational roots for non-perfect squares. Understanding these roots is essential in mathematics, particularly in algebraic equations and geometric calculations.
More About Square Root 1 to 10
Square Root 1 to 30 for Perfect Squares
| √1 = 1 |
| √4 = 2 |
| √9 = 3 |
| √16 = 4 |
| √25 = 5 |
Square roots for perfect squares from 1 to 30 result in whole numbers, as they are the square roots of integers. Perfect squares have rational roots, making their square roots terminate or repeat in decimal form.
Square Root 1 to 30 for Non-Perfect Squares
| √2 = 1.414 | √18 = 4.243 |
| √3 = 1.732 | √19 = 4.359 |
| √5 = 2.236 | √20 = 4.47 |
| √6 = 2.449 | √21 = 4.583 |
| √7 = 2.646 | √22 = 4.690 |
| √8 = 2.828 | √23 = 4.796 |
| √10 = 3.162 | √24 = 4.899 |
| √11 = 3.317 | √26 = 5.099 |
| √12 = 3.464 | √27 = 5.196 |
| √13 = 3.606 | √28 = 5.292 |
| √14 = 3.742 | √29 = 5.385 |
| √15 = 3.873 | √30 = 5.477 |
| √17 = 4.123 |
Square roots of non-perfect squares from 1 to 30 result in irrational numbers, such as √2, √3, and √5, which cannot be expressed as simple fractions. These roots are crucial in mathematics for their role in geometry, calculus, and other advanced fields.
How to Calculate Square Root from 1 to 30?
To calculate square roots from 1 to 30, you can use various methods such as:
- Prime Factorization Method: Express each number as a product of its prime factors and group them into pairs to find the square root.
- Estimation Method: Approximate the square root by identifying the nearest perfect squares above and below the given number.
- Long Division Method: Utilize the long division method to iteratively approximate the square root to a desired level of accuracy.
- Calculator: Use a scientific calculator with a square root function to directly compute the square roots of numbers from 1 to 30.
FAQs
Are there any patterns or shortcuts for calculating square roots from 1 to 30?
Some numbers may have recurring patterns in their square roots, allowing for faster mental calculations or estimation techniques.
What is the significance of calculating square roots from 1 to 30?
Understanding square roots in this range provides a foundational grasp of mathematical concepts and their applications in various fields.
What are some real-world applications of square roots within the range of 1 to 30?
Square roots in this range are fundamental in geometry, engineering, finance, and various scientific disciplines for measurements, calculations, and problem-solving.
Share :
Practice Test
Which number squared equals 25?
3
4
5
6
of 10
What is the approximate square root of 3?
1.5
1.6
1.7
1.8
of 10
What is the square root of 9?
2
3
4
5
of 10
Which of the following is the square root of 4?
1
2
3
4
of 10
Which number squared equals 36?
5
6
7
8
of 10
What number squared equals 49?
6
7
8
9
of 10
What is the approximate square root of 2?
1.3
1.4
1.5
1.6
of 10
Which number squared equals 16?
2
3
4
5
of 10
What is the square root of 12 (approximately)?
3.2
3.3
3.4
3.5
of 10