What is the square of 1000?
1,000,000
1,000,100
1,000,000,000
1,000,000,100

The square numbers of 1000, or 1000 squared, equals 1,000,000. This result signifies the area of a square with sides measuring 1000 units. It holds significance in math and practical applications for its magnitude and precision.
The square root of 1000 is expressed as √1000. It equals approximately 31.62 because 31.62 multiplied by itself (31.62 × 31.62) equals 1000. Mathematically, finding the square root of a number involves determining the non-negative value x such that f(x) = 1000 in the function f(x) = x².
Square Root of 1000 : 31.622
Exponential Form: 1000^1/2 or 1000^0.5
Radical Form: √1000
Irrational numbers cannot be expressed as fractions of integers. The square root of 1000, approximately 31.62, cannot be simplified to a fraction. It is a non-repeating, non-terminating decimal, making it irrational and not expressible as a simple fraction.
rational numbers are those that can be expressed as the quotient of two integers, where the denominator is not zero.
Examples : 1/2, -3, and 5.
Irrational numbers can’t be written as fractions of integers. Their decimal forms go on forever without repeating. They show up in geometry and algebra, like square roots of non-perfect squares or π.
Examples : √2 ,√3 , √5 , π (pi)
No, because it cannot be expressed as the square of an integer. Its square root is approximately 31.622776, which is not a whole number. Therefore, 1000 is not a perfect square root.
The square root of 1000 can be found using methods like estimation, calculator, or algebraic manipulation.
The square root of 1000 is important in mathematics and various fields for calculations involving area, geometry, and algebraic equations.
The square root of 1000 is not a whole number because 1000 is not a perfect square.
No, the square root of 1000 cannot be simplified further as it is an irrational number.
1000 is a milestone number and is used as a base in various numerical systems and calculations.

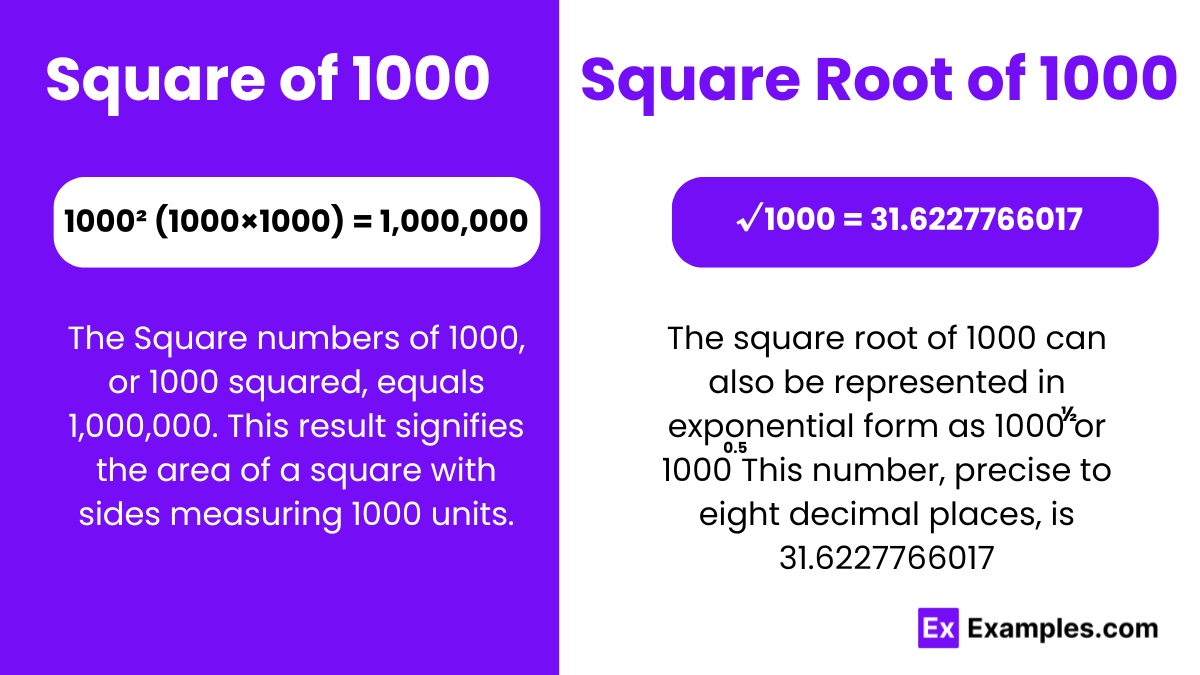
1000² (1000×1000) = 1,000,000
The square numbers of 1000, or 1000 squared, equals 1,000,000. This result signifies the area of a square with sides measuring 1000 units. It holds significance in math and practical applications for its magnitude and precision.
√1000 = 31.6227766017
or
√1000 = 31.622
The square root of 1000 is expressed as √1000. It equals approximately 31.62 because 31.62 multiplied by itself (31.62 × 31.62) equals 1000. Mathematically, finding the square root of a number involves determining the non-negative value x such that f(x) = 1000 in the function f(x) = x².
Square Root of 1000 : 31.622
Exponential Form: 1000^1/2 or 1000^0.5
Radical Form: √1000
The square root of 1000 is an irrational number
Irrational numbers cannot be expressed as fractions of integers. The square root of 1000, approximately 31.62, cannot be simplified to a fraction. It is a non-repeating, non-terminating decimal, making it irrational and not expressible as a simple fraction.
rational numbers are those that can be expressed as the quotient of two integers, where the denominator is not zero.
Examples : 1/2, -3, and 5.
Irrational numbers can’t be written as fractions of integers. Their decimal forms go on forever without repeating. They show up in geometry and algebra, like square roots of non-perfect squares or π.
Examples : √2 ,√3 , √5 , π (pi)
Estimation: Using approximation techniques like the Babylonian method.
Calculator: Utilizing a calculator or a computer software to calculate the square root directly.
Table lookup: Referring to square root tables or online resources for the value.
Algebraic manipulation: Employing algebraic techniques to simplify or approximate the value.
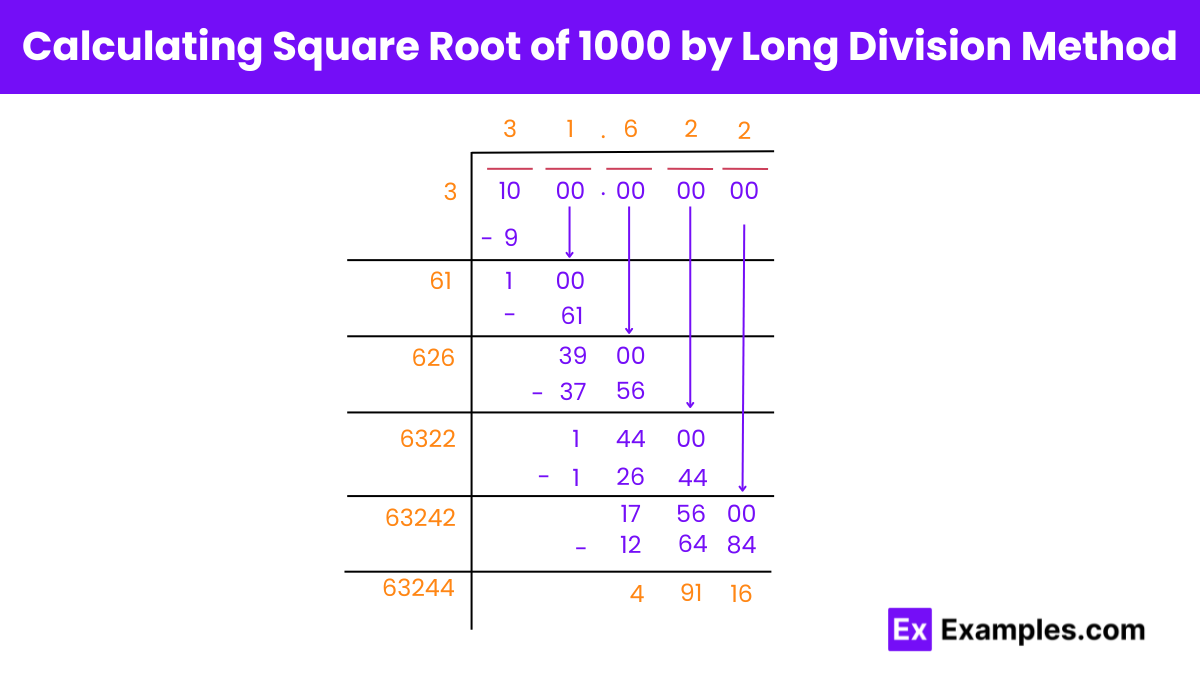
Start with Decimal Form: Write 1000 as 1000.000000.
Pair the Number: Pair digits from right to left with a bar on top.
Find Initial Quotient and Remainder: Divide 10 by a number, yielding a product less than or equal to 10. The quotient is 3 with a remainder of 1.
Double the Quotient: Obtain 6 and assume 60 as the new divisor. Bring down 00 for division.
Find Next Quotient and Remainder: Find a number such that (60 + number) × number ≤ 100. It’s 61, resulting in a quotient of 31 and a remainder of 39.
Repeat the Process: Continue this process, doubling the quotient each time and bringing down the appropriate digits, until reaching the desired level of precision.
Obtain Approximation: The approximate square root of 1000 is 31.622.
No, 1000 is not a perfect square
No, because it cannot be expressed as the square of an integer. Its square root is approximately 31.622776, which is not a whole number. Therefore, 1000 is not a perfect square root.
The square root of 1000 can be found using methods like estimation, calculator, or algebraic manipulation.
The square root of 1000 is important in mathematics and various fields for calculations involving area, geometry, and algebraic equations.
The square root of 1000 is not a whole number because 1000 is not a perfect square.
No, the square root of 1000 cannot be simplified further as it is an irrational number.
1000 is a milestone number and is used as a base in various numerical systems and calculations.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What is the square of 1000?
1,000,000
1,000,100
1,000,000,000
1,000,000,100
What is the approximate square root of 1000 to two decimal places?
31.50
31.60
31.62
31.70
Which of the following numbers is closest to the square root of 1000?
31
32
33
34
If x² = 1000, what is the value of x?
30
31
31.62
32
What is the square of 1000 plus 1000?
1,001,000
1,002,000
1,000,000
2,000,000
What is the square root of 1000 multiplied by 2?
62.44
63.24
64.24
65.24
Which of the following is the closest to the square of 31.62?
900
950
1000
1050
If the side length of a square is 1000 units, what is the area of the square?
1,000,000 square units
2,000,000 square units
1,000,100 square units
1,000,010 square units
If the side length of a square is the square root of 1000, what is the perimeter of the square?
126.48 units
127.48 units
128.48 units
129.48 units
Which number squared is equal to 1000?
31.60
31.61
31.62
31.63
Before you leave, take our quick quiz to enhance your learning!

