What is the square of 119?
12,000
14,161
13,321
15,361

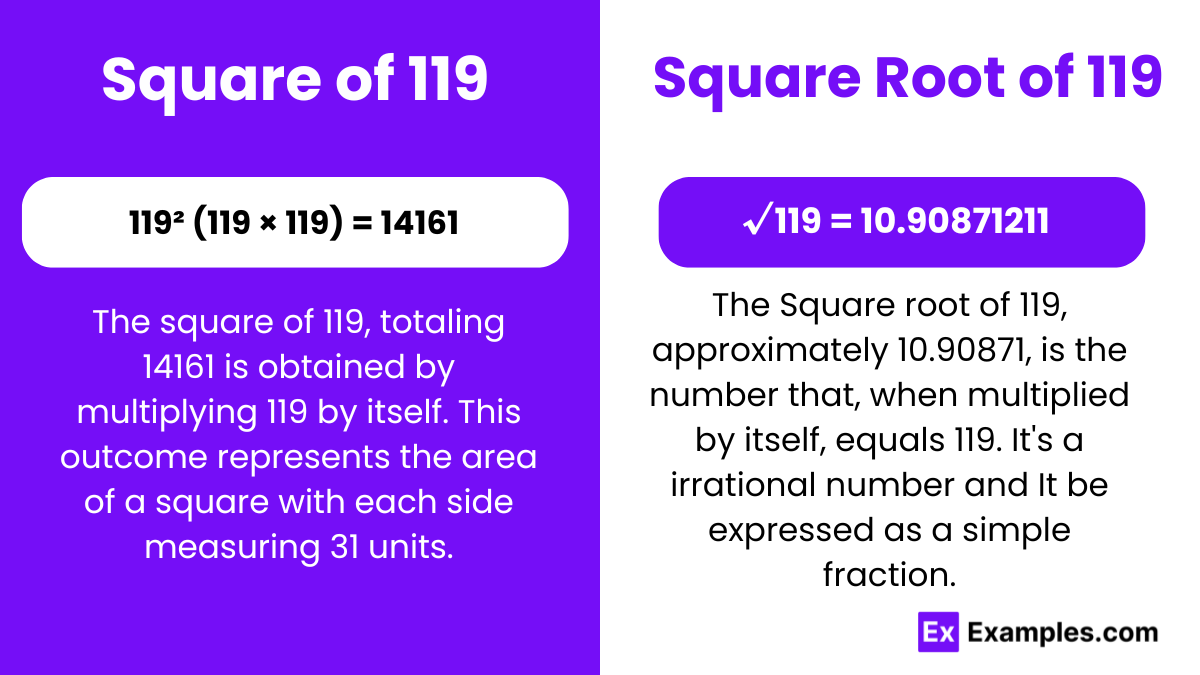
In mathematics, especially within the domain of algebra, grasping the concepts of squares and square roots is essential. Squaring the number 119, by multiplying it by itself, results in 14161. This computation sheds light on the characteristics of whole numbers and serves as a foundation for probing into more intricate mathematical relationships and patterns. Engaging in such calculations is fundamental for deepening our understanding of advanced mathematical concepts and theories.
The square of 119 refers to the result of multiplying the number 119 by itself. Mathematically, this is expressed as 119×119. Squaring a number is one of the fundamental operations in arithmetic and algebra, serving as a key concept for developing more complex mathematical ideas. When you square 119, you obtain 14161. This calculation helps illustrate properties of exponents and powers, which are crucial for understanding advanced topics in mathematics such as quadratic equations, power functions, and polynomial identities.
√119 = 10.90871211
Or
√119 = 10.909 (up to 3 decimals)
The square root of 119 refers to the number that, when multiplied by itself, results in 119. Mathematically, this is expressed as √119. The exact square root of 119 is an irrational number, meaning it cannot be expressed as a simple fraction, and its decimal representation continues indefinitely without repeating. This concept is central in various branches of mathematics, including algebra and geometry, where understanding square roots is crucial for solving equations and calculating dimensions. The square root of 119, approximately 10.90871211, is particularly useful in real-world applications where precise calculations are necessary, such as in engineering and physics.
Square Root of 119: 10.90871211
Exponential Form of 119: 119^½ or 119^0.5
Radical Form of 119: √119
Finding the value of the square root of 119 involves a few methods. Here are a couple of common ones:
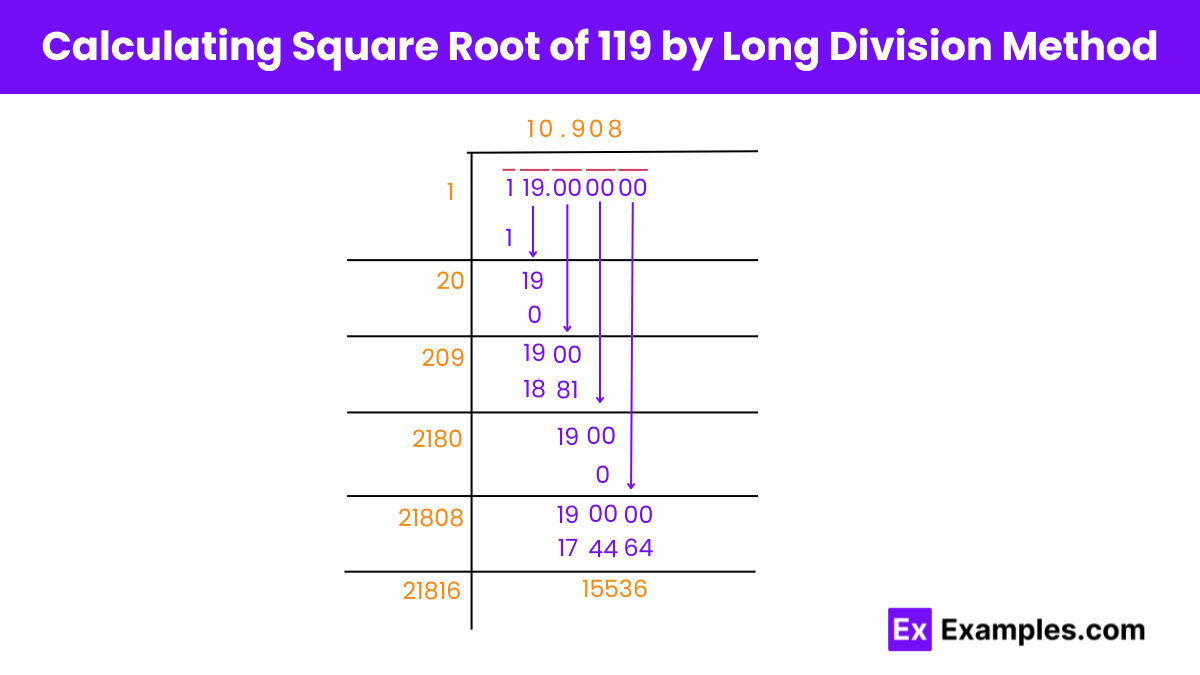
Step 1: Initial Setup
Step 2: Find Next Digit
Step 3: Continue Division
A perfect square is a number that can be expressed as the product of an integer with itself. For example, 121 is a perfect square because it can be expressed as 11×11. However, 119 cannot be expressed as the product of an integer with itself, so it is not a perfect square.
The simplified form of the square root of 119 is √119, as it cannot be simplified further into a product of smaller square roots.
No, 119 is not a perfect square. A perfect square is an integer that can be expressed as the square of another integer.
The factors of 119 are 1, 7, 17, and 119. These numbers can divide 119 without leaving a remainder.
The numbers 7 and 17 multiply to get 119, since 7 × 17 = 119.
Yes, 119 is divisible by 1, 7, 17, and 119, as these numbers divide 119 without leaving a remainder.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What is the square of 119?
12,000
14,161
13,321
15,361
What is the square root of 119 rounded to two decimal places?
10.88
10.91
11.03
11.09
If the square of a number is 14,161, what is the number?
118
119
120
121
What is the approximate square of the square root of 119?
119
120
121
225
Which of the following is closest to the square root of 119?
9
10
11
12
What is 119 squared minus 1000?
13,161
14,161
15,161
12,161
If the square of a number is 14,161, what is the number of its square root rounded up?
10
11
12
13
How much more is the square of 120 compared to the square of 119?
2,481
1,441
2,401
2,000
What is the result of squaring the square root of 119 and then subtracting 1?
118
119
120
121
Which number has the same square root as the square of 119?
119
14,161
10,000
11,000
Before you leave, take our quick quiz to enhance your learning!

