Square & Square Root of 130

In the field of mathematics, particularly within algebraic studies, the concepts of squares and square roots are crucial. Squaring a number, as seen when 130 is multiplied by itself to produce 16900, is a core operation. This mathematical function is essential for exploring properties of both rational and irrational numbers. Rational numbers can be expressed as a fraction of two integers, while irrational numbers cannot be neatly expressed as such fractions. Understanding squares and square roots enhances one’s grasp of mathematical relationships and patterns, establishing a foundational knowledge that is integral to further mathematical exploration and learning. This comprehension is vital in appreciating the structure and logic that underpin mathematical theories.

Square of 130

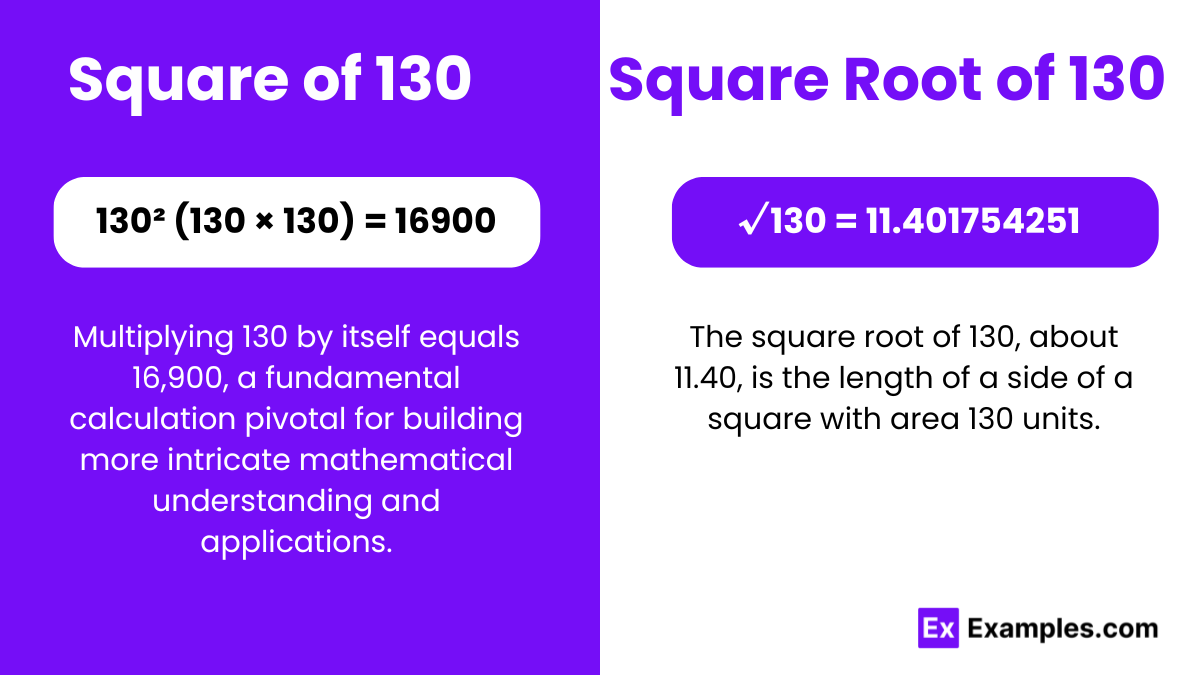
130² (130 × 130) = 16900

The square of 130 is a prime example of a square number, calculated by multiplying 130 by itself, resulting in 16900. This operation illustrates the concept of square numbers, which are products of an integer multiplied by itself.

Square Root of 130

√130 = 11.401754251

Or

√130 = 11.401 Upto 3 decimals

The square root of 130, a key mathematical concept, is the value that, when multiplied by itself, yields 130. This square root, approximately 11.4018, demonstrates the process of finding a number that perfectly squares to produce the original number, 130.

Square Root of 130: 11.401754251

Exponential Form: 130^½ or 130^0.5

Radical Form: √130

Is the Square Root of 130 Rational or Irrational?

The square root of 130 is an irrational number

The square root of 130 is an irrational number. It cannot be expressed as a simple fraction because it is a non-terminating, non-repeating decimal. This property is typical of square roots of non-perfect squares, making them irrational, as they do not result in neat, fractional outcomes.

Rational Numbers:

Rational numbers are those that can be expressed as the ratio of two integers, represented as (a/b), where the denominator (b) is not zero. These numbers include positive, negative, and zero values, such as (3/4), (-5/2), (0), (1), and (-2).

Example: Take (3/4); since both 3 and 4 are integers and the denominator is not zero, (3/4) qualifies as a rational number.

Irrational Numbers:

Irrational numbers, on the other hand, cannot be expressed as a simple fraction of two integers. Their decimal expansions are infinite and non-repetitive, which makes them impossible to represent in the form (a/b).

Example: Consider (√2); it features a decimal expansion that continues indefinitely without repeating ((√2 \approx 1.41421356…)), classifying it as irrational.

Method to Find Value of Root 130

To find the value of the square root of 130, you can use several methods, but here’s a straightforward approach using the long division method, which is particularly effective for square roots of non-perfect squares:

Estimation: Begin by finding the two perfect squares between which 130 lies. The square root of 121 (which is 11) is less than the square root of 130, and the square root of 144 (which is 12) is more. Thus, the square root of 130 is between 11 and 12.

Long Division Method:

Pair the digits of the number starting from the decimal point. For 130, the pair will just be 130 (and 00 if you extend it).

Find the largest number whose square is less than or equal to 130. Here, it’s 11 because (11² = 121).

Subtract 121 from 130, which gives 9, and bring down two zeros, making it 900.

Now, double the divisor (11 becomes 22). Find a digit (X) such that (22X × X) is less than or equal to 900. (X) will be 4, as (224 × 4 = 896).

Subtract 896 from 900 to get 4, and bring down another pair of zeros to continue.

Decimal Precision: You can continue this process, extending the number with more zeros and following the same steps to get more decimal places for a more precise value.

Verification with a calculator: After calculation, you can always check your result using a calculator to ensure accuracy, especially if precision is critical.

This method, while somewhat laborious, is very educational and provides a deep understanding of how square roots are calculated manually. For practical purposes, calculators or software tools can compute the square root of 130 much more quickly and with great precision.

Square Root of 130 by Long Division Method

Step 1: Grouping the Digits

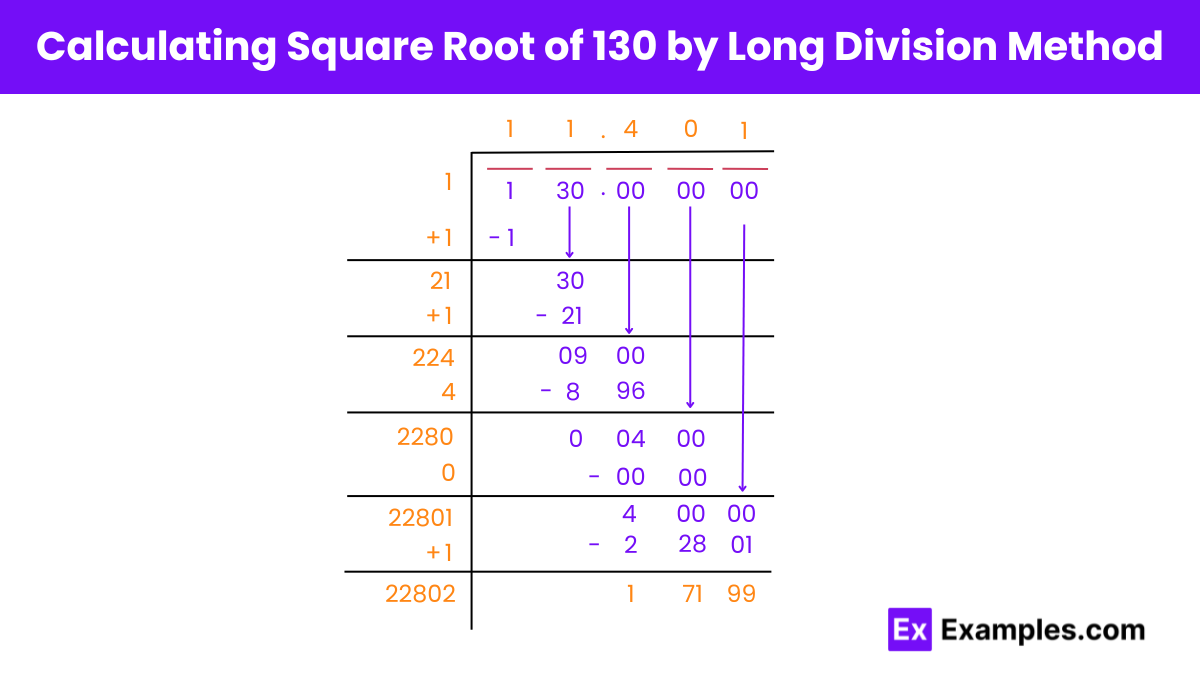
Begin by grouping the digits of 130 from the unit’s place in pairs of two, placing a bar above them. For 130, the groups are 30 and 1 (grouped from the right).

Step 2: Finding the Initial Quotient

Identify a number ( t ) whose square is less than or equal to 1. Here, ( t = 1 ) since ( 1 × 1 = 1 ), which satisfies the condition. The quotient now is 1.

Step 3: Updating the Divisor

Add the initial divisor ( t ) to itself to get the new divisor, which is ( 2t ) or 2.

Step 4: Dragging the Next Pair of Digits

Bring down the next pair of digits, making the new dividend 030. Find a number ( N ) such that ( 2N × N <₋ 30 ). Here, ( N = 1 ).

Step 5: Adding Decimals and More Digits

Place a decimal in both the dividend (130) and quotient (11). Extend the dividend by adding three pairs of zeros after the decimal. Repeat the previous steps with these extended digits.

Step 6: Calculating to Higher Precision

By repeating the process with the extended digits, you refine the quotient to more decimal places. Continue this until the desired accuracy is achieved.

Conclusion: Final Result

After following these steps, the calculated square root of 130 by the long division method is approximately 11.401.

130 is Perfect Square root or Not?

No, 130 is not a perfect square number

130 is not a perfect square, as its square root is approximately 11.4018, which is not an integer. Perfect squares have integer square roots.

FAQS