What is the square of 23?
529
512
543
532

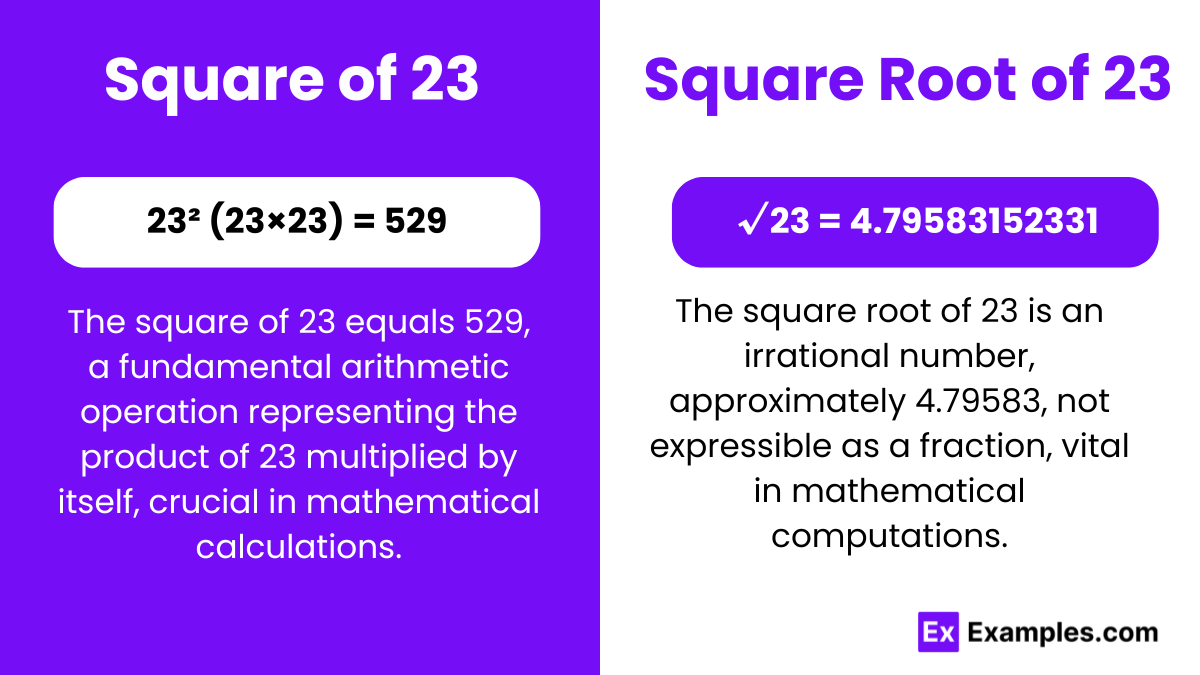
In the vast realm of mathematics, particularly within the domain of algebraic studies, the foundational concepts revolving around square and square roots are of utmost significance. The process of squaring a number, as demonstrated by the example of 23 being multiplied by itself to yield the result 529, stands as a fundamental operation. This operation serves as a cornerstone in exploring the inherent properties of both rational numbers, which are expressible as fractions of two integers, and irrational numbers, which defy neat fractional representation. A grasp of these fundamental concepts enriches one’s comprehension of mathematical relationships and patterns, fostering a deeper understanding of algebraic principles.
23² (23 × 23) = 529
The square of 23, a prime number, equals 529. This square number represents the product obtained by multiplying 23 by itself. Square numbers play a pivotal role in mathematics, showcasing fundamental properties and serving as building blocks for understanding algebraic concepts and mathematical patterns.
Or
√23 = 4.795 Upto 3 decimals
The square root of 23, an irrational number approximately equal to 4.79583, represents the value that, when multiplied by itself, yields 23. Understanding square roots elucidates fundamental mathematical principles, aiding in solving equations, analyzing geometric shapes, and comprehending the relationship between squares and their roots in algebraic contexts.
Square Root of 23: 4.79583152331
Exponential Form: 23^½ or 23^0.5
Radical Form: √23
The square root of 23 is irrational. This means it cannot be expressed as a simple fraction of two integers and its decimal representation continues infinitely without repeating.
Certainly!
Rational numbers : A rational number can be represented as a fraction of two integers, such as 3/4 or -5/2.
Irrational number : An irrational number cannot be expressed as a fraction of two integers, and its decimal expansion neither terminates nor repeats. For example, the square root of 2 (√2) is approximately 1.41421356…, and it is irrational.
There are several methods to find the value of the square root of 23:
Approximation: Use estimation techniques such as Newton’s method or the Babylonian method to iteratively improve an initial guess of the square root.
Long Division: Employ long division to manually compute the square root of 23 to a desired degree of accuracy.
Calculator: Utilize a scientific calculator or a computer program capable of calculating square roots directly.
Table Lookup: Refer to mathematical tables or digital resources providing pre-calculated values of square roots.
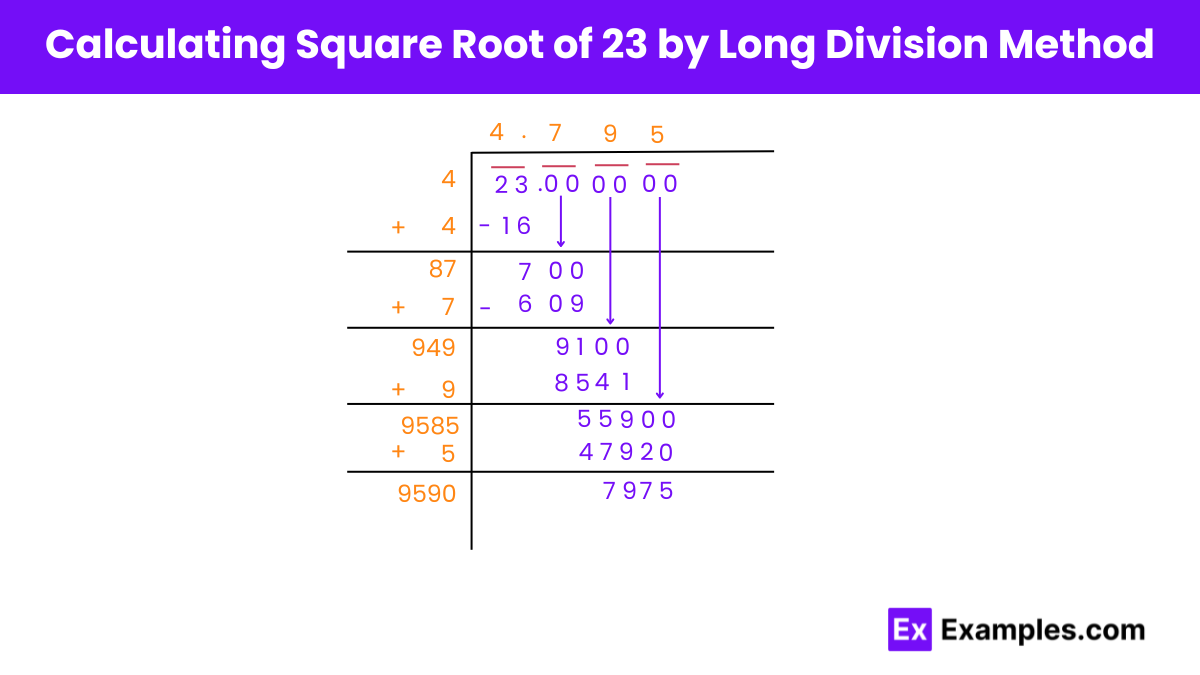
Long Division Method for Finding the Square Root of 23
Step 1: Pairing Digits
Starting from the right, pair up the digits “23” by placing a bar above them. Also, pair the 0s in decimals in pairs of 2 from left to right.
Step 2: Initial Approximation
Find a number that, when multiplied by itself, gives a product less than or equal to 23. In this case, the number 4 fits as (4^2) equals 16. Divide 23 by 4, giving a quotient of 4 and a remainder of 7.
Step 3: Adjusting the Dividend
Drag a pair of 0s down and append them next to the remainder (7) to create the dividend 700.
Step 4: Iterative Calculation
Double the divisor (4) to get 8. Enter 8 below and leave a blank digit to its right. Guess the largest possible digit (X) to fill in the blank, such that the product of 87 and X is less than or equal to 700. In this case, X equals 7, so fill in 7 in the quotient after the decimal point. Divide and record the remainder.
Step 5: Repeat Iteratively
Repeat the process to obtain further decimal places as needed. Continue until the desired level of precision is achieved.
No, 23 is not a perfect square number
No, 23 is not a perfect square because it cannot be expressed as the square of an integer. The square root of 23 is an irrational number, approximately equal to 4.79583, indicating that it cannot be written as the quotient of two integers.
Understanding squares and square roots is crucial in various mathematical applications, including geometry, algebra, and physics, aiding in problem-solving and mathematical reasoning.
No, the square root of 23 is an irrational number and cannot be represented as a fraction of two integers.
The square root of 23 can be used to calculate the length of the diagonal of a square with side length 23 units.
Yes, the square root of 23 may be used in various real-world scenarios, such as calculating distances, areas, or volumes in engineering and construction projects.
The square root of 23 is related to Pythagorean triples, particularly those involving integers that are multiples of 23.

In the vast realm of mathematics, particularly within the domain of algebraic studies, the foundational concepts revolving around square and square roots are of utmost significance. The process of squaring a number, as demonstrated by the example of 23 being multiplied by itself to yield the result 529, stands as a fundamental operation. This operation serves as a cornerstone in exploring the inherent properties of both rational numbers, which are expressible as fractions of two integers, and irrational numbers, which defy neat fractional representation. A grasp of these fundamental concepts enriches one’s comprehension of mathematical relationships and patterns, fostering a deeper understanding of algebraic principles.
23² (23 × 23) = 529
The square of 23, a prime number, equals 529. This square number represents the product obtained by multiplying 23 by itself. Square numbers play a pivotal role in mathematics, showcasing fundamental properties and serving as building blocks for understanding algebraic concepts and mathematical patterns.
√23 = 4.79583152331
Or
√23 = 4.795 Upto 3 decimals
The square root of 23, an irrational number approximately equal to 4.79583, represents the value that, when multiplied by itself, yields 23. Understanding square roots elucidates fundamental mathematical principles, aiding in solving equations, analyzing geometric shapes, and comprehending the relationship between squares and their roots in algebraic contexts.
Square Root of 23: 4.79583152331
Exponential Form: 23^½ or 23^0.5
Radical Form: √23
The square root of 23 is an irrational number
The square root of 23 is irrational. This means it cannot be expressed as a simple fraction of two integers and its decimal representation continues infinitely without repeating.
Certainly!
Rational numbers : A rational number can be represented as a fraction of two integers, such as 3/4 or -5/2.
Irrational number : An irrational number cannot be expressed as a fraction of two integers, and its decimal expansion neither terminates nor repeats. For example, the square root of 2 (√2) is approximately 1.41421356…, and it is irrational.
There are several methods to find the value of the square root of 23:
Approximation: Use estimation techniques such as Newton’s method or the Babylonian method to iteratively improve an initial guess of the square root.
Long Division: Employ long division to manually compute the square root of 23 to a desired degree of accuracy.
Calculator: Utilize a scientific calculator or a computer program capable of calculating square roots directly.
Table Lookup: Refer to mathematical tables or digital resources providing pre-calculated values of square roots.

Long Division Method for Finding the Square Root of 23
Step 1: Pairing Digits
Starting from the right, pair up the digits “23” by placing a bar above them. Also, pair the 0s in decimals in pairs of 2 from left to right.
Step 2: Initial Approximation
Find a number that, when multiplied by itself, gives a product less than or equal to 23. In this case, the number 4 fits as (4^2) equals 16. Divide 23 by 4, giving a quotient of 4 and a remainder of 7.
Step 3: Adjusting the Dividend
Drag a pair of 0s down and append them next to the remainder (7) to create the dividend 700.
Step 4: Iterative Calculation
Double the divisor (4) to get 8. Enter 8 below and leave a blank digit to its right. Guess the largest possible digit (X) to fill in the blank, such that the product of 87 and X is less than or equal to 700. In this case, X equals 7, so fill in 7 in the quotient after the decimal point. Divide and record the remainder.
Step 5: Repeat Iteratively
Repeat the process to obtain further decimal places as needed. Continue until the desired level of precision is achieved.
No, 23 is not a perfect square number
No, 23 is not a perfect square because it cannot be expressed as the square of an integer. The square root of 23 is an irrational number, approximately equal to 4.79583, indicating that it cannot be written as the quotient of two integers.
Understanding squares and square roots is crucial in various mathematical applications, including geometry, algebra, and physics, aiding in problem-solving and mathematical reasoning.
No, the square root of 23 is an irrational number and cannot be represented as a fraction of two integers.
The square root of 23 can be used to calculate the length of the diagonal of a square with side length 23 units.
Yes, the square root of 23 may be used in various real-world scenarios, such as calculating distances, areas, or volumes in engineering and construction projects.
The square root of 23 is related to Pythagorean triples, particularly those involving integers that are multiples of 23.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What is the square of 23?
529
512
543
532
If x² = 23, what is the approximate value of x?
4.5
4.8
4.9
5.0
What is √23 rounded to the nearest whole number?
4
5
6
7
What is the result of 23 × 23?
529
532
543
556
Which of the following is closest to √23?
4.6
4.7
4.8
4.9
What is the value of (√23)²?
23
24
25
26
What is the value of 23 raised to the power of 0.5?
4.5
4.6
4.7
4.8
Find the approximate square root of 23 to one decimal place.
4.7
4.8
4.9
5.0
If √x = 4.8, what is x?
23
24
25
26
Which value is closest to the square of √23?
22
23
24
25
Before you leave, take our quick quiz to enhance your learning!

