Square & Square Root of 243
In mathematics, especially in algebraic studies, squares and square roots are fundamental. Squaring a number, such as 243, involves multiplying it by itself to get 59049, a key operation. It’s crucial for exploring properties of rational (expressible as fractions) and irrational numbers (not neat fractions). Understanding these concepts enriches comprehension of mathematical relationships and patterns. Squares reveal how numbers interact, forming the basis of algebraic analysis. Likewise, square roots unveil the origin of numbers, shedding light on their nature and significance within mathematical frameworks. Mastering these concepts enhances one’s mathematical prowess and analytical skills.
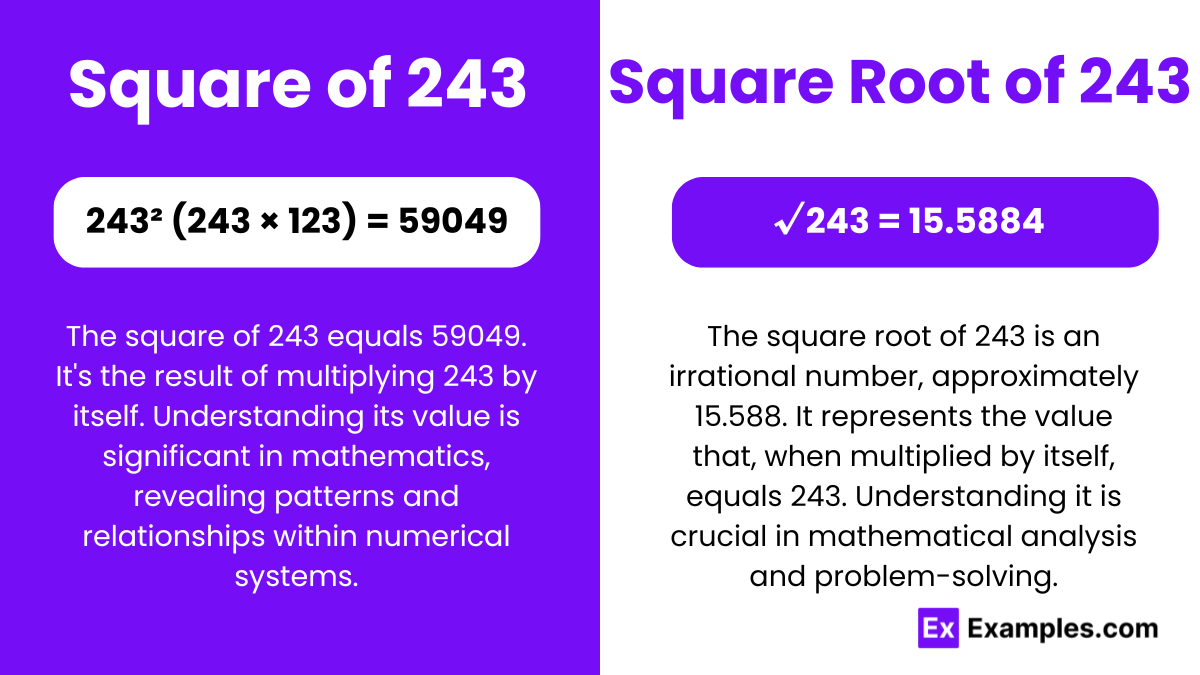
Square of 243
A square number, like 243, is obtained by multiplying a number by itself. The square of 243 equals 59049. Understanding square numbers elucidates their role in algebraic analysis, showcasing their significance in mathematical patterns and relationships, essential for comprehending fundamental mathematical concepts and their applications.
Square Root of 243
√243 = 15.5884
Or
√243 = 15.588 Upto 3 decimals
The square root of 243, when squared, equals 243. It’s the number that, when multiplied by itself, gives 243. The square root, approximately 15.588, unveils the origin of 243. Understanding square roots elucidates their role in algebraic analysis, showcasing their significance in mathematical patterns and relationships.
Square Root of 243: 15.588
Exponential Form: 243^½ or 243^0.5
Radical Form: √243
Is the Square Root of 243 Rational or Irrational?
The square root of 243 is an irrational number. This is because it cannot be expressed as a fraction of two integers. It is approximately equal to 15.5884572681, and its decimal representation goes on indefinitely without repeating or terminating.
Rational Number:
A rational number is expressible as a fraction of two integers, where the denominator is non-zero. It’s represented as a/b, where a and b are integers. Examples include 3/4, -5/2, 0, 1, -2, etc.
Example: Consider 3/4; it’s a fraction with 3 as the numerator and 4 as the denominator. Since both are integers and the denominator isn’t zero, 3/4 is a rational number.
Irrational Number:
An irrational number is a real number that cannot be expressed as a ratio of two integers. Its decimal representation is non-terminating and non-repeating, and it cannot be written as a/b, where a and b are integers and b is not zero. Examples include √2, π, and φ.
Example: Take √2; its decimal expansion is approximately 1.41421356…, non-repeating and infinite. Being unable to be written as a fraction of two integers, √2 is an irrational number.
Methods to Find Value of Root 243
There are several methods to find the value of the square root of 243:
Prime Factorization Method: Express 243 as a product of prime factors (3 × 3 × 3 × 3 × 3). Group pairs of similar factors and take one factor from each pair outside the square root sign. The remaining factors inside the square root represent the square root of 243.
Estimation Method: Make an initial estimate (e.g., 15) and square it (225). Adjust the estimate based on whether the square is greater or less than 243, refining the estimate until it’s close enough.
Long Division Method: Perform long division to find the square root iteratively, similar to the traditional long division method.
Newton’s Method: Utilize iterative approximation using Newton’s method, which involves refining guesses based on the tangent line to the function curve.
Calculator or Software: Use a calculator or mathematical software capable of computing square roots directly.
Each method has its advantages depending on the context and the tools available.
Square Root of 243 by Long Division Method

Square Root of 243 By Long Division
Dividing Digits into Pairs:
Separate the digits into pairs from the right side: 2 and 43.
First Iteration:
Find a number (y) whose square is less than or equal to 2. Since 1² = 1 ≤ 2, y = 1. The quotient and remainder are both 1.
Updating Divisor:
Double the divisor (2y = 2) to add to itself.
Second Iteration:
Bring down the next pair (124) and find a number (d) such that (2d)² ≤ 143. The value of d is 5.
Decimal Placement:
Add a decimal in the dividend and quotient simultaneously. Append three pairs of zeros after the decimal in the dividend.
Continuing Iterations:
Repeat the process for the remaining pairs of zeros.
Result:
The square root of 243, using long division, is approximately 15.588.
243 is Perfect Square root or Not
No, 243 is not a perfect square number
243 is not a perfect square because it cannot be expressed as the square of an integer. Its square root is an irrational number, approximately equal to 15.5884572681.
FAQs
How can I find the square root of 243?
You can find the square root of 243 using various methods like prime factorization, estimation, long division, Newton’s method, or using a calculator or mathematical software.
Why is the square root of 243 irrational?
The square root of 243 is irrational because it cannot be expressed as a fraction of two integers. Its decimal representation goes on indefinitely without repeating or terminating.
Is there a simple way to determine if a number is a perfect square?
Yes, one simple way is to check if the prime factorization of the number contains only even powers. If it does, then the number is a perfect square. If not, then it’s not a perfect square.