Square & Square Root of 2601

Within the domain of algebraic mathematics, squares and square roots hold significant importance. Squaring a number, such as 2601, entails multiplying it by itself, yielding 6,767,401. This foundational operation is vital for exploring rational and irrational numbers. A grasp of these fundamentals enriches understanding of mathematical relationships and patterns. Squares illuminate inherent number properties, while square roots unravel intricate numerical mysteries. These concepts serve as beacons, guiding mathematical explorations into fractional territories. Proficiency in squares and square roots equips mathematicians to navigate varied mathematical landscapes, unveiling the elegance and complexity inherent within algebraic frameworks.

Square of 2601

2601² (2601 × 2601) = 6,767,401

The square number of 2601 equals 6,767,401, obtained by multiplying 2601 by itself, a fundamental operation in algebraic mathematics, uncovering inherent number properties.

Square Root of 2601

√2601 = 51

The square root of 2601 is 51. This fundamental mathematical operation reveals the value that, when multiplied by itself, equals 2601.

Square Root of 2601: 51

Exponential Form: 2601^½ or 2601^0.5
Radical Form: √2601

Is the Square Root of 2601 Rational or Irrational?

The square root of 2601 is rational.

Rational numbers are expressible as the quotient of two integers. Irrational numbers, however, cannot be represented as fractions of integers. Examples of irrational numbers include the square roots of non-perfect squares.

Methods to Find the Value of the Root 2601

• Prime Factorization Method: Break down 2601 into its prime factors. However, since 2601 is a perfect square (51²), its prime factorization results in only the prime factors of 51.
• Long Division Method: Utilize the long division algorithm to directly calculate the square root of 2601.
• Using a Calculator: Most calculators are equipped with a square root function, enabling direct calculation of the square root of 2601.

Square Root of 2601 by Long Division Method

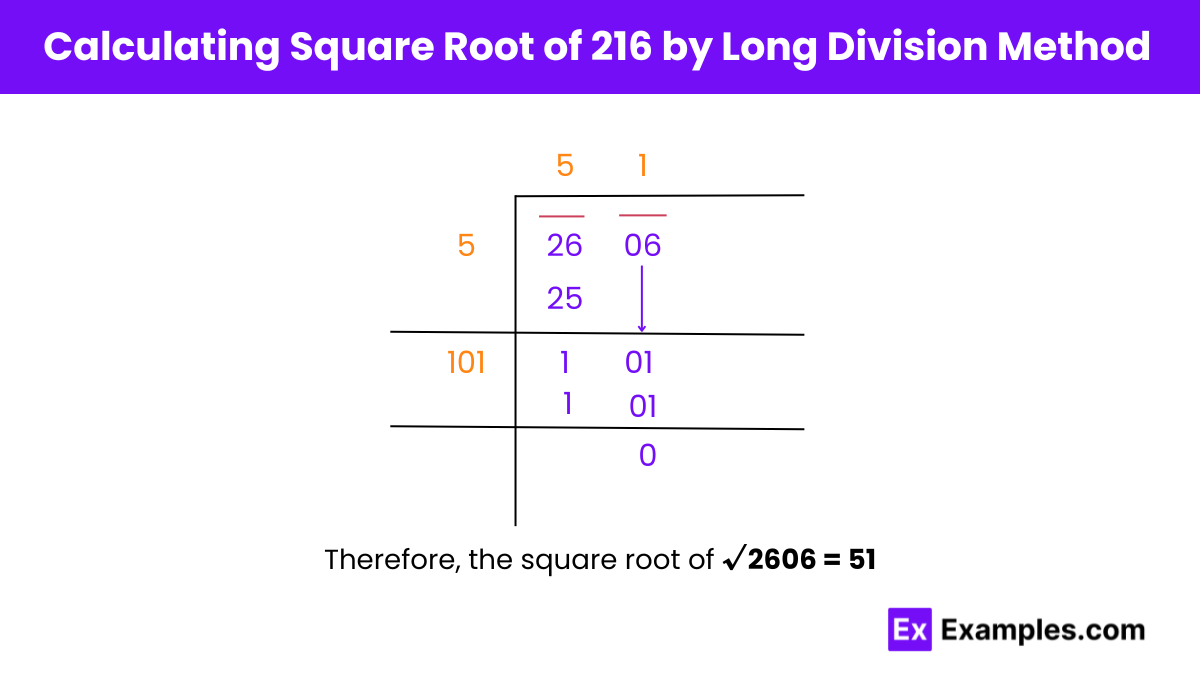
Forming pairs: 26 and 01

Step 1: Find a number (Y) (5) such that its square is less than or equal to 26. (5² = 25).

Step 2: Divide 26 by 5 with the quotient as 5.

Step 3: Bring down the next pair, 01, to the right of the remainder 1. The new dividend is now 101.

Step 4: Add the last digit of the quotient (5) to the divisor (5), i.e., (5 + 5 = 10).

Step 5: To the right of 10, find a digit (Z) (which is 1) such that (10Z × Z ≤ 101). After finding (Z), together 10 and (Z) (1) form a new divisor 101 for the new dividend 101.

Step 6: Divide 101 by 101 with the quotient as 1, giving the remainder (= 101 – 101 × 1 = 101 – 101 = 0).

Step 7: We stop the process since the remainder is now 0 and there are no more digits that can be brought down.

Is 2601 a Perfect Square or Not?

Yes, 2601 is a perfect square.

A perfect square is an integer that can be expressed as the square of another integer. Therefore, the square root of 2601 is 51.