What is the approximate square root of 91?
8.5
9.5
9
10

Within the domain of algebraic mathematics, squares and square roots hold significant importance. Squaring a number, such as 91, entails multiplying it by itself, yielding 8281. This operation is fundamental, pivotal in exploring rational and irrational numbers. Grasping these fundamentals enriches understanding of mathematical relationships and patterns. Squares unveil inherent properties of numbers, while square roots untangle intricate numerical mysteries. These concepts brighten mathematical horizons, guiding exploration into fractional realms. Mastery of squares and square roots enables mathematicians to navigate diverse mathematical landscapes, unveiling the elegance and complexity woven within algebraic frameworks.
The square of 91 equals 8,281, derived by multiplying 91 by itself, showcasing a fundamental operation in algebraic mathematics and revealing inherent properties of numbers.
Or
√91 ≈ 9.539 upto 3 decimals
The square root of 91 is approximately 9.53939. This fundamental operation in mathematics reveals the value that, when multiplied by itself, equals 91.
Square Root of 91 ≈ 9.53939
Exponential Form: 91^½ or 91^0.5
Radical Form: √91
Rational Numbers: Rational numbers can be expressed as the quotient of two integers, where the denominator is not zero. Examples include integers and fractions.
Irrational Numbers: Irrational numbers cannot be expressed as fractions of integers. Examples include the square roots of non-perfect squares.
Prime Factorization Method: Break down 91 into its prime factors to find its square root.
Long Division Method: Utilize the long division algorithm to approximate the square root of 91.
Using a Calculator: Most calculators feature a square root function to directly compute the square root of 91.
Estimation: Since 91 is close to the perfect square of 81 (9 × 9) and 100 (10 × 10), estimate its square root to be between 9 and 10.
Step 1: Write 91 with pairs of zeros after the decimal point as 91.000000.
Step 2: Find a number that, when squared, gives 91 or less. 9 × 9 = 81, leaving a remainder of 10.
Step 3: Bring down the next pair of zeros. 1000 becomes the new dividend.
Step 4: Double the quotient. It is 18. Write 180 in the place of the new divisor.
Step 5: Find a number such that (180 + number) × number ≤ 1000. We determine that 180 + 5 = 185 and 184 × 5 = 920.
Step 6: Subtract 920 from 1000. The result is 80, and we bring down the next pair of zeros, making the new dividend 8000.
Step 7: Repeat the process until the approximation is accurate to 3 decimal places.
Thus, √91 ≈ 9.539.
91 is not a perfect square because it cannot be expressed as the square of an integer.
The number closest to the square root of 91 is 9.539, rounded to three decimal places.
The square root of 91 is approximately 9.539338, so the negative square root of 91 would be approximately -9.539338.
The factors of 91 are 1, 7, 13, and 91, as it can be divided evenly by these integers without leaving a remainder.
The cube root of 91 is approximately 4.497941.

Within the domain of algebraic mathematics, squares and square roots hold significant importance. Squaring a number, such as 91, entails multiplying it by itself, yielding 8281. This operation is fundamental, pivotal in exploring rational and irrational numbers. Grasping these fundamentals enriches understanding of mathematical relationships and patterns. Squares unveil inherent properties of numbers, while square roots untangle intricate numerical mysteries. These concepts brighten mathematical horizons, guiding exploration into fractional realms. Mastery of squares and square roots enables mathematicians to navigate diverse mathematical landscapes, unveiling the elegance and complexity woven within algebraic frameworks.
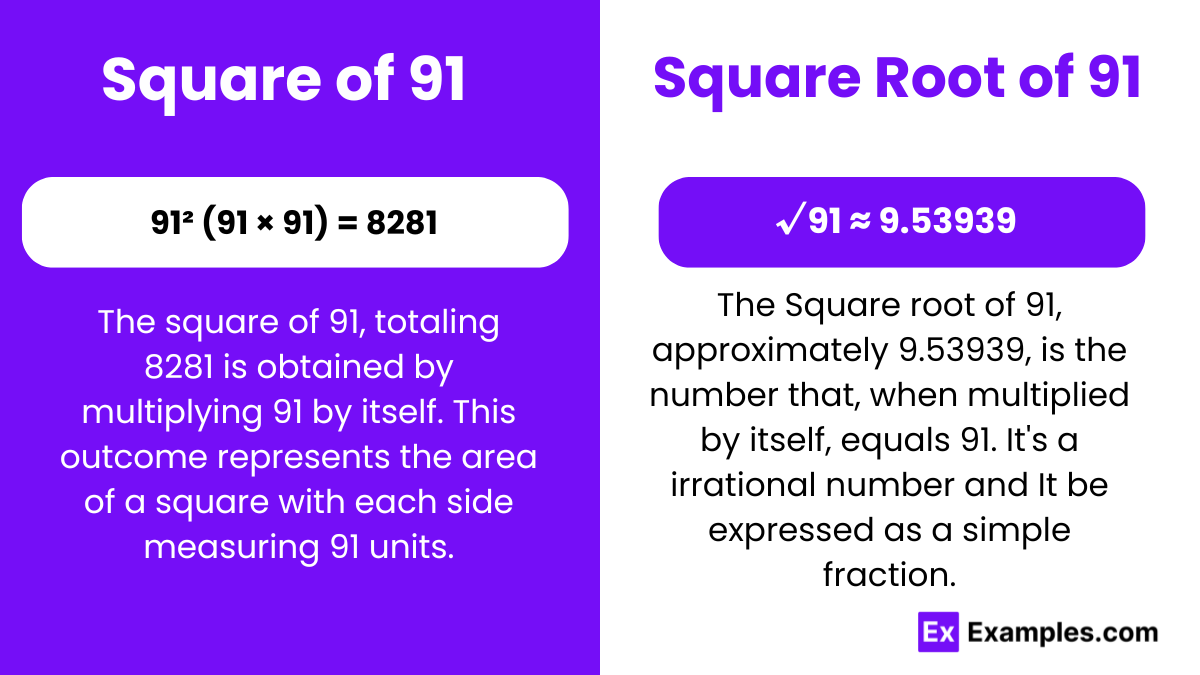
91² (91 × 91) = 8281
The square of 91 equals 8,281, derived by multiplying 91 by itself, showcasing a fundamental operation in algebraic mathematics and revealing inherent properties of numbers.
√91 ≈ 9.53939
Or
√91 ≈ 9.539 upto 3 decimals
The square root of 91 is approximately 9.53939. This fundamental operation in mathematics reveals the value that, when multiplied by itself, equals 91.
Square Root of 91 ≈ 9.53939
Exponential Form: 91^½ or 91^0.5
Radical Form: √91
The square root of 91 is irrational.
Rational Numbers: Rational numbers can be expressed as the quotient of two integers, where the denominator is not zero. Examples include integers and fractions.
Irrational Numbers: Irrational numbers cannot be expressed as fractions of integers. Examples include the square roots of non-perfect squares.
Prime Factorization Method: Break down 91 into its prime factors to find its square root.
Long Division Method: Utilize the long division algorithm to approximate the square root of 91.
Using a Calculator: Most calculators feature a square root function to directly compute the square root of 91.
Estimation: Since 91 is close to the perfect square of 81 (9 × 9) and 100 (10 × 10), estimate its square root to be between 9 and 10.

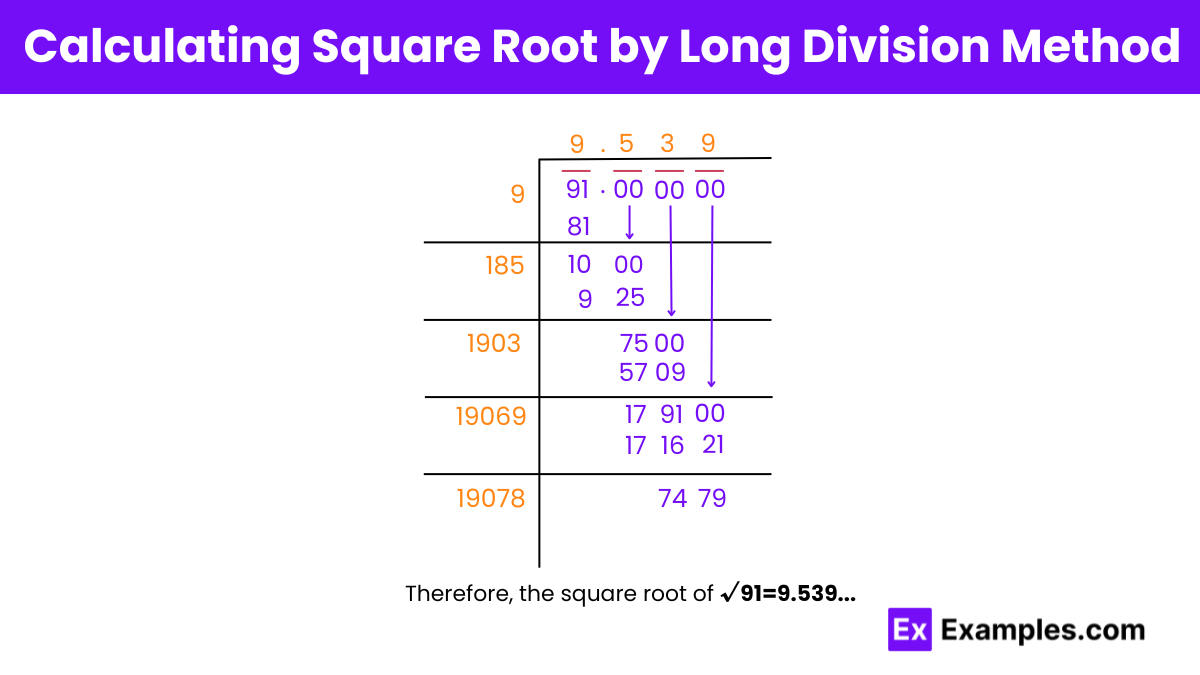
Step 1: Write 91 with pairs of zeros after the decimal point as 91.000000.
Step 2: Find a number that, when squared, gives 91 or less. 9 × 9 = 81, leaving a remainder of 10.
Step 3: Bring down the next pair of zeros. 1000 becomes the new dividend.
Step 4: Double the quotient. It is 18. Write 180 in the place of the new divisor.
Step 5: Find a number such that (180 + number) × number ≤ 1000. We determine that 180 + 5 = 185 and 184 × 5 = 920.
Step 6: Subtract 920 from 1000. The result is 80, and we bring down the next pair of zeros, making the new dividend 8000.
Step 7: Repeat the process until the approximation is accurate to 3 decimal places.
Thus, √91 ≈ 9.539.
No, 91 is not a perfect square
91 is not a perfect square because it cannot be expressed as the square of an integer.
The number closest to the square root of 91 is 9.539, rounded to three decimal places.
The square root of 91 is approximately 9.539338, so the negative square root of 91 would be approximately -9.539338.
The factors of 91 are 1, 7, 13, and 91, as it can be divided evenly by these integers without leaving a remainder.
The cube root of 91 is approximately 4.497941.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What is the approximate square root of 91?
8.5
9.5
9
10
If x² = 91, what is the value of x?
±9
±10
±8.5
±9.5
Which of the following is true about the square of 91?
It is 8281
It is 8100
It is 9000
It is 9001
How much more is the square of 92 than the square of 91?
183
91
1830
182
What is the closest integer greater than the square root of 91?
8
9
10
11
What is the value of 91 raised to the power of 1/2?
9
91
8.5
10
Which option approximates the square root of 91 when rounded to two decimal places?
9.13
9.54
9.45
9.00
What is the approximate square root of 91 expressed as a fraction?
9/1
10/1
19/2
3/2
If the square root of 91 is represented by x, then x² equals what?
91
9
23
27
If you square the square root of 91, what do you get?
1
0
91
9
Before you leave, take our quick quiz to enhance your learning!

