Squares

What is a Square? – definition

A square is a fundamental shape in geometry that boasts an array of intriguing properties. Defined by its four equal-length sides and four right angles, a square stands out as a special type of rectangle where all sides have the same measurement. This unique characteristic gives rise to its distinctive symmetry and balance, making it a foundational element in both mathematics and various applications in daily life.

Basic Formulas Related to Squares

Download This Image

Area of a Square

• Formula: Area of square=side²
• Explanation: The area of a square is calculated by squaring the length of one of its sides. This formula arises from the fact that a square has all sides of equal length, and the area is essentially the amount of space enclosed within its boundaries.

Perimeter of a Square

• Formula: Perimeter=4×side
• Explanation: The perimeter of a square is the total length of its four sides. Since all sides of a square are equal, the perimeter is simply four times the length of one side. The perimeter represents the boundary length of the square.

Diagonal of a Square

• Formula:Diagonal=side×√2​​
• Explanation: The length of the diagonal of a square can be calculated using the Pythagorean theorem, given that the diagonal splits the square into two right-angled triangles. This formula reflects the diagonal’s length as a function of the square’s side, highlighting the square’s inherent symmetry.

Circumference of the Circumscribed Circle

• Formula: Circumference=2π×diagonal/2​​
• Explanation: The circumscribed circle is the circle that passes through all four vertices of the square. Its circumference can be calculated by using the diameter, which is the same as the diagonal of the square, indicating the circle’s boundary length.

Area of the Circumscribed Circle

• Formula:Area=π×(diagonal/2​)²
• Explanation: Similar to the circumference, the area of the circumscribed circle can be determined using the diagonal of the square, which acts as the diameter of the circle. This formula calculates the space enclosed within the circumscribed circle.

Properties of a Square

A square, a fundamental figure in geometry, is celebrated for its simplicity and symmetry. It is defined as a quadrilateral with four equal sides and four right angles. The properties of a square make it a unique and special case among polygons, particularly rectangles.

Equal Length Sides

• Uniformity of Sides: Each side of a square has the exact same length, denoting a perfect symmetry and balance. This equality of sides sets the square apart as a regular polygon, where all sides and angles are equal.

Right Angles

• Orthogonal Corners: A square features four right angles, each measuring exactly 90 degrees. This property ensures that a square is not only a rectangle but also a figure of precise regularity and orthogonality.

Equal Diagonals

• Diagonal Properties: The diagonals of a square are of equal length, intersect at the center, and divide each other into two equal parts. Moreover, they are perpendicular to each other, further highlighting the square’s symmetry.

Congruent Diagonals

• Diagonal Symmetry: Beyond their equality and perpendicularity, the diagonals of a square bisect its angles. This means that they cut the square’s angles in half, ensuring that the symmetry of the square extends to its diagonals as well.

Area and Perimeter Formulas

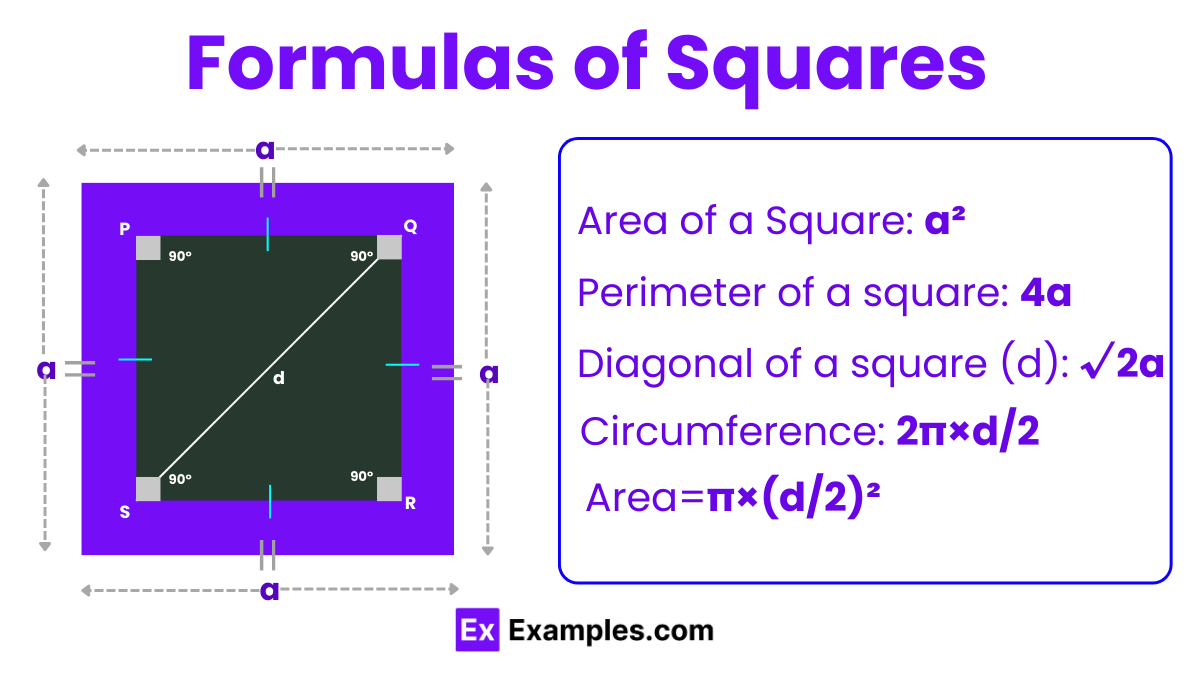
• Calculating Area: The area of a square is found by squaring the length of one of its sides (Area = side²). This straightforward formula is a direct consequence of its equal-sided property.
• Determining Perimeter: The perimeter of a square, which is the total length of its boundaries, is calculated as four times the length of one side (Perimeter = 4 × side), a reflection of its uniformity.

Circumscribed and Inscribed Circles

• Circumscribed Circle: A square has a circumscribed circle (a circle that passes through all its vertices), highlighting its rotational symmetry.
• Inscribed Circle: Similarly, a square can inscribe a circle (a circle contained within the square touching all its sides), demonstrating its central symmetry.

Area of a Square

The area of a square is calculated by squaring the length of one of its sides. Since all sides of a square are equal, the formula is quite straightforward:

$²$

For example, if one side of a square is 6 units, the area is 6² = 36 square units.

Perimeter of a Square

The perimeter of a square is the total length of all its sides. Given that a square has four sides of equal length, the formula to calculate the perimeter is:

Perimeter=4×side

So, if the length of a side is 6 units, the perimeter would be 4×6=24units.

Diagonal of a Square

The diagonal of a square connects two opposite corners, passing through the square’s center. The length of the diagonal can be found using the Pythagorean theorem, given that a diagonal splits the square into two right triangles. The formula is:

$\surd 2$

Therefore, if the side of the square is 6 units, the length of the diagonal is $\surd 2$ units.

Practice Questions on the Topic of Squares

Question 1

Calculate the area of a square whose side length is 8 units.

Answer: Use the formula for the area of a square, A = side$²$

Thus, A = 8$²\; =\; 64\; square\; units.$

Question 2

A square has a perimeter of 48 units. Find the length of one side.

Answer: The formula for the perimeter of a square is $P=4\times \text{side}$.

Thus, side = P/4 = 48/4 = 12unit

Question 3

If the diagonal of a square is 14 units long, what is the area of the square?

Answer: The formula relating the diagonal (d) of a square to its side (s) is d = s√2

So, s = d/√2 = 14/√2 = 7√2units
Then , The area is A= s$²\; =\; (7\surd 2)²=98Square\; unit.$

Question 4

How long is the diagonal of a square with a side length of 5 units?

Answer: Use the diagonal formula, d = s$\surd 2.$

d=5√2​ ≈ 7.07 units.

Question 5

The area of a square is 81 square units. What is the perimeter of this square?

Answer: First, find the side length: s$²\; =\; 81,\; So\; s\; =\; 9units.$

Then, calculate the perimeter: P= 4×s = 4×9 = 36 units.

Question 6

If the area of a square is equal to the square of its perimeter, what is the side length of the square?

Answer: Let $s$ be the side length. The area is s$²$, and the perimeter is $4s$. According to the question, s$²\; =\; (4s)²$. Simplifying, s$²\; =\; 16s²$ , which is not possible under normal circumstances since it implies s = 0 for any non-zero coefficient. This question seems to be incorrectly stated as it suggests an impossible scenario under conventional geometric rules.

Short Type Questions and Answers

1. What is the formula for the area of a square?
   • A= side$²$
2. How do you calculate the perimeter of a square?
   • P = 4 × side
3. If a square has an area of 64 square units, what is the length of one side?
   • Side length = 8 units
4. What is the perimeter of a square whose area is 49 square units?
   • Perimeter = 28 units
5. How long is the diagonal of a square with a perimeter of 20 units?
   • $Diagonal=5²\approx 7.07$ units
6. If one side of a square is 10 units, what is its area?
   • Area = 100 square units
7. A square has a diagonal of 102102​ units. What is its perimeter?
   • Perimeter = 40 units
8. What is the area of a square with a diagonal length of 8 units?
   • Area = 32 square units
9. If the perimeter of a square is 16 units, what is the length of its diagonal?
   • Diagonal = 4√2≈ 5.66units

FAQs

Is A Diamond a Square?

A diamond shape, often referred to in geometry as a rhombus, is not necessarily a square. While both have four sides, a square requires all angles to be 90 degrees, which is not a requirement for a diamond. The key difference lies in the angles.

Is A Rhombus a Square?

A rhombus is not always a square, but a square is a type of rhombus. The distinction hinges on the angles; a square has four right angles, whereas a rhombus has equal sides but not necessarily equal angles. A square meets all rhombus criteria and the additional requirement of right angles.

Is A Square A Parallelogram?

Yes, a square is a type of parallelogram. It not only has opposite sides that are parallel and equal in length, but it also has all four angles equal to 90 degrees. A square meets all the criteria of a parallelogram, with the added feature of equal angles and sides.

How Can I Learn Squares Easily?

Learning squares easily involves practice and pattern recognition. Start by memorizing small squares as a base. Notice patterns, such as the last digit cycle for squares of numbers ending in 5, and use multiplication shortcuts for quick calculation. Regular practice enhances recall.