Balancing chemical equations is essential in understanding chemical reactions, ensuring that the law of conservation of mass is followed. On the MCAT, you’ll explore how to balance equations by adjusting coefficients, recognizing reactants and products, and identifying reaction types. Mastering this topic is fundamental to solving stoichiometry problems and understanding reaction mechanisms, both of which are key to excelling in the chemistry and biochemistry sections of the exam.
Learning Objectives
In studying "Balancing Chemical Equations" for the MCAT, you should learn to apply the law of conservation of mass by ensuring that the number of atoms for each element is the same on both sides of the equation. Understand the steps involved in balancing equations systematically, including identifying reactants and products, balancing atoms sequentially, and adjusting coefficients appropriately. Analyze chemical reactions to classify them into types, such as combination, decomposition, single displacement, and double displacement. Evaluate the stoichiometric relationships between reactants and products to predict the quantities needed or produced in a reaction. Additionally, develop problem-solving strategies to balance more complex equations efficiently, especially in biological and biochemical processes relevant to the MCAT.
Applying the Law of Conservation of Mass
The law of conservation of mass states that matter cannot be created or destroyed in a chemical reaction, only rearranged. This principle is fundamental in chemistry and applies to both physical and chemical processes, ensuring that the mass of the products in a reaction equals the mass of the reactants.
Understanding the Law of Conservation of Mass
Balanced Chemical Reactions: The total mass of all reactants must equal the total mass of all products. This is why chemical equations must be balanced, with the same number of atoms for each element on both sides of the equation.
Rearrangement of Atoms: Atoms are neither created nor destroyed but are rearranged to form new substances in chemical reactions.
Examples of Conservation of Mass in Real Life
1. Combustion Reactions
Example: When wood burns, the mass of the ash, gases (like carbon dioxide and water vapor), and heat released equals the mass of the original wood and the oxygen used during combustion.
This principle helps calculate the amount of fuel needed in engines and furnaces.
2. Chemical Manufacturing
Example: In the synthesis of ammonia using the Haber process, nitrogen and hydrogen react to form ammonia. The total mass of nitrogen and hydrogen used equals the mass of the ammonia produced.
Ensures efficient use of raw materials and helps manufacturers predict product yields accurately.
3. Cooking and Baking
Example: When ingredients like flour, sugar, and eggs are mixed and baked, their total mass (after accounting for gases like steam) remains equal to the final baked product.
In food industries, ensuring the right amount of ingredients helps maintain product consistency and avoid waste.
4. Environmental Science – Carbon Cycle
Example: Plants absorb carbon dioxide during photosynthesis and release it back through respiration. The total amount of carbon remains constant in the ecosystem, following the law of conservation of mass.
Helps scientists track carbon emissions and absorption to manage climate change.
5. Waste Management and Recycling
Example: In waste treatment, the mass of pollutants removed must match the mass of chemicals used for treatment plus any residual waste.
Ensures efficient and sustainable recycling processes.
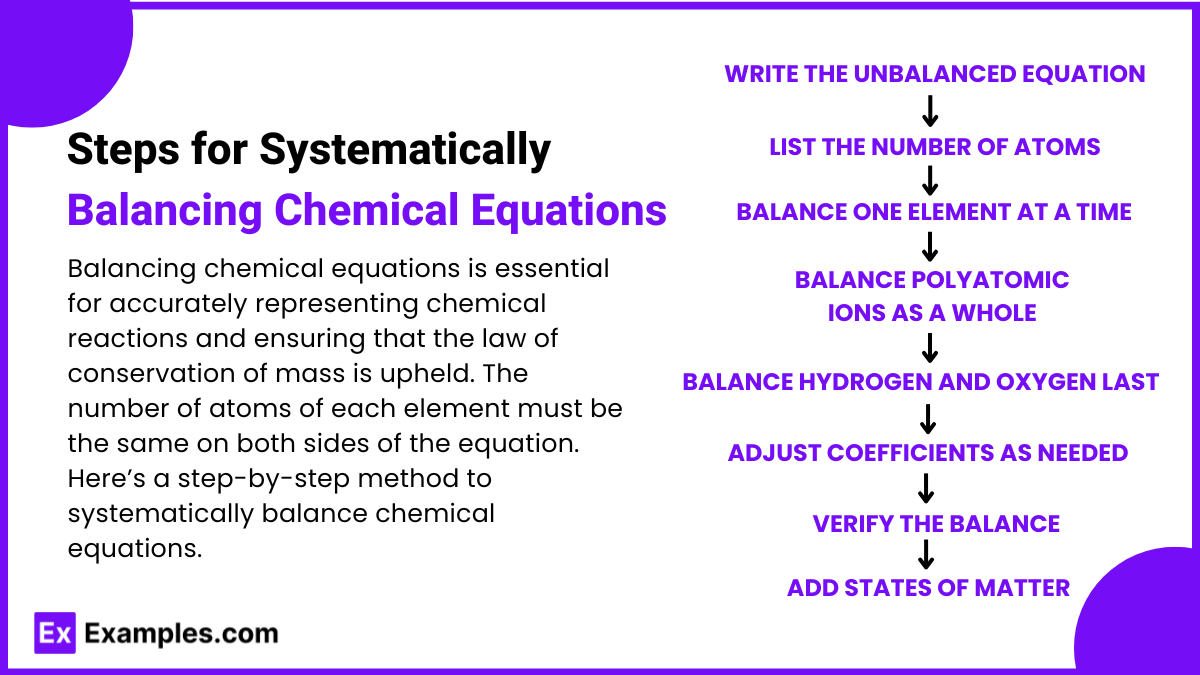
Steps for Systematically Balancing Chemical Equations
Balancing chemical equations is essential for accurately representing chemical reactions and ensuring that the law of conservation of mass is upheld. The number of atoms of each element must be the same on both sides of the equation. Here’s a step-by-step method to systematically balance chemical equations.
Steps for Balancing Chemical Equations
1. Write the Unbalanced Equation
Start by writing the correct formulas for all the reactants and products.
Do not change any subscripts in the chemical formulas—only coefficients will be adjusted to balance the equation.
2. List Elements and Count Atoms
Identify all the elements present in the reactants and products.
Count the number of atoms of each element on both sides of the equation.
3. Balance One Element at a Time
Begin with elements that appear in only one reactant and one product. Balance these elements first by placing appropriate coefficients in front of their formulas.
Adjust the coefficients to make the number of atoms of the element equal on both sides.
4. Balance Polyatomic Ions as a Whole (if possible)
If the same polyatomic ion appears on both sides of the equation, treat it as a unit rather than balancing individual atoms within the ion.
5. Balance Hydrogen and Oxygen Last
In many reactions, hydrogen and oxygen appear in multiple reactants and products. Save these for last to avoid unnecessary adjustments.
6. Adjust Coefficients as Needed
If adjusting one coefficient affects other elements, go back and adjust other coefficients as necessary. Balancing equations often requires multiple iterations.
7. Verify the Balance
Ensure that the number of atoms for each element is the same on both sides of the equation.
Double-check that all coefficients are in the smallest possible whole-number ratio.
8. Add States of Matter (Optional)
Indicate the physical state of each substance (solid (s), liquid (l), gas (g), or aqueous (aq)) if required.
Strategies for Balancing Complex Equations Efficiently
Balancing complex chemical equations can be challenging, especially when multiple elements or polyatomic ions are involved. However, by applying strategic approaches, the process can become more manageable and systematic. Here are several effective strategies to balance complex equations efficiently.
Key Strategies for Balancing Complex Equations
1. Balance Elements One at a Time (Start with Single Elements)
Approach: Start by balancing elements that appear in only one reactant and one product. This prevents unnecessary adjustments later in the process.
Example: If carbon appears in only one reactant and one product, balance it first before dealing with more complex molecules.
2. Balance Polyatomic Ions as a Unit (If They Appear Intact)
Approach: If the same polyatomic ion appears on both sides of the equation, treat the entire ion as a unit rather than balancing its individual atoms. This simplifies the process by reducing the number of variables.
Example: In an equation involving sulfate ions (SO₄²⁻), treat SO₄²⁻ as a single entity instead of balancing sulfur and oxygen separately.
3. Balance Compounds Containing the Most Elements First
Approach: If a compound contains several different elements, balance it first to minimize the need for further adjustments.
Example: In combustion reactions, start by balancing hydrocarbons (which contain both carbon and hydrogen) before balancing simpler molecules like O₂.
4. Save Hydrogen and Oxygen for Last
Approach: Hydrogen and oxygen often appear in multiple reactants and products, making them harder to balance initially. Focus on balancing other elements first, then tackle hydrogen and oxygen last.
Example: In combustion reactions, balance the hydrocarbon and then address oxygen (O₂) and water (H₂O) at the end.
5. Use Fractional Coefficients for Complex Molecules (Then Clear the Fraction)
Approach: If you encounter difficulty balancing certain elements, consider using fractional coefficients (for example, ½) to balance the equation temporarily. Once the equation is balanced, multiply the entire equation by the denominator to convert all coefficients to whole numbers.
Example: In balancing oxygen atoms in combustion reactions, fractional coefficients can simplify the process.
6. Check for Conservation of Charge (in Ionic Reactions)
Approach: In ionic equations, ensure that the total charge is balanced on both sides of the reaction, in addition to balancing the atoms.
Example: For redox reactions, ensure that the sum of charges on the reactants and products is equal after balancing the atoms.
7. Use the Algebraic Method for Highly Complex Equations
Approach: For very complex reactions, assign a variable to each coefficient (e.g., aaa, bbb, ccc) and create a system of linear equations based on the number of atoms of each element. Solve the system to determine the appropriate coefficients.
Example: This approach is useful for reactions with many reactants and products or when balancing oxidation-reduction (redox) reactions.
8. Redox Reactions: Use the Half-Reaction Method
Approach: For redox reactions, split the reaction into two half-reactions—one for oxidation and one for reduction. Balance each half-reaction separately for both mass and charge, then combine them to obtain the overall balanced equation.
Example: In redox reactions in acidic or basic solutions, balance oxygen using water (H₂O) and hydrogen using hydrogen ions (H⁺) in acidic solutions or hydroxide ions (OH⁻) in basic solutions.
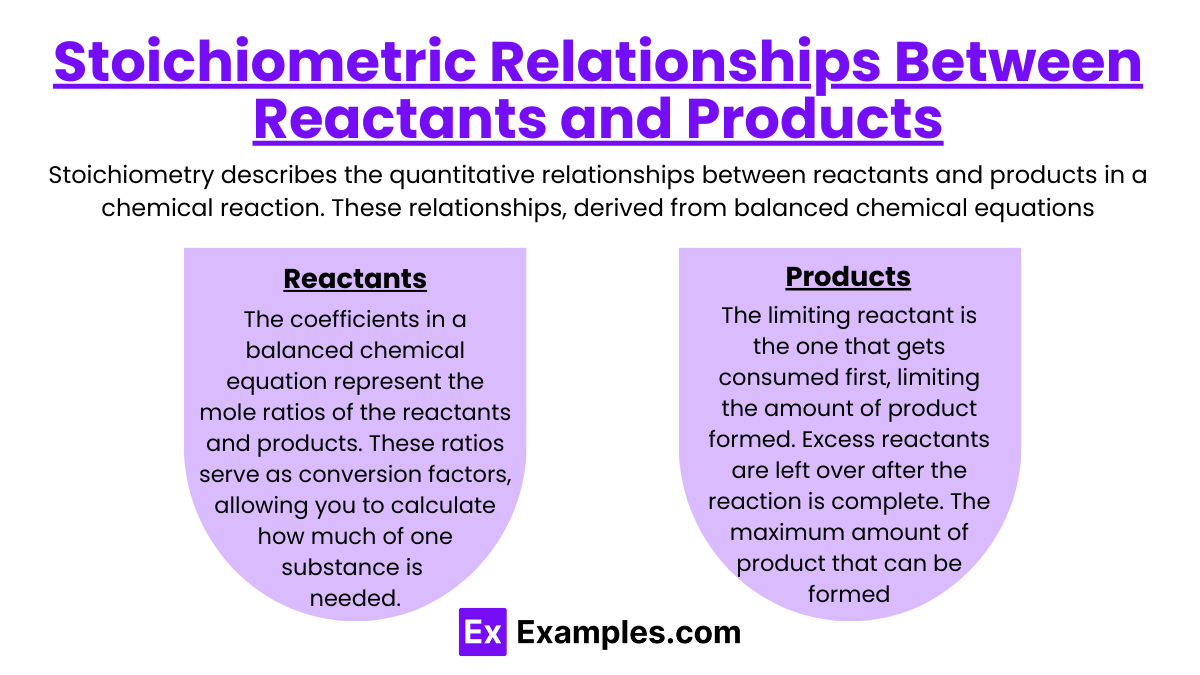
Stoichiometric Relationships Between Reactants and Products
Stoichiometry describes the quantitative relationships between reactants and products in a chemical reaction. These relationships, derived from balanced chemical equations, help determine the precise amounts of substances required or produced, ensuring that no reactants are wasted and the reaction occurs efficiently.
Key Stoichiometric Concepts
Mole Ratios:
The coefficients in a balanced chemical equation represent the mole ratios of the reactants and products.
These ratios serve as conversion factors, allowing you to calculate how much of one substance is needed or produced based on the amount of another.
Balanced Chemical Equation:
A balanced equation ensures the same number of atoms for each element on both sides, following the law of conservation of mass.
Example: 2H2+O2→2H2O This tells us that for every 2 moles of hydrogen gas (H2), 1 mole of oxygen gas (O2) is required, producing 2 moles of water (H2O).
Limiting and Excess Reactants:
The limiting reactant is the one that gets consumed first, limiting the amount of product formed.
Excess reactants are left over after the reaction is complete.
Theoretical Yield:
The maximum amount of product that can be formed, based on the amount of limiting reactant used.
Actual Yield and Percent Yield:
The actual yield is the amount of product obtained from an experiment.
Percent yield measures the efficiency of a reaction: Percent Yield = (Actual Yield/Theoretical Yield) × 100
Stoichiometric Calculations: Reactant-Product Relationships
1. Mole-to-Mole Conversions
Use mole ratios from the balanced equation to determine the relationship between reactants and products.
Example:
Given: 4 moles of hydrogen gas (H2)
Find: Moles of water (H2O) produced.
From the equation 2H2+O2→2H2O, the ratio is 2:2.
Therefore, 4 moles of hydrogen will produce 4 moles of water.
Examples
Example 1: Combustion of Methane
In the combustion of methane, methane reacts with oxygen to produce carbon dioxide and water. Balancing this equation involves ensuring that the number of carbon, hydrogen, and oxygen atoms is the same on both sides. This process illustrates how energy is released during combustion reactions.
Example 2: Formation of Ammonia
The Haber process combines nitrogen and hydrogen to produce ammonia. To balance this equation, coefficients are adjusted so that the number of nitrogen and hydrogen atoms matches the number of ammonia molecules produced. This balanced equation is crucial for understanding industrial ammonia synthesis.
Example 3: Photosynthesis
In photosynthesis, plants convert carbon dioxide and water into glucose and oxygen. Balancing this reaction requires determining the correct coefficients to reflect the quantities of each reactant and product involved. This balanced equation represents the fundamental process that sustains life on Earth.
Example 4: Decomposition of Hydrogen Peroxide
When hydrogen peroxide decomposes, it breaks down into water and oxygen gas. Balancing this reaction ensures that the number of hydrogen and oxygen atoms is equal on both sides. This reaction is often used in laboratories and demonstrates the principles of decomposition reactions.
Example 5: Neutralization Reaction
In a typical acid-base neutralization, hydrochloric acid reacts with sodium hydroxide to form sodium chloride and water. Balancing this equation confirms that the quantities of each type of atom remain constant before and after the reaction. This example highlights the importance of balancing in acid-base chemistry.
Practice Questions
Question 1
Why is it important to balance a chemical equation?
A) To ensure the reaction occurs at a faster rate
B) To comply with the law of conservation of mass
C) To determine the temperature of the reaction
D) To calculate the energy released during the reaction
Correct Answer: B) To comply with the law of conservation of mass.
Explanation: Balancing a chemical equation is essential because it reflects the law of conservation of mass, which states that matter cannot be created or destroyed in a chemical reaction. This means that the total number of atoms of each element must be the same on both sides of the equation. Options A, C, and D do not accurately describe the primary reason for balancing chemical equations.
Question 2
In a balanced chemical equation, what do the coefficients represent?
A) The temperature of the reaction
B) The energy change during the reaction
C) The number of moles of each substance involved
D) The volume of gas produced
Correct Answer: C) The number of moles of each substance involved.
Explanation: In a balanced chemical equation, the coefficients indicate the relative number of moles of each reactant and product involved in the reaction. For example, if a coefficient of "2" is present before a reactant, it means two moles of that reactant are required. Understanding this concept is vital for accurately calculating reactant quantities and predicting product yields. Options A, B, and D do not relate to the meaning of coefficients in a balanced equation.
Question 3
Which of the following statements is true regarding balancing chemical equations?
A) Balancing only applies to the reactants in a chemical reaction.
B) The total mass of reactants must equal the total mass of products.
C) Only the products need to be balanced, not the reactants.
D) Coefficients can only be added, not adjusted.
Correct Answer: B) The total mass of reactants must equal the total mass of products.
Explanation: The correct statement is that in a balanced chemical equation, the total mass of the reactants must equal the total mass of the products. This reflects the law of conservation of mass. Balancing applies to both reactants and products, ensuring that the number of atoms of each element is conserved. Option A is incorrect as both sides must be balanced, option C is misleading since both reactants and products are involved, and option D is incorrect because coefficients can be adjusted to achieve balance.