Work and energy

Work and energy are fundamental concepts in physics, essential to understanding the transfer and transformation of energy in various systems. On the MCAT, you’ll explore topics such as kinetic and potential energy, the work-energy theorem, and conservation of energy principles. Grasping these ideas is crucial, as they connect to concepts like thermodynamics, mechanical systems, and biological processes, which are key components of the exam’s physics and biochemistry sections.

Learning Objectives

In studying “Work and Energy” for the MCAT, you should learn to define work, energy, and power, understanding their significance in physical systems. Recognize the differences between kinetic and potential energy, including gravitational and elastic potential energy. Apply the work-energy theorem, which connects the net work done on an object to changes in its kinetic energy. Analyze the concepts of conservative and non-conservative forces, focusing on how they affect energy conservation within a system. Understand the principles of mechanical energy conservation and practice solving problems that involve energy transformations. Evaluate power as the rate of doing work and learn to calculate work done by variable forces. Additionally, develop proficiency in identifying real-world scenarios where energy principles apply, such as in biological processes and human movement.

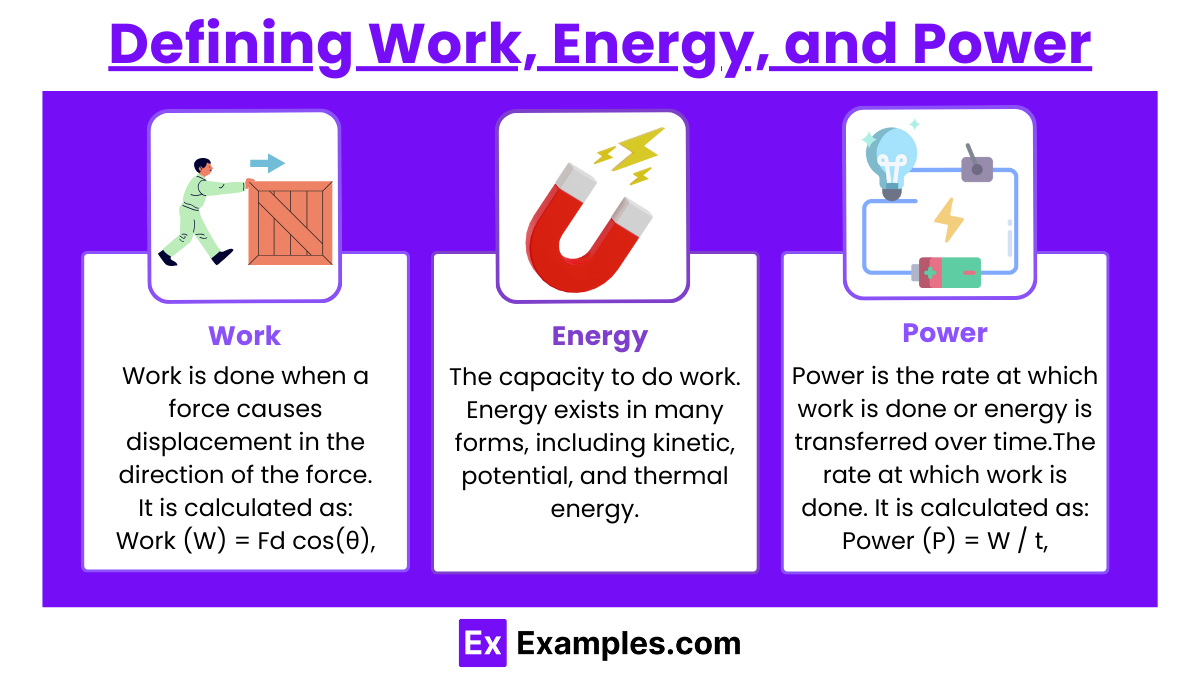
Defining Work, Energy, and Power

Work: Work is done when a force causes displacement in the direction of the force. It is calculated as:
Work (W) = Fd cos(θ),
where F is the applied force, d is the displacement, and θ is the angle between the force and displacement vector.

Example: Pushing a box with a force of 10 N over a distance of 5 meters at an angle of 0° (force parallel to displacement) results in W = (10)(5) cos⁡(0) = 50 J.

Energy: The capacity to do work. Energy exists in many forms, including kinetic, potential, and thermal energy.

Power: Power is the rate at which work is done or energy is transferred over time. The rate at which work is done. It is calculated as:
Power (P) = W / t,
where W is the work done and t is the time over which the work is performed.

Example: If 100 J of work is done in 10 seconds, the power output is P = 100/10 = 10 W.

Conservative and Non-Conservative Forces

Conservative Forces

Conservative forces are those where the work done in moving an object between two points is independent of the path taken. Crucially, the work done by a conservative force on a closed path (returning to the starting point) is zero. This means that these forces do not dissipate mechanical energy but rather transform it from one form to another (e.g., from potential energy to kinetic energy and vice versa).

Characteristics of Conservative Forces:

• Path Independence: The total work done by a conservative force is the same regardless of the path between two points.
• Potential Energy: Conservative forces are associated with potential energy. The change in potential energy is defined only by the initial and final positions in a field, such as gravitational potential energy or elastic potential energy.
• Examples: Gravity, spring force, and electrostatic force.

Non-Conservative Forces

Non-conservative forces are those where the work done depends on the path taken. Work done by non-conservative forces on a closed path is not zero, implying that they can cause a net loss or gain of mechanical energy by converting it to other forms like heat or light, which are not recoverable within the system.

Characteristics of Non-Conservative Forces:

• Path Dependence: The work done by non-conservative forces is path-dependent, meaning the energy transferred by these forces varies depending on the route taken.
• Energy Dissipation: These forces generally convert mechanical energy into other energy forms that are not recoverable as mechanical energy within that system, such as friction converting kinetic energy into thermal energy.
• Examples: Friction, air resistance, and muscular force.

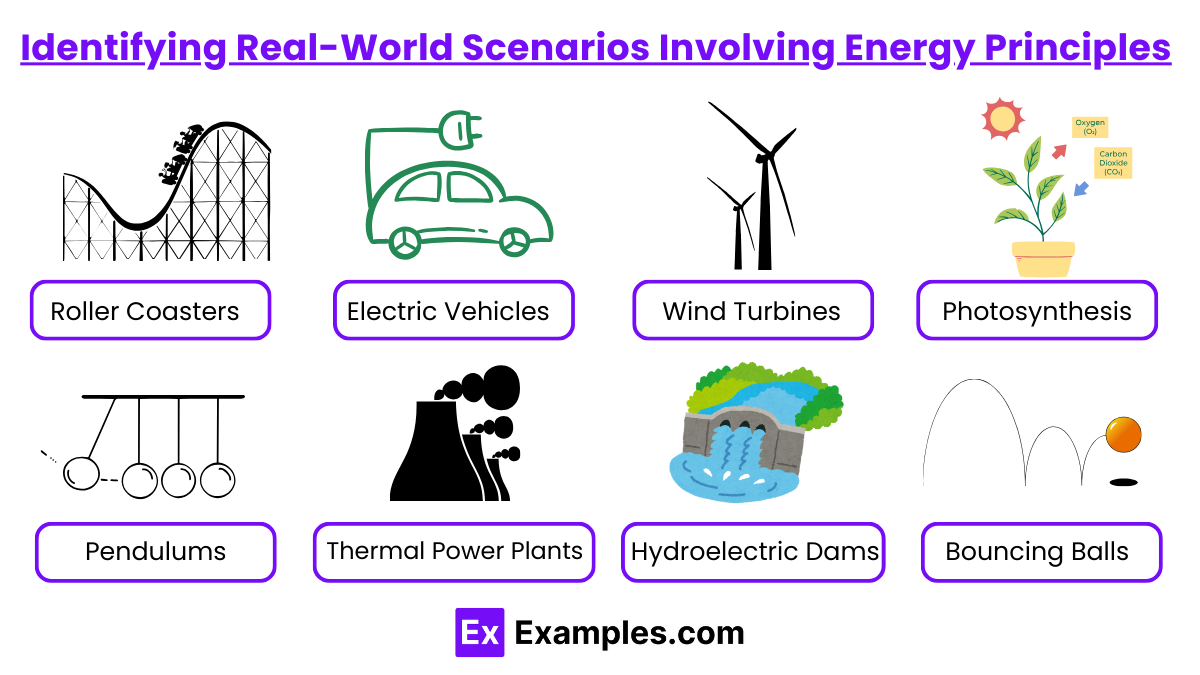
Identifying Real-World Scenarios Involving Energy Principles

1. Roller Coasters

• Energy Principle: Conservation of mechanical energy (kinetic and potential energy).
• As the roller coaster climbs to the top of a track, its potential energy increases, reaching a maximum at the peak. As it descends, this potential energy is converted into kinetic energy, causing it to accelerate. If friction and air resistance are negligible, the mechanical energy (sum of potential and kinetic energy) remains approximately constant.

2. Electric Vehicles

• Energy Principle: Conservation of energy and energy efficiency.
• Electric vehicles (EVs) convert electrical energy stored in batteries into mechanical energy to drive the wheels. The regenerative braking system in EVs captures kinetic energy during braking and converts it back into electrical energy, which recharges the battery, enhancing energy efficiency.

3. Wind Turbines

• Energy Principle: Conversion of kinetic energy to electrical energy.
• Wind turbines convert the kinetic energy of wind into mechanical energy in the rotor and then into electrical energy through a generator. This process demonstrates the transformation of energy from one form to another, harnessing renewable resources.

4. Photosynthesis

• Energy Principle: Conversion of solar energy into chemical energy.
• In photosynthesis, plants convert the radiant energy from the sun into chemical energy stored in glucose molecules. This process is fundamental for life on Earth, as it provides the primary energy source for nearly all ecosystems.

5. Pendulums

• Energy Principle: Periodic conversion between kinetic and potential energy.
• As a pendulum swings, its energy continually shifts between kinetic and potential. At the highest points of the swing, its energy is all potential (highest height), and at the lowest point, it is all kinetic (maximum speed). This scenario is a classic physics problem illustrating the conservation of mechanical energy in a closed system.

6. Thermal Power Plants

• Energy Principle: Conversion of thermal energy to mechanical energy and then to electrical energy.
• Coal, gas, or nuclear power plants convert the chemical energy of fuel or nuclear energy into thermal energy (heat), which then heats water to create steam. The steam drives turbines, converting thermal energy into mechanical energy, which is finally converted into electrical energy by generators.

7. Hydroelectric Dams

• Energy Principle: Potential energy to kinetic energy to electrical energy.
• Water stored in a reservoir behind a dam possesses potential energy due to its elevation. As water flows down through turbines, this potential energy is converted to kinetic energy and then to electrical energy via the turbines and generators.

8. Bouncing Balls

• Energy Principle: Conversion between kinetic energy and elastic potential energy.
• When a ball is dropped, its gravitational potential energy is converted into kinetic energy as it falls. Upon hitting the ground, the kinetic energy is briefly stored as elastic potential energy as the ball deforms, then reconverted to kinetic energy as it rebounds.

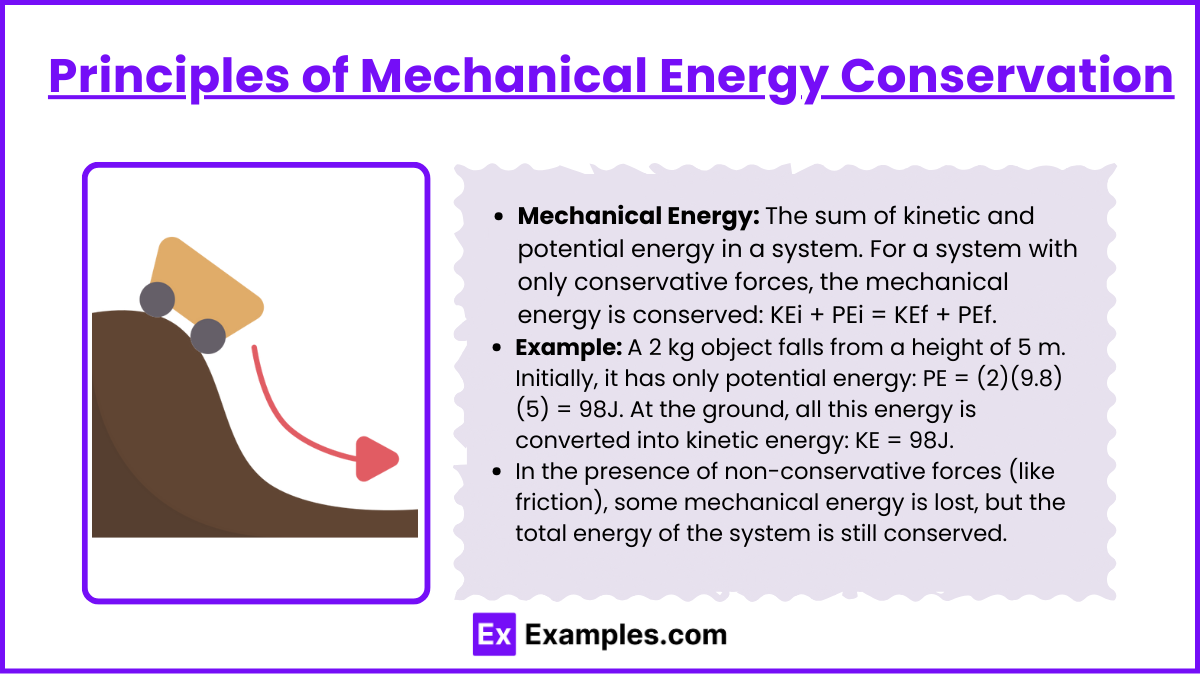
Principles of Mechanical Energy Conservation

• Mechanical Energy: The sum of kinetic and potential energy in a system. For a system with only conservative forces, the mechanical energy is conserved:
  KE_i + PE_i = KE_f​ + PE_f.
• Example: A 2 kg object falls from a height of 5 m. Initially, it has only potential energy: PE = (2)(9.8)(5) = 98J. At the ground, all this energy is converted into kinetic energy: KE = 98J.
• In the presence of non-conservative forces (like friction), some mechanical energy is lost, but the total energy of the system is still conserved.

Examples

Example 1: Lifting a Weight

When you lift a box from the ground to a shelf, you perform work against the force of gravity. The energy you use in this process is transferred to the box as gravitational potential energy. The higher you lift the box, the more potential energy it gains, demonstrating the relationship between work done and energy transformation.

Example 2: Kinetic Energy of a Moving Car

A car traveling at a certain speed possesses kinetic energy, which depends on its mass and velocity. If the driver accelerates the car, the work done by the engine increases the car’s kinetic energy, allowing it to move faster. This example illustrates how energy is transferred and transformed through work to change the speed of an object.

Example 3: Roller Coaster

As a roller coaster climbs to the top of a hill, it gains gravitational potential energy. At the peak, this energy is at its maximum. When the coaster descends, the potential energy converts into kinetic energy, causing the coaster to speed up. This cycle of energy transformation between potential and kinetic energy is a classic example of work and energy in action.

Example 4: Stretching a Spring

When you stretch a spring, work is done on the spring, which stores energy as elastic potential energy. The further you stretch the spring, the more potential energy it stores. Once released, this stored energy converts back into kinetic energy, propelling whatever is attached to the spring forward. This example highlights how work leads to energy storage and subsequent release.

Example 4: Heating Water

When you heat water in a kettle, electrical energy is converted into thermal energy. The work done by the heating element increases the internal energy of the water, raising its temperature. This process showcases the concept of energy transformation, where energy is transferred from one form (electricity) to another (heat) to accomplish a task.

Practice Questions

Question 1

When you lift a heavy object, what happens to the work you do on that object?

A) The work is lost as heat.
B) The object gains kinetic energy only.
C) The object gains potential energy.
D) No energy is transferred to the object.

Correct Answer: C) The object gains potential energy.

Explanation: When you lift a heavy object, you are doing work against the force of gravity. This work results in the object gaining gravitational potential energy, which is energy stored due to its position relative to the ground. As the object is lifted higher, its potential energy increases. Thus, option C correctly describes the outcome of the work done during the lifting process.

Question 2

A car traveling at a constant speed on a flat road has a certain amount of kinetic energy. If the car encounters a hill and begins to climb, what happens to its kinetic energy?

A) It remains the same.
B) It decreases as potential energy increases.
C) It increases as potential energy increases.
D) It is converted entirely into heat energy.

Correct Answer: B) It decreases as potential energy increases.

Explanation: As the car climbs the hill, it converts some of its kinetic energy into gravitational potential energy. The work done against gravity results in a decrease in the car’s speed (if the engine does not provide additional force) and thus a decrease in kinetic energy. At the same time, the car gains potential energy due to its elevation. Therefore, option B accurately describes the relationship between kinetic energy and potential energy during this process.

Question 3

Which of the following scenarios best illustrates the principle of conservation of energy?

A) A ball thrown into the air rises, stops momentarily at the peak, and then falls back down.
B) A car consumes gasoline and converts it into heat energy.
C) A person running uses energy from food to maintain speed.
D) A candle burns and produces light without any energy loss.

Correct Answer: A) A ball thrown into the air rises, stops momentarily at the peak, and then falls back down.

Explanation: The principle of conservation of energy states that energy cannot be created or destroyed; it can only change forms. In the scenario of the ball being thrown, as it rises, its kinetic energy is converted into potential energy. At the peak of its flight, all the kinetic energy has been converted into potential energy. As it falls back down, that potential energy is converted back into kinetic energy. This continuous transformation between kinetic and potential energy illustrates conservation of energy effectively, making option A the correct choice.