Angle

What is Angle?

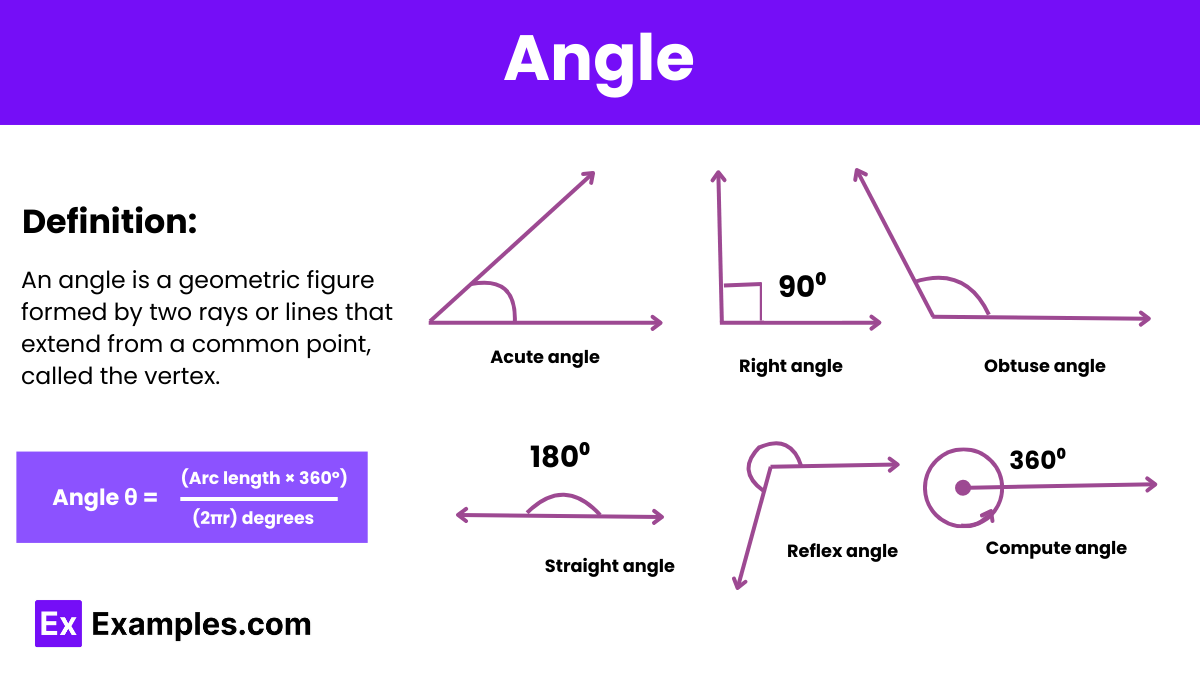
an Angle is a measure of the rotation or separation between two intersecting lines or surfaces at their point of intersection. It quantifies the degree of rotation necessary to align one line or surface with another and is typically measured in degrees or radians. Angles are fundamental in describing rotational motions, orientations, and various geometrical configurations.

Additional Formula

Angular Displacement in Circular Motion

Angular displacement θ (in radians) can be calculated if you know the arc length s and the radius r of the circle

Snell’s Law (Refraction)

When light passes from one medium to another, the angle of incidence θᵢ and the angle of refraction θᵣ are related by

Where n₁ and n₂ are the refractive indices of the two media.

Law of Reflection

The angle of incidence θᵢ is equal to the angle of reflection θᵣ

Using Torque in Rotational Dynamics

Torque (τ) is related to the force applied (F), the distance from the point of rotation (r), and the angle θ between the force vector and the lever arm vector

Lorentz Force Law (Electromagnetism)

The force F on a charged particle moving in a magnetic field is perpendicular to the direction of the velocity v and the magnetic field B, and can be calculated as

Where q is the charge of the particle, v is the velocity, B is the magnetic field strength, and
θ is the angle between v and B.

Applications of Angle

1. Engineering and Architecture:
   • Structural Design: Angles are crucial in determining the stability and balance of structures. Engineers use angles to design beams, trusses, and other structural components.
   • Machine Parts: Gears, levers, and other mechanical parts function based on angular relationships that determine how they interact and transmit forces.
2. Art and Design:
   • Perspective Drawing: Artists use angles to create depth and perspective in their drawings, helping to simulate three-dimensional space on a two-dimensional canvas.
   • Computer Graphics: Angles are used in computer algorithms to render scenes, especially in video games and simulations, where the perspective and shading are angle-dependent.
3. Navigation and Geography:
   • GPS Technology: Angles are used in the triangulation methods that GPS systems employ to determine a user’s exact location on Earth.
   • Astronomy and Astrophysics: Angles help astronomers measure the positions and movements of celestial bodies. Angles also determine the phases of moons, eclipses, and other celestial events.
4. Physics and Mathematics:
   • Circular Motion: In physics, angles are used to describe the position and angular velocity of objects in circular motion, such as satellites orbiting the Earth.
   • Optics: Snell’s Law uses angles to describe how light bends when passing through different media, which is essential for lenses and optical devices.
   • Electromagnetism: The Lorentz force law involves angles to calculate forces on charged particles in magnetic fields.
5. Sports:
   • Ballistics and Projectile Motion: In sports like basketball, golf, and soccer, the angle of launch is critical for achieving desired distances and trajectories.
   • Performance Analysis: Coaches and athletes use angles to enhance performance and technique, especially in sports involving throwing, kicking, or swinging.
6. Medicine:
   • Radiology and Imaging: Angles are used in X-ray imaging and ultrasound to optimize the view of organs and internal structures.
   • Physical Therapy and Biomechanics: Understanding the angles of joints and limbs is crucial for diagnosing issues and planning treatments in physical therapy.
7. Technology and Robotics:
   • Robot Arm Manipulation: Angles determine the positioning and movement of robot arms, which are essential in manufacturing and surgical robots.
   • Drones and Autonomous Vehicles: Angles help in navigating and stabilizing drones and autonomous vehicles, ensuring they operate safely and efficiently.

SI multiples of Angle

Uses of Angle

1. Mathematics: Angles are central to the study of geometry, trigonometry, and calculus. They help in understanding the properties of shapes and solving problems involving distances and rates of change.
2. Engineering: Angles are crucial in civil, mechanical, and aerospace engineering for designing structures, machinery, and aircraft. Engineers use angles to calculate forces, stresses, and optimize designs.
3. Architecture: Architects use angles to design buildings and other structures, ensuring they are not only aesthetically pleasing but also structurally sound. Angles influence the balance, light distribution, and overall functionality of architectural designs.
4. Art and Design: Angles are used in visual art to create perspectives, dynamic compositions, and to guide the viewer’s eye throughout the artwork. In graphic design, angles can affect the layout and usability of visual elements.
5. Navigation: Angles are integral to navigation, particularly in sailing and aviation where they help in plotting courses and in the use of instruments like compasses and sextants.
6. Sports: In sports, understanding angles can improve performance in activities like golf, soccer, basketball, and billiards, where the angle of the shot can determine its success.
7. Technology: Angles are used in technology for things like developing computer graphics, animation, and in the algorithms that guide the functioning of various soft wares and applications.
8. Astronomy: Angles are essential in astronomy for measuring positions and movements of celestial bodies. They help astronomers plot the trajectories of planets and stars, and understand the structure of the universe.

FAQ’S

What is zero angle?

A zero angle occurs when the two arms of the angle overlap completely, essentially creating a straight line with no visible separation between the arms. It measures 0 degrees and is often used as a starting point in angle measurement.

What is 180 degree angle called?

A 180-degree angle is commonly referred to as a straight angle. It looks like a straight line and represents a half rotation from one point back to itself, dividing the plane into two equal parts.

What degree is 3 o clock?

When considering a clock face as a circle divided into 360 degrees, each hour represents 30 degrees of rotation from 12 o’clock. Therefore, 3 o’clock corresponds to 90 degrees from the top of the clock (12 o’clock).

What is angle in trigonometry?

In trigonometry, an angle is typically defined in terms of rotation from a standard initial side (usually the positive x-axis) within a Cartesian coordinate system. It is used to explore the relationships between the angles and sides of triangles. Trigonometric functions like sine, cosine, and tangent are based on angles and are fundamental in calculating various properties and solving problems involving triangles and other geometric figures.