What does Avogadro’s Hypothesis state about gases of equal volume at the same temperature and pressure?
They contain different numbers of molecules
They contain the same number of molecules
They have the same mass
They have different densities

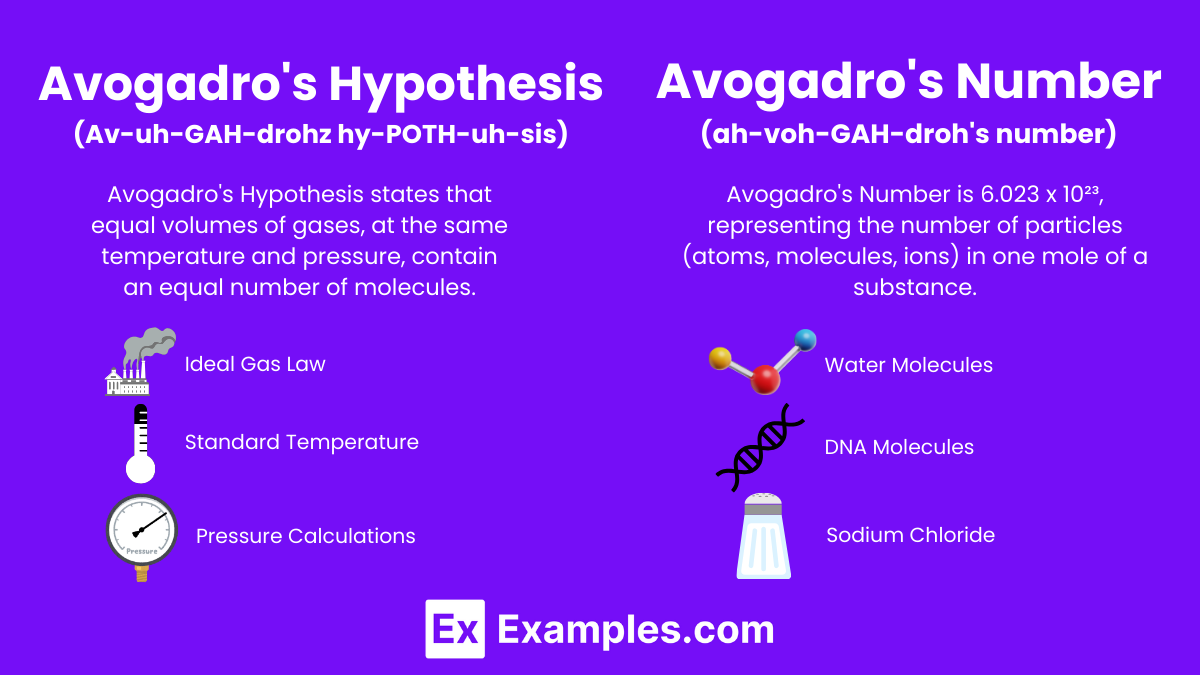
Avogadro’s Hypothesis and Number play a crucial role in both chemistry and physics by providing a fundamental understanding of how gases behave under various conditions. Avogadro’s Hypothesis states that equal volumes of gases, at the same temperature and pressure, contain an equal number of molecules. This principle laid the groundwork for Avogadro’s Number, which defines the number of particles in one mole of a substance as 6.022×10²³. This concept bridges the gap between the microscopic world of atoms and molecules and the macroscopic world we observe, helping scientists apply the laws of physics to chemical reactions and processes.
The mathematical expression of Avogadro’s Hypothesis is:
Where:
Avogadro’s Law can also be written as:
Where:
he formula involving Avogadro’s Number relates the number of particles (N) to the amount of substance in moles (n). It is expressed as
This formula allows scientists to convert between the macroscopic scale of moles and the microscopic scale of individual particles, providing a fundamental tool in chemistry for quantifying substances.
At constant pressure and temperature, Avogadro’s hypothesis can be expressed via the following formula:
𝑉 ∝ 𝑛
𝑉/𝑛=𝑘
Where 𝑉 is the volume of the gas, 𝑛 denotes the amount of gaseous substance (often expressed in moles), and 𝑘 is a constant. When the amount of gaseous substance is increased, the corresponding increase in the volume occupied by the gas can be calculated with the help of the following formula:
𝑉₁/𝑛₁ = 𝑉₂/𝑛₂ (=𝑘, as per Avogadro’s hypothesis)
The graphical representation of Avogadro’s hypothesis (with the amount of substance on the X-axis and volume on the Y-axis) is illustrated below:
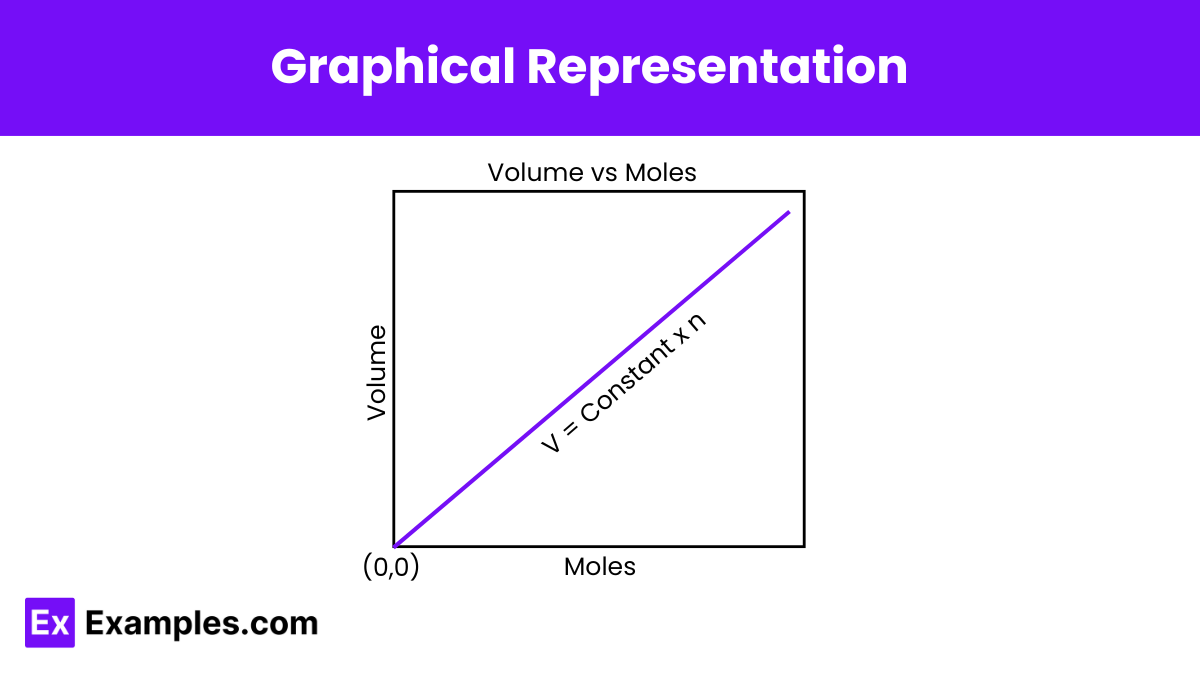
Here, the straight line (which indicates that the two quantities are directly proportional) passes through the origin, implying that zero moles of gas will occupy zero volume.
The Ideal Gas Law is:
𝑃𝑉 = 𝑛𝑅𝑇
Where:
To explore the relationship between volume (𝑉) and the number of moles (𝑛), we rearrange the Ideal Gas Law:
𝑉=𝑛𝑅𝑇/𝑃
Assume that the temperature (𝑇) and pressure (𝑃) are constant. Under these conditions, the equation simplifies to:
𝑉 ∝ 𝑛
This means that the volume (𝑉) is directly proportional to the number of moles (𝑛) of the gas when temperature and pressure are constant.
Since 𝑉 ∝ 𝑛, if you have equal volumes of two different gases at the same temperature and pressure, they must contain the same number of moles of gas.
One mole of any gas contains Avogadro’s number (𝑁ₐ) of molecules, where:
𝑁𝐴 ≈ 6.022×10²³ molecules/mol
Therefore, if equal volumes of different gases contain the same number of moles under the same conditions of temperature and pressure, they also contain the same number of molecules.
Avogadro’s Number was determined through various experimental methods and theoretical calculations, providing a way to quantify the number of particles in a mole. Here is a simplified explanation of the process:
By combining these experimental and theoretical approaches, scientists established Avogadro’s Number as 6.022×10²³, providing a fundamental constant that bridges the microscopic world of atoms and molecules with the macroscopic world of grams and liters.
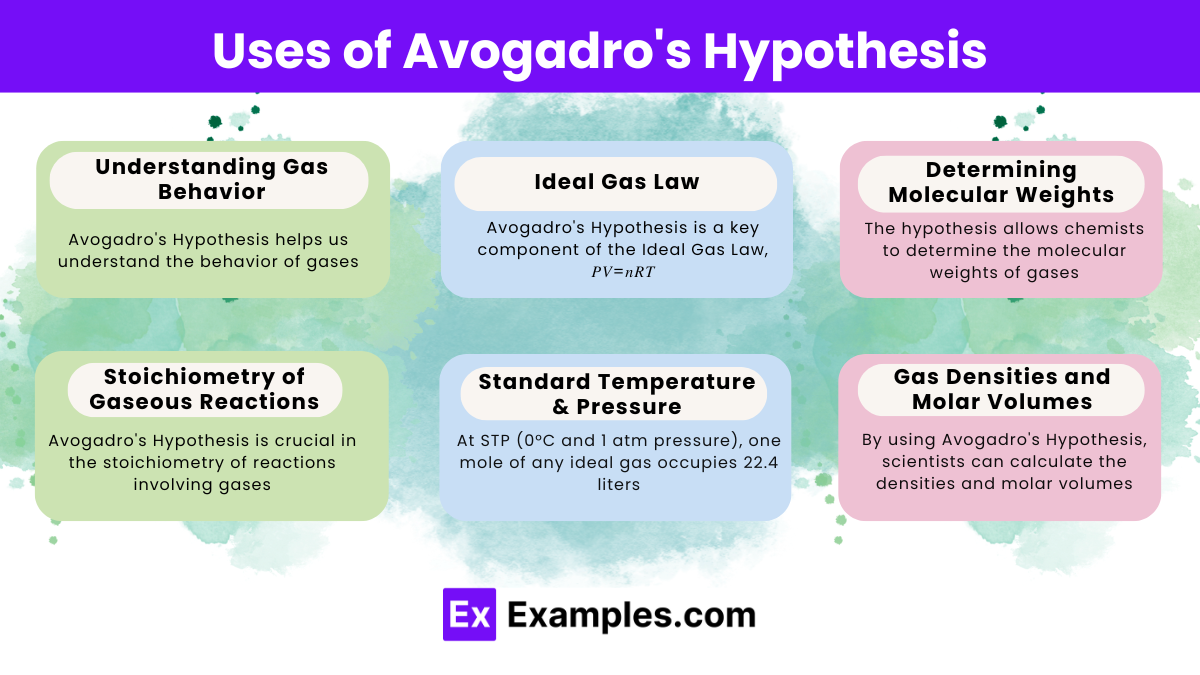

Amedeo Avogadro, an Italian scientist, proposed Avogadro’s Hypothesis in 1811.
Avogadro’s Hypothesis helps understand the behavior of gases and forms the basis for the Ideal Gas Law.
Avogadro’s Hypothesis is a key component, stating that one mole of any gas occupies the same volume at a given temperature and pressure.
Chemists use it to determine molecular weights and to balance chemical equations involving gases.
It allows for predicting the volumes of reactants and products in gas reactions, essential for stoichiometric calculations.
One mole of an ideal gas occupies 22.4 liters at standard temperature and pressure (STP).
Yes, it helps calculate the density of gases by relating volume and number of moles.
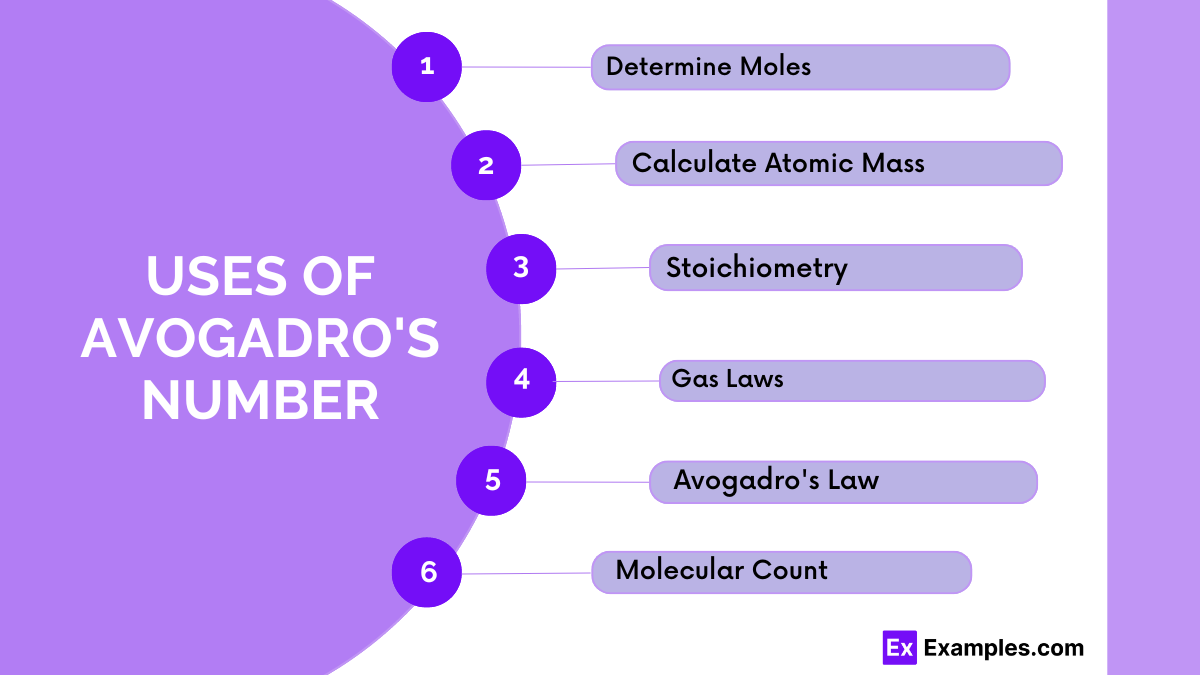
You multiply by Avogadro’s Number to find the number of particles and divide by it to find the number of moles from particles.
Avogadro didn’t calculate it himself; later scientists derived it through experiments like electrolysis and Brownian motion analysis, relating atomic masses to macroscopic quantities.
A mole is 6.022 × 10²³ because it represents the number of atoms in 12 grams of carbon-12, a standard for measuring substances.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What does Avogadro’s Hypothesis state about gases of equal volume at the same temperature and pressure?
They contain different numbers of molecules
They contain the same number of molecules
They have the same mass
They have different densities
What is Avogadro’s number used for in chemistry?
Calculating the number of moles in a substance
Determining the volume of a gas
Converting between mass and volume
Finding the molecular weight of a compound
Avogadro’s Hypothesis implies that which of the following is true about gases?
The density of different gases is the same
The volume of different gases is directly proportional to their number of moles
The temperature affects the number of molecules in a gas
The pressure of a gas affects its number of molecules
Which of the following is NOT a consequence of Avogadro’s Hypothesis?
Equal volumes of gases at the same conditions have the same number of molecules
The mass of gases can be compared directly
Moles of gas can be used to calculate the number of molecules
Gases of the same volume at the same temperature and pressure have different densities
How is Avogadro’s number used in stoichiometry?
To find the mass of elements in a compound
To convert between grams and moles
To determine the number of molecules in a given number of moles
To calculate the volume of gases
In what type of reaction is Avogadro’s number particularly useful?
Chemical equilibrium reactions
Redox reactions
Gas reactions
Acid-base reactions
How does Avogadro’s Hypothesis support the concept of mole in chemistry?
By providing a direct relation between mass and volume
By defining the number of particles in a mole
By explaining gas density variations
By determining gas solubility in liquids
If 1 mole of gas occupies 22.4 liters at standard temperature and pressure (STP), what can be inferred about Avogadro’s number?
It specifies the pressure of the gas
It determines the volume of the gas
It defines the temperature of the gas
It relates the number of particles to volume
Which principle does Avogadro’s Hypothesis support about equal volumes of gases?
They have different chemical properties
They have different molecular weights
They have the same number of molecules
They have different atomic weights
What is the primary application of Avogadro’s number in chemistry?
To calculate the density of gases
To determine the rate of reaction
To convert between macroscopic quantities and microscopic particles
To measure the volume of solids
Before you leave, take our quick quiz to enhance your learning!

