Boltzmann Distribution – Examples, Definition, Formula, FAQ’S

The Boltzmann Distribution is a fundamental concept in statistical mechanics, providing a powerful means to describe how particles, such as atoms or molecules, are distributed among various energy states in thermal equilibrium. This distribution is crucial for understanding a wide range of physical phenomena, including the behavior of gases and the principles underlying the Laws of Fluid Dynamics.

What is Boltzmann Distribution?

Boltzmann Distribution Formula

The Boltzmann Distribution Formula is a simple yet powerful expression. It quantifies the probability of particles occupying different energy states in thermal equilibrium. It is represented as:

Here, P(E) denotes the probability of finding a particle in an energy state E, k is the Boltzmann constant, and T represents the absolute temperature. This formula shows that the likelihood of a particle being in a certain energy state. It decreases exponentially with increasing energy and is more favorable at higher temperatures.

Boltzmann Distribution Derivation

The Boltzmann Distribution can be understood and derived through a straightforward conceptual approach, focusing on the behavior of particles in a system at thermal equilibrium without delving into complex equations.

Step 1: Understand Energy States

Consider a system where particles, such as atoms or molecules, can exist in various energy states. These energy states could range from low to high energy levels.

Step 2: Thermal Equilibrium

When the system reaches thermal equilibrium, it means the temperature is uniform throughout, and the energy distribution among the particles is stable over time. At this point, no net energy transfer occurs between different parts of the system.

Step 3: Probability and Energy

In a naturally occurring scenario, particles tend to favor lower energy states because these are more stable and less energy-intensive to maintain. However, with an increase in temperature, particles gain more thermal energy, which allows them to reach and maintain higher energy states.

Step 4: Exponential Decrease

The probability of finding a particle in a higher energy state decreases exponentially as the energy increases. This is because it’s statistically less likely for particles to achieve and sustain higher energy levels without additional energy input (like an increase in temperature).

Step 5: Equilibrium Distribution

At equilibrium, the distribution of particles among the energy states follows a pattern where most particles will be found in the lower energy states, and fewer will populate the higher states, creating a balance dictated by the temperature of the system.

Uses of Boltzmann Distribution

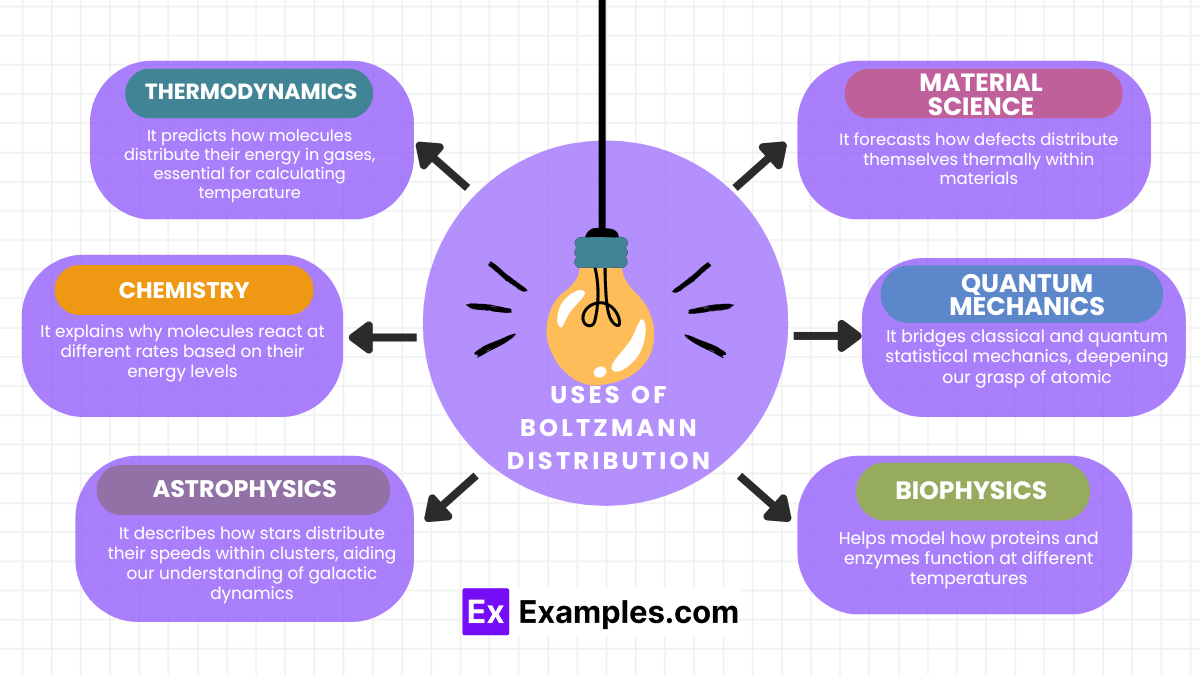
The Boltzmann Distribution plays a pivotal role across various scientific disciplines. Here’s how it actively contributes:

• Thermodynamics: It predicts how molecules distribute their energy in gases, essential for calculating temperature and pressure.
• Chemistry: It explains why molecules react at different rates based on their energy levels, thus influencing reaction dynamics.
• Astrophysics: It describes how stars distribute their speeds within clusters, aiding our understanding of galactic dynamics.
• Material Science: It forecasts how defects distribute themselves thermally within materials, crucial for designing materials that withstand high temperatures.
• Quantum Mechanics: It bridges classical and quantum statistical mechanics, deepening our grasp of atomic and subatomic particles’ behavior.
• Biophysics: Helps model how proteins and enzymes function at different temperatures.

Examples for Boltzmann Distribution

The Boltzmann Distribution provides critical insights across a spectrum of scientific fields. Here are some illustrative examples, enhanced with transition words for clearer understanding:

• Gas Particle Speeds in a Room: Initially, it determines the speed distribution of air molecules in a room at a given temperature, explaining why some molecules move faster than others.
• Population of Atomic Energy Levels: Additionally, it predicts how electrons populate different energy levels in an atom, which is crucial for understanding atomic emission and absorption spectra.
• Thermal Distribution in Stars: Furthermore, it explains the energy distribution among the particles in a star, aiding astrophysicists in their studies of stellar composition and lifecycle.
• Enzyme Reaction Rates: Moreover, it describes how temperature affects the rates at which enzymes catalyze reactions, highlighting the energy dependency of biochemical processes.
• Semiconductor Electron Behavior: Lastly, it models how electrons and holes distribute themselves in semiconductors, key for designing electronic devices.

FAQ’S

Why is it called Boltzmann constant?

Named after Ludwig Boltzmann, it quantifies the relationship between kinetic energy and temperature in gases, reflecting his contributions to statistical mechanics.

What is the difference between Gibbs and Boltzmann distribution?

Gibbs distribution applies to systems with varying particle numbers and energy. Whereas Boltzmann distribution specifically addresses systems at constant temperature and particle count.

What affects Maxwell Boltzmann distribution?

Temperature significantly influences the Maxwell-Boltzmann distribution, as higher temperatures increase the kinetic energy, thereby broadening the distribution of particle speeds.