Buoyancy Formula – Formula, Applications, Limitations, Example Problems

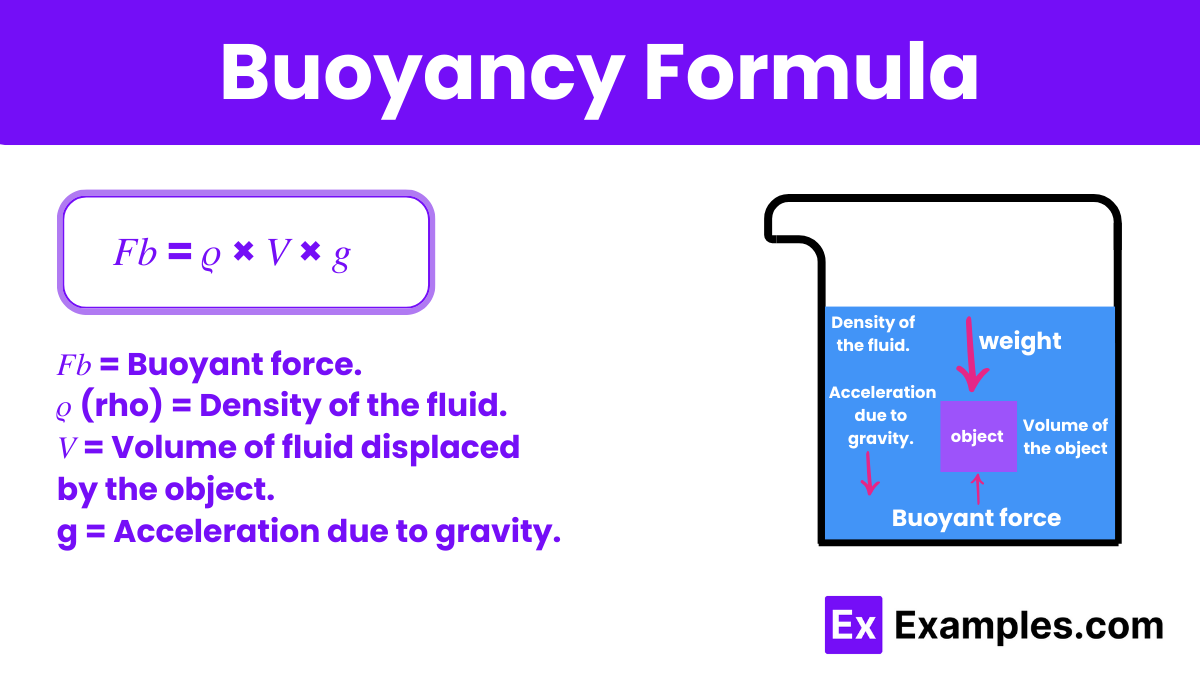
Buoyancy, a fundamental concept in physics, refers to the upward force that a fluid exerts on an object that is partially or wholly immersed in it. This force is crucial for understanding why objects float or sink in water or any other fluid. The formula to calculate buoyancy, also known as the buoyant force, is straightforward and embodies the principle that the buoyant force is equal to the weight of the fluid that the object displaces. Mathematically, it is represented as

• 𝐹𝑏​ is the buoyant force.
• 𝜌 (rho) is the density of the fluid.
• 𝑉 is the volume of fluid displaced by the object.
• g is the acceleration due to gravity.

The concept and the accompanying formula were first rigorously documented by the ancient Greek scientist Archimedes, making it one of the earliest documented principles in classical physics. His discovery not only laid the groundwork for the study of fluid dynamics but also has practical applications in designing ships and submarines, understanding the behavior of floating and submerged objects, and in various engineering tasks involving fluids.

Applications of Buoyancy Formula

• Ship Design: Naval architects apply the Buoyancy formula to determine how much weight a ship can carry without sinking, Optimizing its design for safety and efficiency.
• Submarines: Engineers use buoyancy calculations to control a Submarine’s dive and ascent, ensuring it can safely navigate underwater by adjusting its buoyant force.
• Hydrology: Hydrologists employ the buoyancy principle to predict water levels and the buoyancy effects on submerged structures during floods.
• Aerospace Engineering: Aerospace engineers design spacecraft components, like fuel tanks, to withstand the buoyant forces experienced during launches and while in fluid atmospheres of other planets.
• Recreational Equipment: The buoyancy formula is essential for designing water sport equipment, such as life vests and floatation devices, ensuring they provide adequate buoyancy to keep users afloat.

Limitations of Buoyancy Formula

• Ship Design: Naval architects apply the Buoyancy formula to determine how much weight a ship can carry without sinking, optimizing its design for safety and efficiency.
• Submarines: Engineers use buoyancy calculations to control a submarine’s dive and ascent, ensuring it can safely navigate underwater by adjusting its buoyant force.
• Hydrology: Hydrologists employ the buoyancy principle to predict water levels and the buoyancy effects on submerged structures during floods.
• Aerospace Engineering: Aerospace engineers design spacecraft components, like fuel tanks, to withstand the buoyant forces experienced during launches and while in fluid atmospheres of other planets.
• Recreational Equipment: The Buoyancy formula is essential for designing water sport equipment, such as life vests and Floatation devices, Ensuring they provide adequate buoyancy to keep users a float.

Example Problems on Buoyancy Formula

Example 1: Basic Buoyancy Calculation

Problem: A wooden block with a volume of 0.05 m³ is submerged in water. Given that the density of water is 1000 kg/m³, calculate the buoyant force acting on the block.

Solution:

1. Identify the known values:
   • Volume of the block, 𝑉 = 0.05 m³
   • Density of water, 𝜌 = 1000 kg / m³
   • Acceleration due to gravity, 𝑔 = 9.8 m/s²
2. Apply the buoyancy formula: 𝐹𝑏 = 𝜌 × 𝑉 × 𝑔
3. Calculate:
   • 𝐹𝑏 =1000 × 0.05 × 9.8=490 Newtons
4. Conclusion: The buoyant force on the block is 490 Newtons.

Example 2: Determining Submerged Volume

Problem: A metal cylinder weighs 800 N and displaces water weighing 600 N when completely submerged. Calculate the volume of the cylinder using the buoyancy formula.

Solution:

1. Understand that the buoyant force equals the weight of displaced water, 𝐹𝑏 = 600​ N.
2. Known values:
   • Weight of the cylinder, 𝑊 = 800 N
   • Density of water, 𝜌 = 1000 kg/m³
   • Acceleration due to gravity, 𝑔= 9.8 m/s²
3. Rearrange the buoyancy formula to find the volume, 𝑉=𝐹𝑏 / 𝜌 × 𝑔​​
4. Calculate:
   • 𝑉 = 600 / (1000 × 9.8)≈0.0612 m³
5. Conclusion: The volume of the cylinder is approximately 0.0612 m³.

Example 3: Floatation Problem

Problem: A lifebuoy has a density of 200 kg/m³ and a volume of 0.15 m³. Determine if it will float in seawater with a density of 1025 kg/m³.

Solution:

1. Use the buoyancy formula to calculate the buoyant force:
   • 𝐹𝑏 = 1025 × 0.15 × 9.8 = 1508.25 Newtons
2. Calculate the weight of the lifebuoy:
   • Weight 𝑊 = 200 × 0.15 × 9.8 = 294 Newtons
3. Compare buoyant force and weight:
   • 𝐹𝑏 > 𝑊
4. Conclusion: Since the buoyant force is greater than the weight, the lifebuoy will float.

Example 4: Buoyancy in Different Fluids

Problem: A cube with a side of 0.1 m and a density of 800 kg/m³ is submerged in glycerin (density = 1260 kg/m³). Calculate the buoyant force.

Solution:

1. Calculate the volume of the cube:
   • 𝑉=0.1×0.1×0.1=0.001 m³
2. Use the buoyancy formula:
   • 𝐹𝑏 = 1260 × 0.001 × 9.8 = 12.348 Newtons
3. Conclusion: The buoyant force acting on the cube in glycerin is approximately 12.348 Newtons.

FAQs

How Do You Calculate Buoyancy?

To calculate buoyancy, use the formula 𝐹𝑏=𝜌×𝑉×𝑔, where 𝜌 is fluid density, V is displaced volume, and g is gravity.

What is P in Buoyancy Formula?

In the buoyancy formula, “P” typically does not appear; the primary variables are ρ (density), V (volume), and g (gravity).

What is the Basic Rule of Buoyancy?

The basic rule of buoyancy states that an object will float if it displaces a weight of fluid equal to or greater than its own weight.