Constants

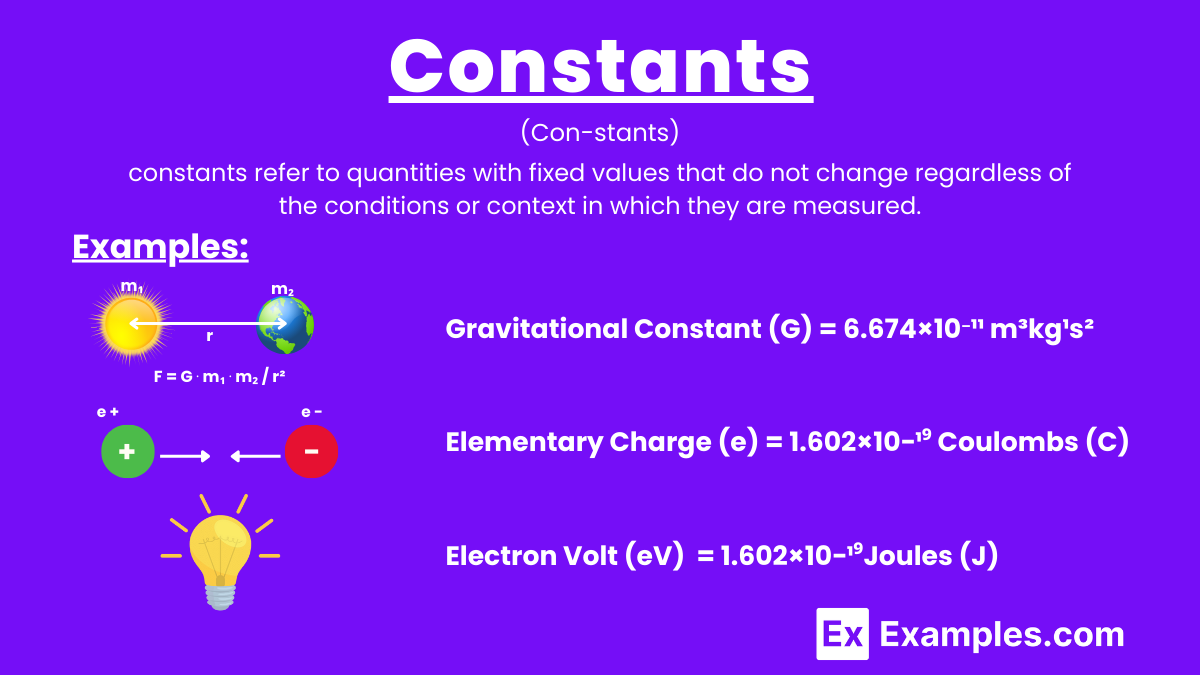
A constant in physics is a quantity with a fixed value that does not change regardless of the conditions or the variables in a given situation. Constants serve as fundamental benchmarks in various equations and theories, providing consistency and predictability in scientific calculations. Examples include the speed of light in a vacuum (c), Planck’s constant (h), and the gravitational constant (G). These constants are crucial for formulating physical laws and principles, enabling scientists to describe and understand the natural world accurately.

What are Constants?

In physics, constants refer to quantities with fixed values that do not change regardless of the conditions or context in which they are measured. These values remain unchanged over time and space, serving as fundamental reference points in scientific calculations and theories.

List of Constants in Physics

Constant	Symbol	Value	Units
Speed of Light	c	2.998×10⁸	meters per second (m/s)
Gravitational Constant	G	6.674×10⁻¹¹	m³kg¹s²
Planck’s Constant	h	6.626×10−34	Joule seconds (Js)
Reduced Planck’s Constant	ℏ	1.055×10−34	Joule seconds (Js)
Elementary Charge	e	1.602×10−19	Coulombs (C)
Avogadro’s Number	NA	6.022×1023	mol−1
Boltzmann Constant	kB	1.381×10−23	Joules per kelvin (J/K)
Gas Constant	R	8.314	J ⋅ mol−1 ⋅ K−1
Permittivity of Free Space	ε0	8.854×10−12	F⋅m−1
Permeability of Free Space	μ0	4π×10−7	N⋅A−2
Electron Mass	me	9.109×10−31	Kilograms (kg)
Proton Mass	mp	1.673×10−27	Kilograms (kg)
Neutron Mass	mn	1.675×10−27	Kilograms (kg)
Fine-Structure Constant	α	7.297×10−3	Dimensionless
Stefan-Boltzmann Constant	σ	5.670×10−8	W⋅m−2⋅K−4
Rydberg Constant	R∞	1.097×107	m−1
Faraday Constant	F	9.649×104	Coulombs per mole (C/mol)
Atomic Mass Unit	u	1.661×10−27	Kilograms (kg)
Bohr Radius	a0	5.292×10−11	Meters (m)
Coulomb’s Constant	ke	8.988×109	N⋅m2⋅C−2
Universal Gas Constant	R	8.314	J⋅mol−1⋅K−1
Planck Length	ℓP	1.616×10−35	Meters (m)
Planck Time	tP	5.391×10−44	Seconds (s)
Planck Mass	mP​	2.176×10−8	Kilograms (kg)
Planck Temperature	TP	1.416×1032	Kelvin (K)
Wien’s Displacement Constant	b	2.898×10−3	m⋅K
Bohr Magneton	μB	9.274×10−24	Joules per Tesla (J/T)
Nuclear Magneton	μN	5.051×10−27	Joules per Tesla (J/T)
Magnetic Flux Quantum	Φ0	2.068×10−15	Weber (Wb)
Conductance Quantum	G0	7.748×10−5	Siemens (S)
Von Klitzing Constant	RK	2.581×104	Ohms (Ω)
Josephson Constant	KJ	4.835×1014	Hz⋅V−1
Compton Wavelength	λC	2.426×10−12	Meters (m)
Electron Volt	eV	1.602×10−19	Joules (J)
Hartree Energy	Eh	4.360×10−18	Joules (J)
Atomic Unit of Charge	e	1.602×10−19	Coulombs (C)
Atomic Unit of Mass	me	9.109×10−31	Kilograms (kg)
Atomic Unit of Length	a0	5.292×10−11	Meters (m)
Atomic Unit of Time	t0	2.418×10−17	Seconds (s)
Fermi Coupling Constant	GF	1.166×10−5	GeV−2
Weak Mixing Angle	sin⁡2θW	0.2229	Dimensionless
Solar Mass	M⊙	1.989×1030	Kilograms (kg)
Astronomical Unit	AU	1.496×1011	Meters (m)
Light Year	ly	9.461×1015	Meters (m)
Parsec	pc	3.086×1016	Meters (m)
Hubble Constant	H0	67.4	km⋅s−1⋅Mpc−1
Chandrasekhar Limit	MCh​	1.4	Solar Masses (M_\odot)
Electron Magnetic Moment	μe​	−9.284×10−24	Joules per Tesla (J/T)

Speed of Light (c)

The speed of light in a vacuum, denoted as c, is approximately 2.998×10⁸ meters per second. This constant is fundamental in physics because it sets the maximum speed at which all energy, matter, and information in the universe can travel. It plays a crucial role in Einstein’s theory of relativity, which shows how space and time are interwoven.

Example: Light from the Sun takes about 8 minutes and 20 seconds to reach Earth, traveling at this constant speed.

Gravitational Constant (G)

The gravitational constant, G, is 6.674×10⁻¹¹ m³kg¹s². It is a key quantity in Newton’s law of universal gravitation, which describes the gravitational attraction between two masses. This constant helps us understand the strength of the gravitational force in the universe.

Example: The gravitational force between two 1 kg masses separated by 1 meter is 6.674×10⁻¹¹ Newtons.

Planck’s Constant (h)

Planck’s constant, h, is 6.626×10⁻³⁴ Joule seconds. It is a fundamental constant in quantum mechanics, reflecting the quantization of energy levels in atomic and subatomic systems. It is central to the Heisenberg uncertainty principle and the Planck-Einstein relation E=hν, linking energy and frequency.

Example: The energy of a photon with a frequency of 5×10¹⁴ Hz is 3.313×10⁻¹⁹ Joules, calculated using E=hν.

Reduced Planck’s Constant (ℏ)

The reduced Planck’s constant, ℏ, is 1.055×10⁻³⁴ Joule seconds. It is used frequently in quantum mechanics and is equal to Planck’s constant divided by 2π. It is pivotal in defining the scales at which quantum effects become significant.

Example: The angular momentum of an electron in the ground state of a hydrogen atom is ℏ.

Elementary Charge (e)

The elementary charge, e, is 1.602×10⁻¹⁹ Coulombs. This constant represents the electric charge carried by a single proton or the magnitude of the charge of a single electron, crucial for understanding electromagnetic interactions.

Example: The charge of an electron is −1.602×10⁻¹⁹ Coulombs.

Avogadro’s Number (N_A​)

Avogadro’s number, N​_A, is 6.022×10 ²³ per mole. It defines the number of atoms, ions, or molecules in one mole of a substance, forming a bridge between the macroscopic and atomic worlds in chemistry and physics.

Example: One mole of water molecules contains 6.022×10²³ water molecules.

Boltzmann Constant (k_B​)

The Boltzmann constant, k_B​, is 1.381×10⁻²³ Joules per Kelvin. It relates the average kinetic energy of particles in a gas with the temperature of the gas, playing a crucial role in statistical mechanics and thermodynamics.

Example: At room temperature (300 K), the average kinetic energy of a gas molecule is 4.143×10⁻²¹
Joules.

Gas Constant (R)

The gas constant, R, is 8.314 J⋅mol⁻¹⋅K⁻¹. It is the constant of proportionality in the ideal gas law PV=nRT, linking pressure, volume, temperature, and the amount of gas.

Example: The pressure of 1 mole of an ideal gas at 1 liter and 300 K is 24.942 atm.

Permittivity of Free Space (ε₀​)

The permittivity of free space, ε₀​, is 8.854×10⁻¹² F⋅m⁻¹. It is a measure of the ability of the vacuum to permit electric field lines, essential for understanding electrostatics and capacitance.

Example: The capacitance of a parallel-plate capacitor with a vacuum between the plates, an area of 1m², and a separation of 1m is 8.854×10⁻⁹ Farads.

Permeability of Free Space (μ₀​)

The permeability of free space, μ₀​, is 4π×10⁻⁷. This constant measures the ability of the vacuum to support magnetic field lines, important in the study of magnetostatics and electromagnetic theory.

Example: The magnetic field around a long straight wire carrying a current of 1 A is 2×10⁻⁷ Tesla at a distance of 1 meter.

Electron Mass (me​)

The electron mass, me​, is 9.109×10⁻³¹ kilograms. This is the mass of a single electron at rest and is a fundamental constant in both atomic physics and quantum mechanics.

Example: The rest energy of an electron is 8.187×10⁻¹⁴ Joules, calculated using E=mc²

Proton Mass (m_p​)

The proton mass, m_p​, is 1.673×10⁻²⁷ kilograms. It represents the mass of a single proton, fundamental in nuclear physics and chemistry, as protons are a primary constituent of atomic nuclei.

Example: The rest energy of a proton is 1.503×10⁻¹⁰ Joules.

Neutron Mass (m_n​)

The neutron mass, m_n​, is 1.675×10⁻²⁷ kilograms. Neutrons, along with protons, make up the atomic nucleus, and their mass is crucial for calculations involving nuclear reactions and stability.

Fine-Structure Constant (α)

The fine-structure constant, α, is 7.297×10⁻³. This dimensionless constant characterizes the strength of the electromagnetic interaction between elementary charged particles, fundamental in quantum electrodynamics.

Stefan-Boltzmann Constant (σ)

The Stefan-Boltzmann constant, σ is 5.670×10⁻⁸ W⋅m⁻²⋅K⁻⁴. It relates the total energy radiated per unit surface area of a black body to the fourth power of its temperature, crucial in thermodynamics and astrophysics.

Rydberg Constant (R∞)

The Rydberg constant, R∞​, is 1.097×10⁷ m⁻¹. It is used in atomic physics to describe the wavelengths of spectral lines of many chemical elements.

Faraday Constant (F)

The Faraday constant, F, is 9.649×10⁴ Coulombs per mole. It represents the charge of one mole of electrons, linking electrochemistry to the amount of substance.

Atomic Mass Unit (u)

The atomic mass unit, u, is 1.661×10⁻²⁷ kilograms. It is used to express atomic and molecular masses, providing a convenient scale for comparison of different atoms and molecules.

Bohr Radius (a₀​)

The Bohr radius, a₀​, is 5.292×10⁻¹¹ meters. It represents the average distance between the nucleus and the electron in a hydrogen atom in its ground state, fundamental in atomic physics.

Coulomb’s Constant (k_e​)

Coulomb’s constant, k_e​, is 8.988×10⁹ N⋅m²⋅C⁻². It is the proportionality constant in Coulomb’s law, describing the force between two point charges.

Universal Gas Constant (R)

The universal gas constant, R, is 8.3148.3148.314 J⋅mol−1⋅K−1\text{J} \cdot \text{mol}^{-1} \cdot \text{K}^{-1}J⋅mol−1⋅K−1. It appears in the ideal gas law and other equations of state, relating the macroscopic properties of gases.

Planck Length (ℓ_P)

The Planck length, ℓ_P​, is 1.616×10⁻³⁵ meters. It is a fundamental scale in quantum mechanics, representing the smallest meaningful length, where classical ideas about gravity and space-time cease to be valid.

Planck Time (t_P​)

The Planck time, t_P​, is 1.416×10³² seconds. It is the time it takes for light to travel one Planck length, significant in quantum gravity and cosmology.

Planck Mass (m_P)

The Planck mass, m_P​, is 2.176×10⁻⁸ kilograms. It is a fundamental mass scale in quantum mechanics, marking the transition between classical and quantum descriptions of gravity.

Planck Temperature (T_P​)

The Planck temperature, T_P​, is 1.416×10³² Kelvin. It is the highest theoretically possible temperature, beyond which the laws of physics as currently understood cease to be useful.

Wien’s Displacement Constant (b)

Wien’s displacement constant, b, is 2.898×10⁻³ m.k. It relates the temperature of a black body to the wavelength at which it emits radiation most strongly, used in thermal radiation studies.

Bohr Magneton (μ_B​)

The Bohr magneton, μ_B​, is 9.274×10⁻²⁴ Joules per Tesla. It is a physical constant related to the magnetic moment of an electron due to its orbital or spin motion, important in quantum mechanics and magnetism.

Nuclear Magneton (μ_N​)

The nuclear magneton, μ_N​, is 5.051×10⁻²⁷ Joules per Tesla. It is similar to the Bohr magneton but smaller, used to describe the magnetic moment of nucleons and nuclei.

Magnetic Flux Quantum (Φ₀​)

The magnetic flux quantum, Φ_0​, is 2.068×10⁻¹⁵ Weber. It is the quantum of magnetic flux, fundamental in the study of superconductivity and quantum Hall effects.

Conductance Quantum (G₀)

The conductance quantum, G₀​, is 7.748×10⁻⁵ Siemens. It represents the quantized unit of electrical conductance, crucial in the study of quantum transport.

Von Klitzing Constant (R_K​)

The von Klitzing constant, R_K, is 2.581×10⁴ Ohms. It is used in the quantum Hall effect to define the resistance quantum, providing a standard for electrical resistance.

Josephson Constant (K_J​)

The Josephson constant, K_J​, is 4.835×10¹⁴ Hz⋅V⁻¹. It relates the voltage across a Josephson junction to the frequency of the resulting AC current, important in superconductivity.

Compton Wavelength (λ_C​)

The Compton wavelength, λ_C​, is 2.426×10⁻¹² meters. It represents a quantum mechanical limit to the localization of particles, significant in quantum field theory.

Electron Volt (e_V)

An electron volt, e_V, is 1.602×10⁻¹⁹ Joules. It is a unit of energy commonly used in atomic, nuclear, and particle physics, representing the energy gained by an electron when accelerated through a potential difference of one volt.

Hartree Energy (E_h​)

The Hartree energy, E_h​, is 4.360×10⁻¹⁸ Joules. It is a unit of energy used in atomic physics and quantum chemistry, representing the electrostatic potential energy of the hydrogen atom in its ground state.

Atomic Unit of Charge (e)

The atomic unit of charge, e, is 1.602×10⁻¹⁹ Coulombs. It is used as a convenient unit of electric charge in atomic physics and quantum chemistry.

Atomic Unit of Mass (m_e​)

The atomic unit of mass, m_e​, is 9.109×10⁻³¹ kilograms. It provides a convenient scale for comparing the masses of different particles in atomic and molecular systems.

Atomic Unit of Length (a₀​)

The atomic unit of length, a₀​, is 5.292×10⁻¹¹ meters. It is the Bohr radius, representing the typical size of atoms and providing a natural length scale in atomic physics.

Atomic Unit of Time (t₀​)

The atomic unit of time, t₀​, is 2.418×10⁻¹⁷ seconds. It provides a natural time scale in atomic and molecular physics, corresponding to the period of an electron orbiting a hydrogen nucleus.

Fermi Coupling Constant (G_F​)

The Fermi coupling constant, G_F, is 1.166×10⁻⁵ GeV⁻². It characterizes the strength of the weak force, one of the four fundamental forces in the universe, crucial in particle physics.

Weak Mixing Angle (sin⁡²θW​)

The weak mixing angle, sin⁡²θW​, is 0.2229. It quantifies the mixing of the electromagnetic and weak forces in the electroweak interaction, fundamental in the Standard Model of particle physics.

Solar Mass (M_⊙​)

The solar mass, M_⊙​, is 1.989×10³⁰ kilograms. It is the mass of the Sun, used as a standard unit for expressing the masses of other stars and galaxies in astrophysics.

Astronomical Unit (A_U)

The astronomical unit, A_U, is 1.496×10¹¹ meters. It represents the average distance between the Earth and the Sun, providing a useful scale for measuring distances within the solar system.

Light Year (ly)

A light year, ly, is 9.461×10¹⁵ meters. It represents the distance that light travels in a vacuum in one year, used to express astronomical distances.

Parsec (pc)

A parsec, pc, is 3.086×10¹⁶ meters. It is a unit of distance used in astronomy, equal to about 3.26 light years, representing the distance at which one astronomical unit subtends an angle of one arcsecond.

Hubble Constant (H₀​)

The Hubble constant, H₀​, is 67.4 km⋅s⁻¹⋅Mpc⁻¹. It measures the rate of expansion of the universe, fundamental in cosmology.

Chandrasekhar Limit (M_Ch​)

The Chandrasekhar limit, M_Ch​, is 1.4 solar masses. It is the maximum mass of a stable white dwarf star, beyond which it will collapse into a neutron star or black hole, significant in stellar evolution.

Electron Magnetic Moment (μe​)

The electron magnetic moment, μe​, is −9.284×10⁻²⁴ Joules per Tesla. It represents the intrinsic magnetic moment of an electron due to its spin, fundamental in quantum mechanics and magnetism.

FAQ’s