Heat Loss Formula – Formula, Usages, Example Problems

What is Heat Loss Formula?

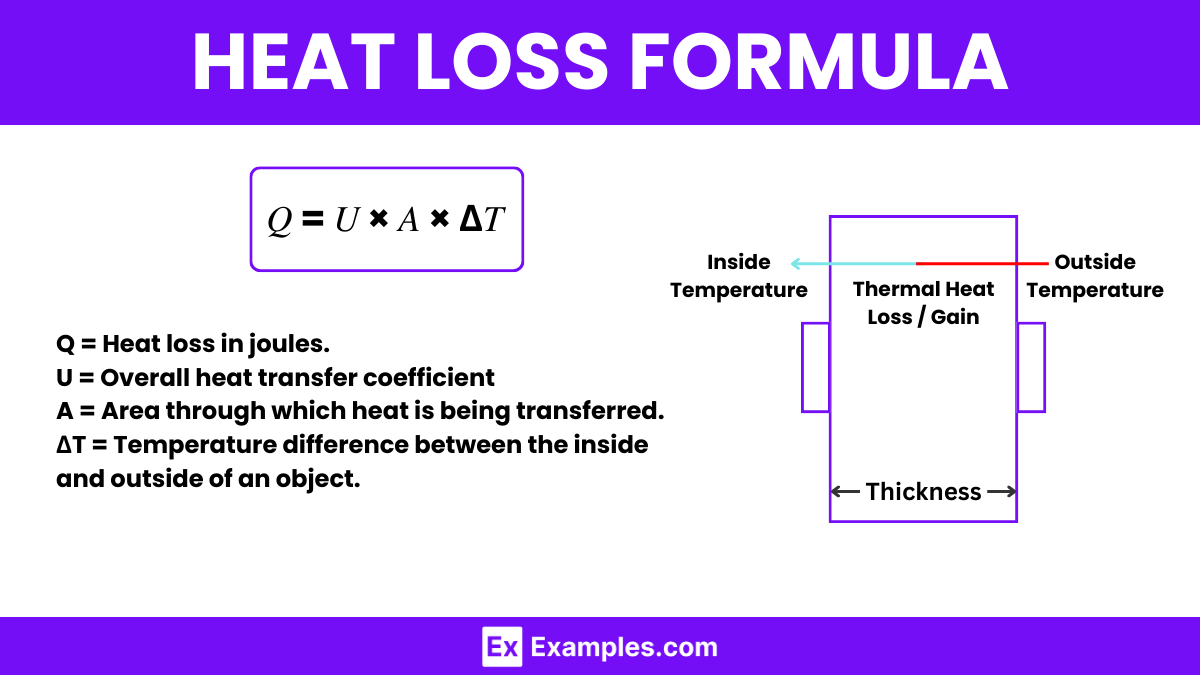
The heat loss formula is crucial in physics for calculating the energy transferred from a warmer object to a cooler environment over a specific time. This formula is particularly useful in fields such as thermodynamics and building engineering, where understanding and managing heat transfer is essential. The general formula for heat loss, discovered by the French physicist Joseph Fourier in the 19th century, is expressed as

• Q represents the heat loss in joules.
• U is the overall heat transfer coefficient in watts per square meter per degree Celsius.
• A is the area through which heat is being transferred in square meters.
• ΔT is the temperature difference between the inside and outside of an object in degrees Celsius.

Fourier’s work laid the foundation for what would later be formalized as Fourier’s Law of Heat Conduction. This principle helps in calculating the rate at which heat escapes through materials, which is vital for designing efficient thermal insulation and for enhancing energy conservation in buildings and industrial applications. The formula not only allows engineers to predict how much heat loss will occur but also to design measures to reduce unnecessary energy expenditures effectively.

Derivation of Heat Loss Formula

Fourier’s Law Expression: The equation based on Fourier’s Law is initially expressed as:

• 𝑄˙​ represents the rate of heat transfer measured in watts (W).
• k stands for the thermal conductivity of the material expressed in watts per meter-kelvin (W/m·K).
• A is the cross-sectional area perpendicular to the direction of heat flow in square meters (m²).
• 𝑑𝑇 is the temperature differential across the material in degrees Celsius (°C).
• dx denotes the thickness of the material in meters (m).

Simplifying Assumptions: For a clearer analysis, it’s assumed that the heat flow is steady-state (constant temperature difference) and one-dimensional. With these assumptions, the equation simplifies to:

• Δ𝑇 is the temperature difference across the material
• 𝐿 is the material’s thickness.

Incorporating Heat Transfer Coefficient: In practical scenarios where materials are often layered, incorporating the concept of thermal resistance and the overall heat transfer coefficient, 𝑈, is beneficial. This coefficient is the inverse of the total thermal resistance of the materials’ assembly. Modifying the equation to include this gives:

This form of the equation now details the rate of heat loss through a material characterized by an area 𝐴 and an overall heat transfer coefficient 𝑈 across a temperature difference Δ𝑇.

Total Heat Loss Over Time: To determine the total heat loss for a specific duration, the time variable is typically included. However, if the interest lies in understanding the rate of heat loss per unit time, we retain the rate form

• 𝑄˙ stands for the rate of heat energy lost in joules per second, or watts, describing how quickly energy is transferred as heat through the specified assembly.

Usage of Heat Loss Formula

• Home Insulation: Contractors use the heat loss formula to determine the right amount of insulation needed for homes. This calculation ensures homes stay warm in winter and cool in summer without excessive energy use.
• HVAC Systems Design: Engineers apply the formula to design efficient heating, ventilation, and air conditioning systems that adequately manage the indoor climate of buildings while minimizing energy costs.
• Industrial Processes: In industries, the formula helps maintain specific temperatures in processes that require heat control, such as in chemical reactions or machinery operations.
• Energy Audits: Energy auditors use the heat loss formula to assess a building’s thermal performance. This evaluation helps identify opportunities for improving energy efficiency.
• Product Development: Manufacturers of thermal products like heaters and coolers calculate expected heat loss to enhance product design for optimal performance.

Example Problems on Heat Loss Formula

Example 1: Calculating Heat Loss from a House

Problem: Calculate the heat loss per hour from a house with walls that have a total area of 150 square meters. The overall heat transfer coefficient (U-value) of the walls is 0.35 W/m²°C. The temperature inside the house is 22°C, and the outside temperature is -3°C.

Solution:

Formula: 𝑄=𝑈×𝐴×Δ𝑇Q=U×A×ΔT

Given:

𝑈 = 0.35 W/m²°C

𝐴 = 150 m²

Δ𝑇 = 22 − (−3) = 25 °C

Calculate:

𝑄 = 0.35 × 150 × 25

𝑄 = 0.35 × 150 × 25 =1312.5 watts

Answer: The house loses 1,312.5 watts of heat per hour.

Example 2: Heat Loss through a Window

Problem: Determine the heat loss in watts through a window measuring 1.2 meters by 1.5 meters if the U-value is 1.8 W/m²°C and the temperature difference between the inside and outside is 15°C.

Solution:

Formula: 𝑄 = 𝑈 × 𝐴 × Δ𝑇

Given:

𝑈=1.8 W/m²°C

𝐴 = 1.2 × 1.5 = 1.8 m²

Δ𝑇 = 15 °C

Calculate:

𝑄=1.8×1.8×15

𝑄=1.8×1.8×15=48.6 watts

Answer: The window loses 48.6 watts of heat.

Example 3: Heat Loss from a Hot Water Pipe

Problem: A hot water pipe has a surface area of 0.75 m² and is exposed to a room where the temperature is consistently 18°C lower than the surface temperature of the pipe. If the U-value of the pipe’s insulation is 0.25 W/m²°C, calculate the heat loss.

Solution:

Formula: 𝑄 = 𝑈 × 𝐴 × Δ𝑇

Given:

𝑈 = 0.25 W/m²°C

𝐴 = 0.75 m²

Δ𝑇 = 18 °C

Calculate:

𝑄 = 0.25 × 0.75 × 18

𝑄 = 0.25 × 0.75 × 18 = 3.375 watts

Answer: The pipe loses 3.375 watts of heat.

FAQs

The formula for current heat loss is 𝑄=𝐼² × 𝑅 × 𝑡, where 𝐼 is current, R resistance, and t time.

What is the Formula for Heat Loss by Convection?

Heat loss by convection is calculated using 𝑄 = ℎ × 𝐴 × Δ𝑇 , where h is the convective heat transfer coefficient.

What is the Basic Formula for Heat Transfer?

The basic formula for heat transfer is 𝑄 = 𝑈 × 𝐴 × Δ𝑇, suitable for conduction and convection scenarios.