Hubbles Law Formula – Formula, Applications, Examples Problems

What is Hubbles Law Formula?

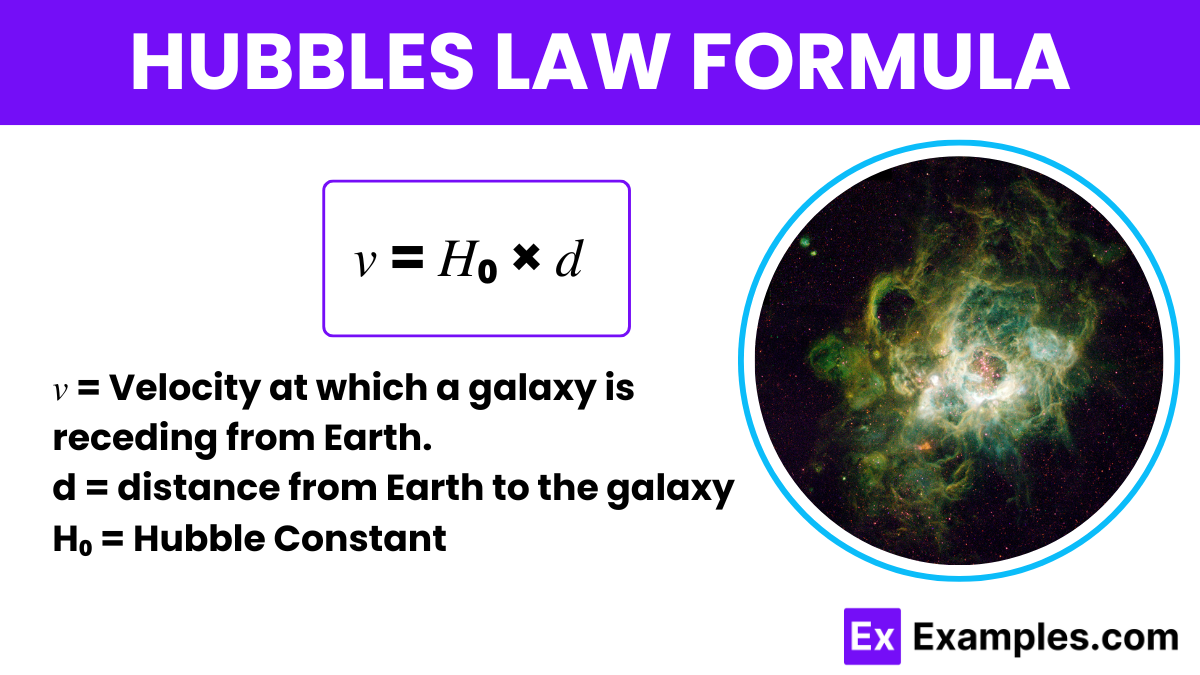
Hubble’s Law describes the relationship between the distance to a galaxy and the speed at which it moves away from us, reflecting the expansion of the universe. Edwin Hubble is an physics scientists, who discovered this phenomenon in 1929, revealing that the universe is expanding.

The formula for Hubble’s Law is:

• v represents the velocity at which a galaxy is receding from Earth, measured in kilometers per second.
• d is the distance from Earth to the galaxy, expressed in megaparsecs (Mpc).
• H₀ is the Hubble Constant, which approximates the rate of expansion of the universe.

To derive this formula, Hubble plotted the velocities of galaxies against their distances, establishing a linear relationship. The slope of this line, the Hubble Constant, indicates how fast the universe expands per megaparsec of distance.

This simple yet profound equation means that the farther a galaxy is from us, the faster it moves away. This observation supports the theory that the universe started from a singular event, commonly known as the Big Bang.

Applications of Hubble Law Formula

1. Determining the Age of the Universe: By measuring the Hubble Constant, scientists estimate the universe’s age, providing a timeline for its expansion and evolution.
2. Validating the Big Bang Theory: The law supports the Big Bang model by showing that galaxies move away from us in all directions, suggesting an initial explosive expansion.
3. Exploring Dark Energy: Hubble’s Law helps researchers study dark energy by observing the acceleration in the universe’s expansion rate over time.
4. Mapping the Universe’s Expansion: It assists in charting the rate of expansion and understanding how this rate has changed, offering insights into the dynamics of the cosmos.
5. Measuring Galactic Distances: Hubble’s Law provides a method for calculating the distances to faraway galaxies based on their redshift, which is crucial for creating detailed maps of the universe’s structure.
6. Understanding Galaxy Formation: By analyzing the speed and distance of galaxies, scientists can infer the history of galaxy formation and the evolution of large-scale structures in the universe.
7. Assessing Cosmic Scales: The formula helps adjust the cosmic distance ladder, a tool used to determine distances within the universe with increasing accuracy, enhancing our understanding of cosmic dimensions.

Limitations of Humble Law Formula

• Non-applicability to Local Group: The law does not accurately predict velocities and distances within our local group of galaxies due to gravitational interactions.
• Assumes Uniform Expansion: It assumes the universe expands uniformly, which may not account for variations due to structures like galaxy clusters.
• Redshift Interpretation: The law interprets all redshifts as due to expansion, potentially overlooking other causes such as gravitational effects.

Examples Problems on Hubble Law Formula

Problem 1: Calculating Recessional Velocity

Problem: If a galaxy is located 100 megaparsecs (Mpc) away from Earth, and the Hubble Constant (H₀) is estimated to be 70 kilometers per second per megaparsec (km/s/Mpc), what is the recessional velocity of this galaxy?

Solution: To find the recessional velocity (v), use the formula:

v=H₀ ​× d

Substitute the given values:

𝑣=70 km/s/Mpc×100 Mpc

𝑣=7000 km/s

The galaxy is moving away from Earth at a velocity of 7000 km/s.

Problem 2: Estimating Distance from Velocity

Problem: A galaxy is moving away from us at a velocity of 2100 km/s. Using a Hubble Constant of 70 km/s/Mpc, estimate the distance from Earth to this galaxy.

Solution: Rearrange the Hubble’s Law formula to solve for distance (d):

𝑑=𝑣 x 𝐻₀

Substitute the given values:

𝑑= (2100 km/s) / (70 km/s/Mpc)

𝑑=30 Mpcd

The galaxy is approximately 30 megaparsecs away from Earth.

Problem 3: Determining the Hubble Constant

Problem: Astronomers measure the recessional velocity of a galaxy as 2800 km/s. If this galaxy is known to be 40 megaparsecs (Mpc) away from Earth, calculate the Hubble Constant (H₀).

Solution: Rearrange Hubble’s Law to solve for the Hubble Constant 𝐻₀​:

𝐻₀=𝑣 / 𝑑

Substitute the given values: 𝐻₀= (2800 km/s) / (40 Mpc)

​ 𝐻₀=70 km/s/Mpc

The Hubble Constant is calculated to be 70 km/s/Mpc.

Problem 4: Predicting Future Velocity

Problem: Assuming the Hubble Constant remains constant at 70 km/s/Mpc, calculate the future recessional velocity of a galaxy currently 50 megaparsecs away, assuming it moves to 55 megaparsecs away from Earth.

Solution: First, use Hubble’s Law to calculate the current velocity:

𝑣 current=𝐻₀ × 𝑑 current = 70 km/s/Mpc × 50 Mpc

𝑣 current=3500 km/s

Next, calculate the future velocity:

𝑣 future=𝐻₀ × 𝑑 future=70 km/s/Mpc × 55 Mpc

𝑣 future=3850 km/s

The future recessional velocity of the galaxy, when it is 55 Mpc away, will be 3850 km/s.

FAQs

How to Calculate Hubble’s Constant?

Measure the slope of the velocity-distance graph for distant galaxies to find Hubble’s constant.

What is Hubble’s Constant Value?

As of the latest observations, Hubble’s constant is approximately 70 kilometers per second per megaparsec.

What is Hubble’s Length?

Hubble’s length, derived from Hubble’s constant, represents the scale of the observable universe, approximately 14 billion light-years.