Law of Conservation of Angular Momentum

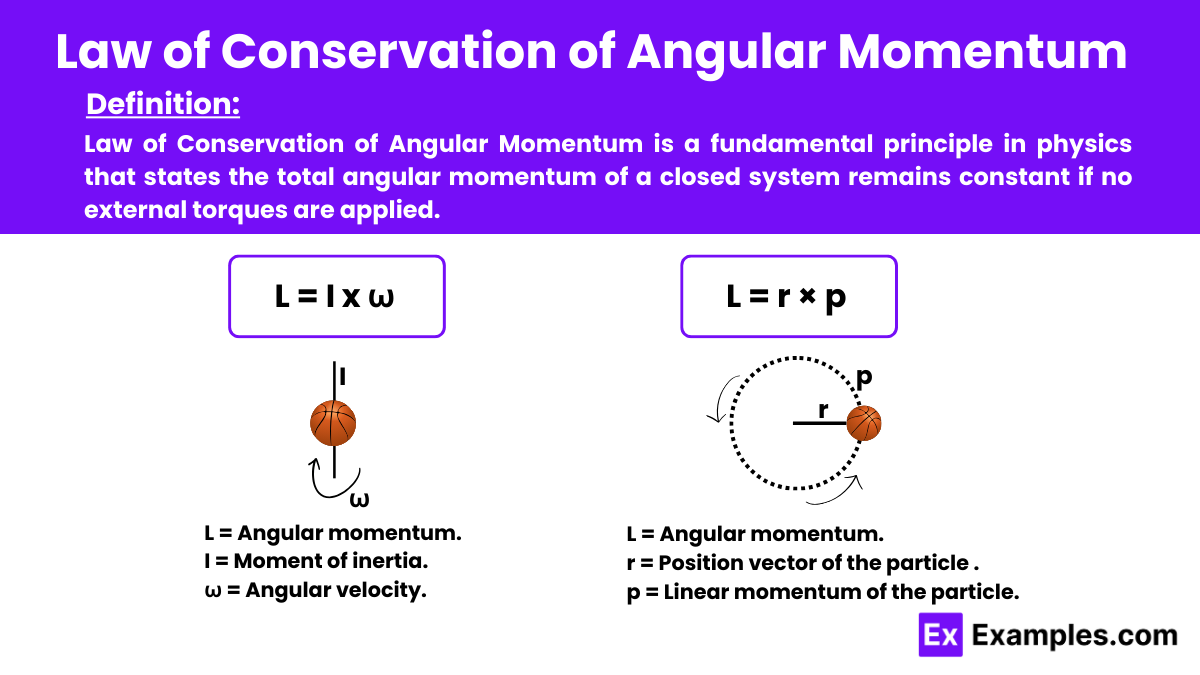
Law of Conservation of Angular Momentum is a fundamental principle in physics that states the total angular momentum of a closed system remains constant if no external torques are applied. This law of physics reflects the rotational analog of linear momentum conservation, emphasizing that angular momentum is conserved in systems where net external torque is zero. This principle is crucial for analyzing the rotational motion of various systems, from simple mechanical setups to complex celestial interactions.

What is Law of Conservation of Angular Momentum?

The Law of Conservation of Angular Momentum states that the total angular momentum of a system remains constant if no external torques act on it. This principle, a cornerstone in physics, particularly mechanics, applies to many phenomena, from the motion of orbiting celestial bodies to the behavior of spinning objects and moving particles. The law implies that in a closed system, where no external forces alter rotational motion, the system retains its initial angular momentum at any point in time.

Law of Conservation of Angular Momentum Formula

The Law of Conservation of Angular Momentum is mathematically represented by the formula:

𝐿=𝐼 x 𝜔

where:

• 𝐿 is the angular momentum,
• I is the moment of inertia of the object or system,
• 𝜔 (omega) is the angular velocity.

In a closed or isolated system where no external torques apply, the system keeps its total angular momentum constant. Thus, if the system starts with a specific angular momentum, it retains that angular momentum throughout the motion unless an external torque acts upon it. We can express this conservation as:

L = r x p

• 𝐿 is the angular momentum,
• r is the position vector of the particle relative to the point about which the momentum is measured
• p is the linear momentum of the particle.

This principle is crucial for understanding rotational dynamics in various physical contexts, from spinning objects to orbital mechanics in astronomy.

Torque and Angular Momentum Relationship

The relationship between torque and angular momentum is a fundamental concept in rotational dynamics. The key relationship is expressed by the following equation:

𝜏⃗=𝑑𝐿⃗/𝑑𝑡

where:

• 𝜏⃗ represents the torque applied to an object,
• 𝐿⃗ represents the angular momentum of the object,
• 𝑑𝐿⃗/𝑑𝑡 is the rate of change of angular momentum over time.

This equation means that the torque applied to a system is equal to the rate of change of its angular momentum. In simpler terms, any change in the angular momentum of a system is directly caused by and is proportional to the net external torque acting on it. When the net external torque is zero, angular momentum is conserved, and 𝑑𝐿⃗/𝑑𝑡=0, indicating no change in angular momentum.

This relationship is essential for analyzing scenarios where forces are applied off-center to rotating objects. Such as in wheels, spinning tops, planets in orbit, or any system undergoing rotational motion. The direction of the torque vector also determines the direction in which the angular momentum changes. According to the right-hand rule: if you curl the fingers of your right hand in the direction of rotation caused by the torque, your thumb points in the direction of the angular momentum vector.

Uses of Law of Conservation of Angular Momentum

• Figure Skating: Athletes increase their spinning speed by pulling their arms and legs close to their body. By reducing their moment of inertia, their angular velocity increases, demonstrating the conservation of angular momentum.
• Astronomy and Planetary Motion: Planets accelerate in their orbit when they are closer to the sun and decelerate when farther away. This variation in speed maintains constant angular momentum, as described by Kepler’s second law.
• Diving: Divers manipulate their rotation speed by tucking their bodies into a smaller shape or extending them to slow down. This action conserves angular momentum during a dive.
• Rotating Spacecraft: Space agencies use reaction wheels and gyroscopes to orient satellites and spacecraft. They spin internal components in one direction to rotate the craft in the opposite direction, leveraging the conservation of angular momentum to make precise adjustments.
• Tornado Formation: As air masses converge and rise, they create smaller, faster-spinning vortexes due to the conservation of angular momentum, similar to the effect seen in ice skaters.
• Wind Turbines: Wind turbines maintain stability and convert the wind’s kinetic energy into electricity by applying principles of angular momentum conservation to their rotating blades.

Examples for Law of Conservation of Angular Momentum

• Cat’s Mid-Air Rotation: When a cat falls, it instinctively adjusts its body position to land on its feet. By rotating its front and hind parts in opposite directions. The cat uses the conservation of angular momentum to orient itself quickly while falling.
• Ballet Pirouette: In ballet, dancers increase their rotation speed during a pirouette by drawing their arms and legs close to their body. This action reduces their moment of inertia. Causing them to spin faster to maintain their angular momentum.
• Synchronized Swimming: Synchronized swimmers often perform underwater spins. By tucking in their limbs or extending them, they control their rotation speed, conserving angular momentum as they perform complex movements.
• Hammer Throw: In athletics, the hammer throw athlete rotates with the hammer to build angular momentum. The athlete releases the hammer at the right moment, and its high speed is a result of the conservation of angular momentum generated during the spin.
• Star Collapse to Neutron Star: In astronomy, a massive star’s core collapses into a neutron star during a supernova. The collapse reduces its radius dramatically. Which increases the star’s rotation speed significantly while conserving angular momentum.
• Helicopter Tail Rotor: Helicopters use tail rotors to counteract the spinning caused by the main rotor. By changing the direction of the tail rotor’s thrust, pilots can control the helicopter’s spin to maintain stability using angular momentum principles.

FAQ’S

What do you mean by angular momentum?

Angular momentum represents an object’s rotational momentum, calculated as the product of its moment of inertia and angular velocity. It describes the quantity of rotation an object has.

Is momentum always conserved?

Yes, momentum is always conserved in a closed system. The total momentum remains constant if no external forces act on the system, according to the law of conservation of momentum.

Is momentum scalar or vector?

Momentum is a vector. It has both magnitude and direction. With the direction of the momentum vector corresponding to the direction of the object’s velocity.