Law of Conservation of Energy – Examples, Definition, Uses, FAQ’S

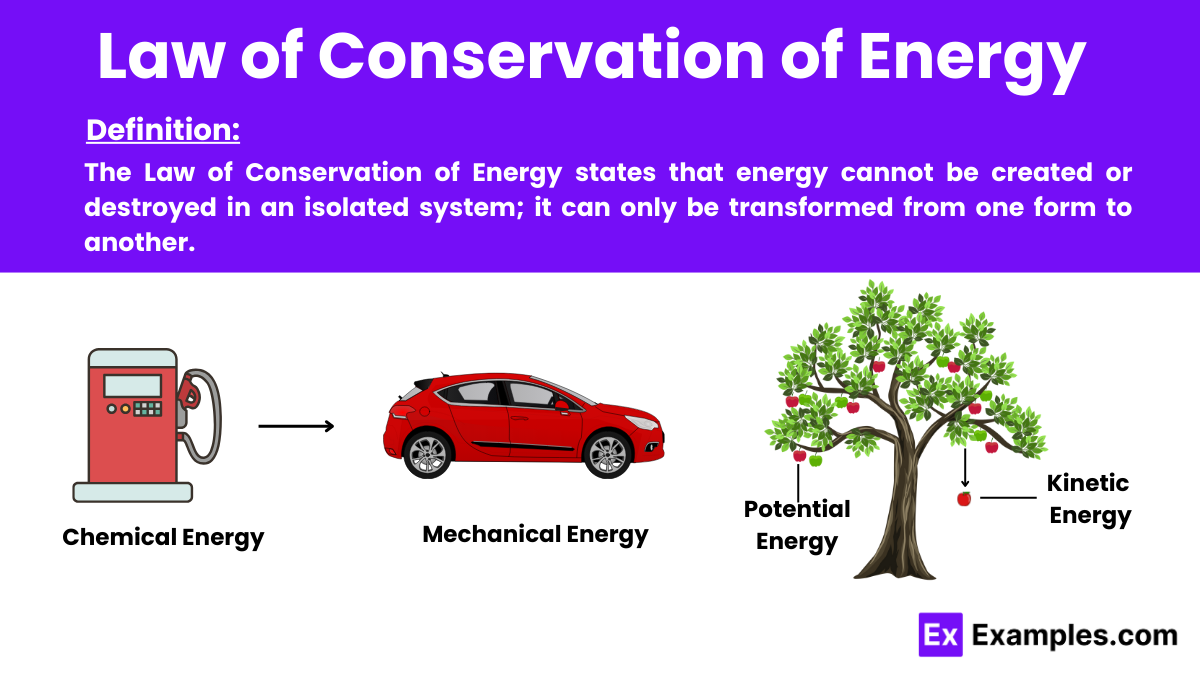
In physics, the Law of Conservation of Energy states that energy cannot be created or destroyed in an isolated system; it can only be transformed from one form to another. This fundamental principle is one of the key laws of physics and underscores the constancy of energy in all physical processes, whether it involves mechanical, thermal, chemical, or electrical energy transformations.

What is Law of Conservation of Energy?

Types of Law of Conservation of Energy

The Law of Conservation of Energy manifests in several forms, each relevant to different physical contexts:

Mechanical Energy Conservation

In mechanical systems, energy shifts between kinetic energy (movement energy) and potential energy (stored energy due to position). For example, a swinging pendulum exchanges its height (potential energy) for speed (kinetic energy) and back.

Thermal Energy Conservation

This form deals with heat energy. When energy transforms into heat, as with friction, it remains within the system, complying with conservation principles, such as in insulated systems where heat does not escape.

Chemical Energy Conservation

In chemical reactions, the energy stored in bonds of reactants transforms into products or releases as heat, yet the total energy remains constant. This principle is crucial for understanding reactions in batteries or metabolic processes in biology.

Electrical Energy Conservation

In electrical circuits, energy converts from electrical potential to other forms like light or heat without loss of total system energy, important for power management and efficiency in electrical systems.

Law of Conservation of Energy Formula

The formula for the Law of Conservation of Energy is straightforward:

This equation states that the total energy 𝐸ₜₒₜₐₗ​ of an isolated system remains constant. Meaning the initial energy 𝐸ᵢₙᵢₜᵢₐₗ​ before any processes occur equals the final energy 𝐸բᵢₙₐₗ​ after those processes. This encapsulates all forms of energy, including kinetic, potential, thermal, and others, ensuring their sum remains unchanged regardless of the transformations that occur within the system

Law of Conservation of Energy Formula Derivation

The derivation of the Law of Conservation of Energy formula is grounded in the fundamental principle that energy cannot be created or destroyed, only transformed from one form to another. Here’s a simplified explanation of how this principle leads to the conservation of energy equation:

Step 1: Understanding Energy Forms

Energy can exist in various forms such as kinetic, potential, thermal, electrical, and chemical. Each form can be quantitatively described by specific equations. Like kinetic energy 𝐾𝐸=1/2𝑚𝑣² and potential energy 𝑃𝐸=𝑚𝑔ℎ, where:

• 𝑚 = mass,
• 𝑣 = velocity,
• g = acceleration due to gravity,
• ℎ = height above a reference point.

Step 2: Energy Transformation

Consider a closed system where energy transformations occur without any external energy input or loss to the surroundings. For instance, a pendulum swinging back and forth converts its energy from kinetic to potential and back without losing energy to the environment.

Step 3: Setting Up the Energy Balance

In any process within a closed system, the total energy at any time can be represented by the sum of all forms of energy present. For the swinging pendulum:

• At the highest points, kinetic energy is zero, and potential energy is maximum.
• At the lowest point, kinetic energy is maximum, and potential energy is minimum.

Step 4: Conservation Equation

By setting up an equation that balances the total energies at different points in time or different states of the system. You acknowledge that the sum of energies remains constant. Mathematically, this is expressed as: Total Energy at Start=Total Energy at End 𝐸ᵢₙᵢₜᵢₐₗ=𝐸բᵢₙₐₗ

Uses of Law of Conservation of Energy

The Law of Conservation of Energy has wide-ranging applications across various fields, demonstrating its fundamental role in both theoretical and practical aspects:

• Engineering Design: Engineers design mechanical systems, such as engines and turbines. To maximize energy efficiency by minimizing energy losses to heat and friction.
• Environmental Science: Researchers analyze ecosystems to understand energy flow among organisms and use this information to study sustainability and conservation strategies.
• Building and Architecture: Architects incorporate energy-conserving principles into building designs to improve insulation and reduce heating and cooling costs.
• Physics Education: Educators use this law to teach fundamental physics concepts. Helping students understand energy transformations in different systems.
• Renewable Energy Technology: Developers create more efficient solar panels, wind turbines, and other renewable energy technologies that harness natural energy sources while minimizing energy waste.

Examples for Law of Conservation of Energy

Here are practical examples demonstrating the Law of Conservation of Energy in everyday and scientific contexts:

• Roller Coasters: A roller coaster converts potential energy into kinetic energy as it descends from the highest point of the track, then back into potential energy as it climbs again.
• Bouncing Ball: When you drop a ball, it converts its potential energy into kinetic energy as it falls. Upon bouncing, it converts some of that kinetic energy back into potential energy.
• Electric Vehicles: Electric vehicles store electrical energy in batteries. This energy transforms into mechanical energy to power the wheels and kinetic energy when the vehicle is moving.
• Photosynthesis: In photosynthesis, plants convert solar energy into chemical energy stored in glucose, demonstrating energy transformation essential for life.
• Thermal Power Plants: Power plants burn fuel to produce thermal energy, which then converts to mechanical energy in turbines and finally into electrical energy through generators.

FAQ’S

Why can energy not be created or destroyed?

According to the Law of Conservation of Energy, energy simply transforms from one form to another, maintaining constant total energy in a closed system.

Can energy be destroyed in a black hole?

In black holes, energy is not destroyed; rather, it becomes trapped indefinitely, effectively removing it from the observable universe but still conserved.

What are the three parts of the law of conservation of energy?

The law asserts that energy remains constant. It transforms between different forms, and cannot disappear or emerge from nothing in an isolated system.