Which principle states that the pressure of a fluid decreases as its velocity increases?
Pascal's Principle
Bernoulli's Principle
Archimedes' Principle
Continuity Equation

Fluid dynamics, a captivating area of physics, explores how fluids (liquids and gases) behave when they are in motion. This branch of physics utilizes various laws of physics to predict and analyze the fluid flow in different scenarios. Alike the flow of air over an airplane wing or water running through pipes. The fundamental laws governing fluid dynamics include Bernoulli’s Principle, which links the speed of a fluid to its pressure, and the continuity equation that assures the mass of fluids in a system remains constant during flow.
The Laws of Fluid Dynamics describe how fluids (liquids and gases) behave under various conditions. These laws consist of three main principles. They are, the Law of Conservation of Mass, which states that mass cannot be created or destroyed in a closed system; Bernoulli’s Principle, which explains that an increase in the speed of a fluid occurs simultaneously with a decrease in pressure or potential energy; and the Law of Viscosity, which tells us how the internal resistance of fluids affects their flow. These principles help scientists and engineers predict how fluids will move, mix, and exert forces in different environments. By making them fundamental to understanding and designing systems in engineering, meteorology, and many other fields.
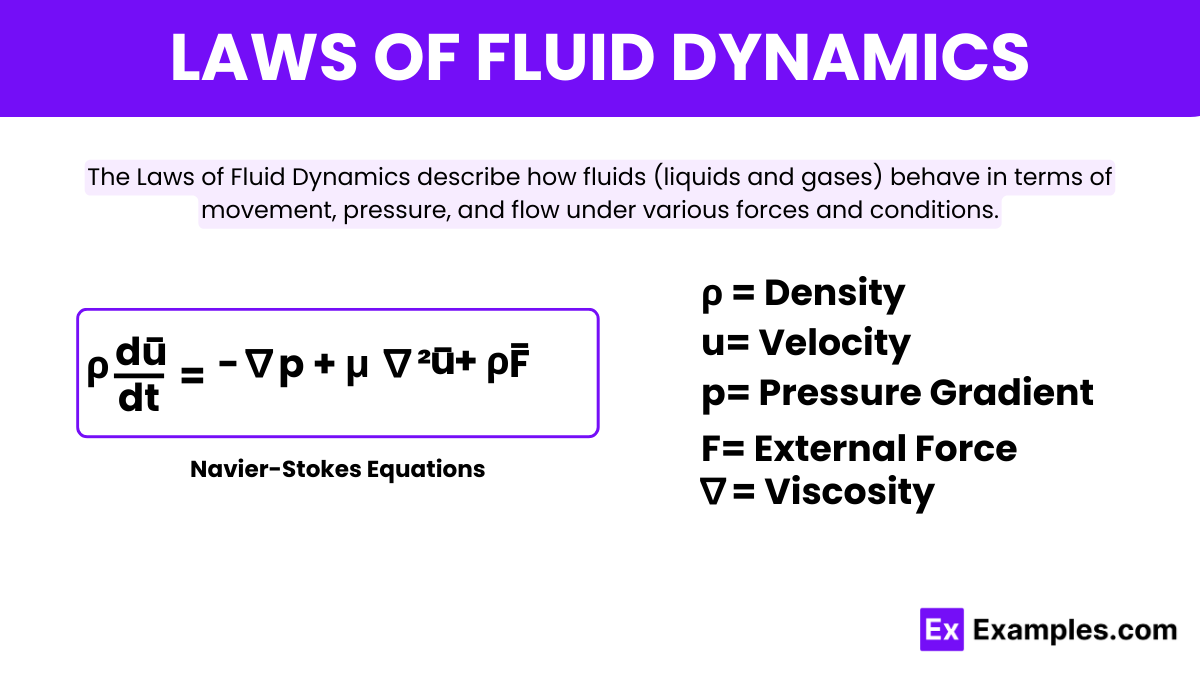
Fluid dynamics is governed by several key equations that describe the movement and behavior of fluids. The most fundamental is the Navier-Stokes Equation, which establishes how the velocity of a fluid changes in response to forces and energy transfer. This equation accounts for the fluid’s viscosity, pressure, and density, enabling detailed predictions of flow patterns in complex scenarios. Another crucial equation is the Continuity Equation, which ensures that the mass of a fluid remains constant as it flows from one point to another, expressing the principle of mass conservation in fluid dynamics. These equations are indispensable tools in fields such as aerospace, mechanical engineering, and environmental science, where understanding fluid behavior is essential.
The Navier-Stokes equations, named after Claude-Louis Navier and George Gabriel Stokes, are a set of partial differential equations that describe the motion of fluid substances such as liquids and gases. These equations fundamentally express how the velocity field of a fluid evolves over time and space due to various forces acting on it. Specifically, they balance the forces of inertia, viscous forces, pressure, and external forces within a fluid. This balance is crucial for predicting how fluids flow under different conditions, such as varying speeds, viscosities, and interacting forces.
Central to the Navier-Stokes equations is their ability to model fluid dynamics under the influences of viscosity, which is the internal friction within the fluid, and pressure, which is the force exerted uniformly in all directions. These equations apply Newton’s second law of motion to fluid motion, incorporating the concepts of momentum and force continuity. The result is a comprehensive description that can predict changes in the flow and speed of the fluid at any given point.
Due to their complexity, the Navier-Stokes equations are often solved with numerical methods and computational simulations. It is especially in scenarios involving turbulent flows or complex boundaries. Engineers and physicists use these equations to design efficient fluid transport systems, predict weather patterns, and even model blood flow in the human body. The ability to solve the Navier-Stokes equations accurately is thus central to advancements in both scientific research and practical applications in engineering.
Fluid dynamics is complex due to the nonlinear nature of fluid motion and the influence of multiple interacting variables like flow velocity and pressure.
There are three primary laws of dynamics: Newton’s three laws of motion, which govern the behavior of all physical objects, including fluids.
An example of fluid dynamics is the swirling pattern of milk stirred into coffee, illustrating fluid motion and mixing behavior.

Fluid dynamics, a captivating area of physics, explores how fluids (liquids and gases) behave when they are in motion. This branch of physics utilizes various laws of physics to predict and analyze the fluid flow in different scenarios. Alike the flow of air over an airplane wing or water running through pipes. The fundamental laws governing fluid dynamics include Bernoulli’s Principle, which links the speed of a fluid to its pressure, and the continuity equation that assures the mass of fluids in a system remains constant during flow.
The Laws of Fluid Dynamics describe how fluids (liquids and gases) behave under various conditions. These laws consist of three main principles. They are, the Law of Conservation of Mass, which states that mass cannot be created or destroyed in a closed system; Bernoulli’s Principle, which explains that an increase in the speed of a fluid occurs simultaneously with a decrease in pressure or potential energy; and the Law of Viscosity, which tells us how the internal resistance of fluids affects their flow. These principles help scientists and engineers predict how fluids will move, mix, and exert forces in different environments. By making them fundamental to understanding and designing systems in engineering, meteorology, and many other fields.
Fluid dynamics is governed by several key equations that describe the movement and behavior of fluids. The most fundamental is the Navier-Stokes Equation, which establishes how the velocity of a fluid changes in response to forces and energy transfer. This equation accounts for the fluid’s viscosity, pressure, and density, enabling detailed predictions of flow patterns in complex scenarios. Another crucial equation is the Continuity Equation, which ensures that the mass of a fluid remains constant as it flows from one point to another, expressing the principle of mass conservation in fluid dynamics. These equations are indispensable tools in fields such as aerospace, mechanical engineering, and environmental science, where understanding fluid behavior is essential.
The Navier-Stokes equations, named after Claude-Louis Navier and George Gabriel Stokes, are a set of partial differential equations that describe the motion of fluid substances such as liquids and gases. These equations fundamentally express how the velocity field of a fluid evolves over time and space due to various forces acting on it. Specifically, they balance the forces of inertia, viscous forces, pressure, and external forces within a fluid. This balance is crucial for predicting how fluids flow under different conditions, such as varying speeds, viscosities, and interacting forces.
Central to the Navier-Stokes equations is their ability to model fluid dynamics under the influences of viscosity, which is the internal friction within the fluid, and pressure, which is the force exerted uniformly in all directions. These equations apply Newton’s second law of motion to fluid motion, incorporating the concepts of momentum and force continuity. The result is a comprehensive description that can predict changes in the flow and speed of the fluid at any given point.
Due to their complexity, the Navier-Stokes equations are often solved with numerical methods and computational simulations. It is especially in scenarios involving turbulent flows or complex boundaries. Engineers and physicists use these equations to design efficient fluid transport systems, predict weather patterns, and even model blood flow in the human body. The ability to solve the Navier-Stokes equations accurately is thus central to advancements in both scientific research and practical applications in engineering.
Aerospace Engineering: Engineers apply fluid dynamics to design aircraft and spacecraft. This reduces drag and improves performance.
Automotive Engineering: Fluid dynamics enhances vehicle aerodynamics. This boosts fuel efficiency and stability.
Civil Engineering: Fluid dynamics is essential in water management for dams, rivers, and sewage systems.
Environmental Science: It aids in forecasting weather, studying ocean currents, and modeling pollution spread.
Medical Science: Fluid dynamics analyzes blood flow, aiding in the design of heart valves and stents.
Industrial Processes: It’s crucial in systems that transport liquids and gases, such as pipelines and air conditioners.
Energy Sector: Fluid dynamics is vital in optimizing wind turbines and hydroelectric stations for better efficiency and output.
Fluid dynamics is complex due to the nonlinear nature of fluid motion and the influence of multiple interacting variables like flow velocity and pressure.
There are three primary laws of dynamics: Newton’s three laws of motion, which govern the behavior of all physical objects, including fluids.
An example of fluid dynamics is the swirling pattern of milk stirred into coffee, illustrating fluid motion and mixing behavior.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
Which principle states that the pressure of a fluid decreases as its velocity increases?
Pascal's Principle
Bernoulli's Principle
Archimedes' Principle
Continuity Equation
What does the Continuity Equation in fluid dynamics represent?
Conservation of energy
Conservation of momentum
Conservation of mass
Conservation of pressure
According to Pascal's Principle, how does pressure applied to a confined fluid behave?
It decreases exponentially
It increases only at the point of application
It is transmitted equally in all directions
It creates a vacuum
Which law explains the buoyant force experienced by objects submerged in a fluid?
Bernoulli's Principle
Continuity Equation
Archimedes' Principle
Pascal's Principle
What does Bernoulli's Equation relate in fluid flow?
Pressure, temperature, and volume
Velocity, pressure, and height
Density, viscosity, and flow rate
Mass, energy, and momentum
Which equation expresses the relationship between pressure, velocity, and cross-sectional area in a fluid flow?
Bernoulli's Equation
Continuity Equation
Pascal's Equation
Navier-Stokes Equation
What is the primary assumption in the application of Bernoulli's Principle?
Fluid is incompressible and non-viscous
Fluid has constant density and viscosity
Fluid is compressible and viscous
Fluid has variable density and temperature
Which principle can be used to explain why an airplane wing generates lift?
Archimedes' Principle
Bernoulli's Principle
Pascal's Principle
Continuity Equation
In fluid dynamics, what does the term "laminar flow" refer to?
Turbulent and chaotic flow
Flow with varying pressure
Smooth and orderly flow
Flow with increasing velocity
What is the Navier-Stokes Equation used to describe?
Fluid flow in open channels
Conservation of mass in fluids
Motion of viscous fluid substances
Static pressure in fluids
Before you leave, take our quick quiz to enhance your learning!

