Newtons Law of Viscosity – Examples, Definition, Formula, FAQ’S

Newton’s Law of Viscosity is a fundamental concept in the realm of fluid mechanics, which is a branch of physics that deals with the behavior of fluids (liquids and gases) and the forces acting upon them. This law provides a quantitative description of the flow of fluids, and it is essential for understanding how viscous forces are characterized in various physical situations.

What is Newtons Law of Viscosity?

Newtons Law of Viscosity Formula

The formula for Newton’s Law of Viscosity is expressed as:

where:

• 𝜏 (tau) represents the shear stress in the fluid,
• 𝜇 (mu) is the dynamic viscosity, which measures the fluid’s resistance to gradual deformation by shear stress or tensile stress,
• 𝑑𝑣/𝑑𝑦 is the velocity gradient perpendicular to the direction of shear.

Types of Fluids

Fluids, substances that can flow and conform to the shape of their containers, play critical roles in both natural and industrial processes. They are primarily categorized based on their ability to resist shear stress and their viscosity behavior under varying conditions. Understanding the different types of fluids is essential for fields such as engineering, meteorology, and various scientific research areas.

Newtonian Fluids

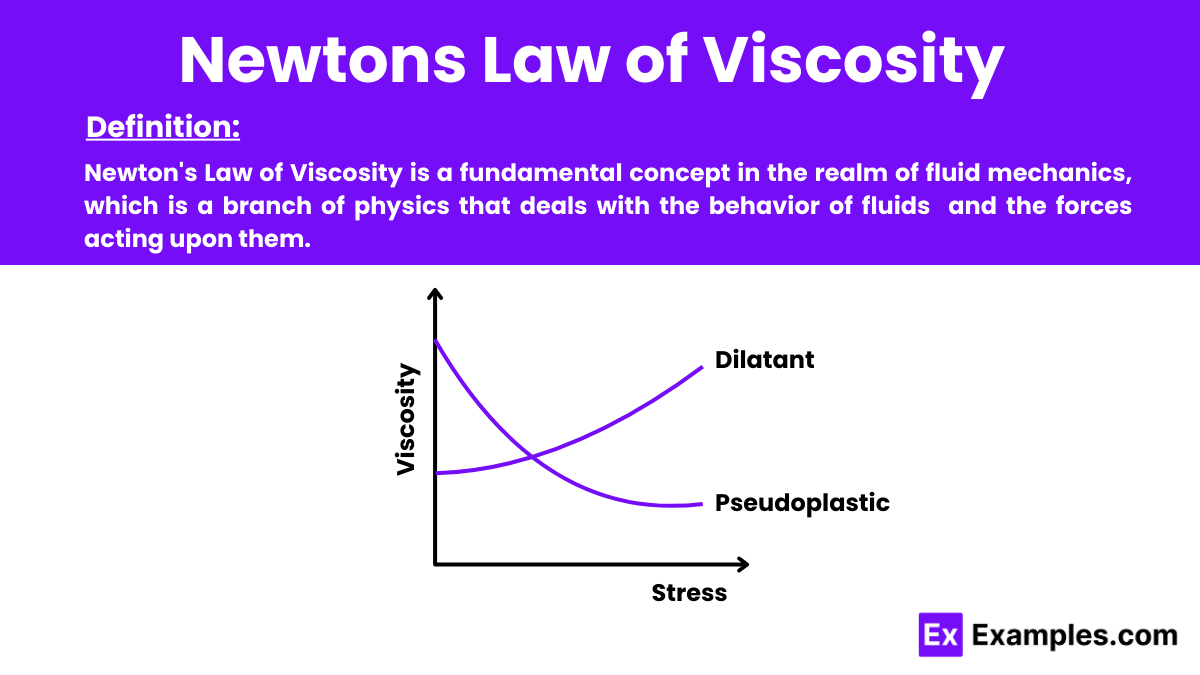
Newtonian fluids exhibit a constant viscosity regardless of the applied shear rate. Their shear stress is directly proportional to the shear rate, making their behavior predictable and consistent under different flow conditions. Common examples include water and most gases, which are staples in countless applications across industries.

Non-Newtonian Fluids

Non-Newtonian fluids do not have a constant viscosity; their viscosity can change when under force to either increase or decrease, depending on the type. They are further divided into several subcategories:

• Shear-thinning fluids decrease in viscosity as shear stress increases, such as latex paint or nail polish.
• Shear-thickening fluids increase in viscosity when subjected to shear stress, like cornstarch in water or some printing inks.
• Bingham plastics that behave like a solid until sufficient shear stress is applied, then flow like a fluid, examples include toothpaste and mayonnaise.

Ideal Fluids

Ideal fluids are hypothetical and do not exhibit viscosity or compressibility. They are used in theoretical physics to simplify the analysis of fluid dynamics by removing complex variables from the equations. While not practical in real-world applications, they provide essential insights into fluid behavior under idealized conditions.

Real Fluids

Real fluids, which include all fluids in nature and industry, exhibit viscosity. They are the opposite of ideal fluids, facing resistance due to their viscosity, which impacts their flow and behavior under various physical conditions.

Compressible and Incompressible Fluids

• Compressible fluids can experience significant changes in density when subjected to pressure changes. Gases are typically compressible because their molecules are far apart, allowing for density adjustments under pressure.
• Incompressible fluids have a constant density regardless of the applied pressure. Most liquids are considered incompressible due to their closely packed molecules, which are not easily compressed.

Uses of Newtons Law of Viscosity

• Lubrication Systems: Newton’s Law of Viscosity is crucial for designing effective lubrication strategies in mechanical systems, ensuring that moving parts operate smoothly with reduced wear and tear.
• Manufacturing Processes: This law aids in determining the optimal flow conditions for fluids in manufacturing processes, such as in the production of paints and other materials where viscosity plays a critical role.
• Hydraulic Systems: Newton’s Law of Viscosity guides the design and maintenance of hydraulic systems, relying on the predictable behavior of fluid under pressure to perform mechanical work.
• Oil Recovery and Pipeline Transport: In the oil industry, understanding viscosity is essential for efficiently extracting and transporting crude oil. This law helps predict how oil behaves under various temperature and pressure conditions.
• Medical Diagnostics: Medical technology uses this law to analyze blood flow dynamics, assisting in the diagnosis and treatment of cardiovascular diseases.
• Environmental Monitoring and Cleanup: Newton’s Law of Viscosity models the dispersion of pollutants in water bodies, aiding in environmental assessments and remediation efforts.

Examples for Newtons Law of Viscosity

• Automotive Oils: Engineers formulate engine oils based on their viscosity characteristics to ensure they provide a stable lubricating film between moving parts at various operating temperatures, crucial for engine performance and longevity.
• Blood Flow in Arteries: Medical professionals apply the principles of Newton’s Law of Viscosity to understand how blood flows through arteries and veins, essential for diagnosing cardiovascular conditions.
• Paint Application: The viscosity of paint dictates how it flows and spreads on a surface. Adjusting the viscosity is crucial for achieving the desired coating thickness and finish quality.
• Food Processing: In the food industry, manufacturers carefully control the viscosity of sauces and syrups to ensure consistent quality and flavor coverage, influencing pouring and coating behavior.
• Manufacturing of Plastics: During injection molding, the flow of molten plastic, governed by viscosity, requires proper understanding and control to ensure high-quality finished products with accurate dimensions and smooth surfaces.
• Cosmetic Products: The viscosity of lotions and creams is critical for their ease of application and absorption into the skin. Manufacturers adjust viscosity to meet consumer preferences and product performance specifications.

FAQ’S

What did Newton observe about viscosity?

Newton observed that the viscous force in fluids is proportional to the velocity gradient perpendicular to the flow direction.

Is Newton a unit of viscosity?

No, Newton is not a unit of viscosity. The unit of dynamic viscosity is the Pascal-second (Pa·s).

What is Newton’s law of viscosity in 3D?

In 3D, Newton’s law states that the stress tensor is proportional to the velocity gradient tensor, modeling more complex flow dynamics in fluids.