What principle states that it is impossible to know both the exact position and momentum of a particle simultaneously?
Pauli Exclusion Principle
Heisenberg Uncertainty Principle
Schrödinger Equation
Planck's Hypothesis
of 10
Quantum Physics – 10+ Examples, Formulas, Laws, Differences
Quantum Physics – 10+ Examples, Formulas, Laws, Differences
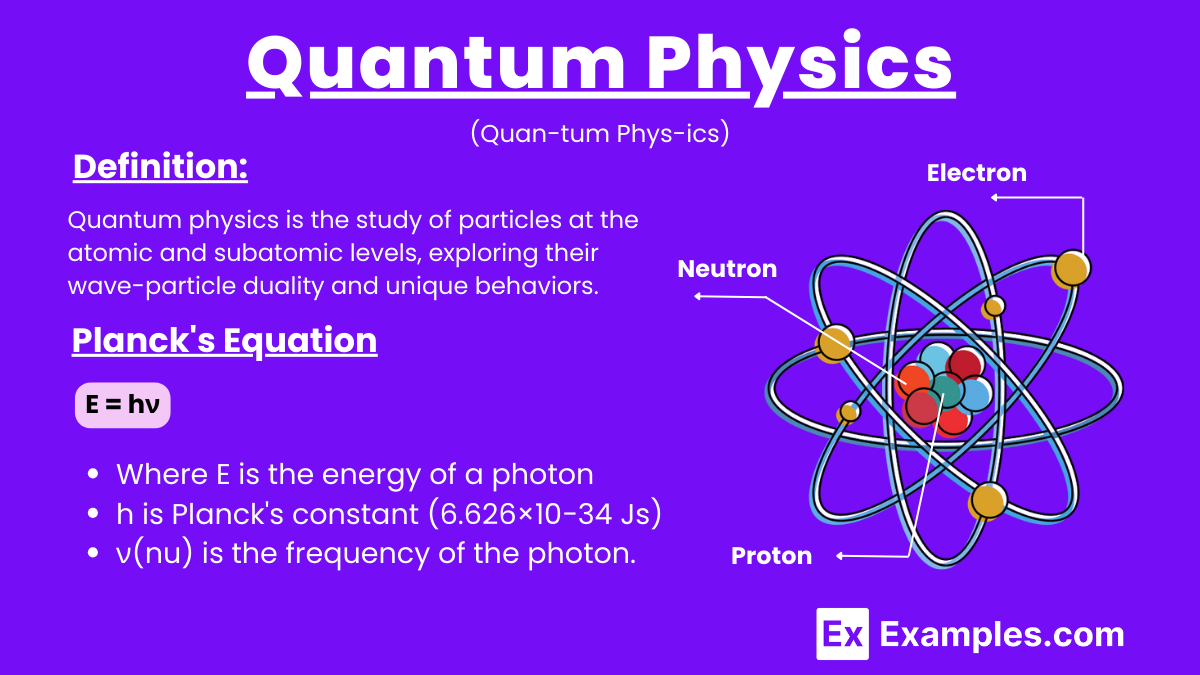
Quantum physics is the branch of physics that explores the behavior and interactions of particles at atomic and subatomic levels. It introduces concepts such as quantization of energy, wave-particle duality, the uncertainty principle, and quantum entanglement. These principles, along with the laws of quantum mechanics, the laws of atomic and molecular physics, and quantum field theory, provide a framework for understanding the complex and often counterintuitive nature of the microscopic universe.
What Is Quantum Physics?
Quantum physics is the study of particles at the atomic and subatomic levels, exploring their wave-particle duality and unique behaviors. It introduces concepts like energy quantization, uncertainty principle, superposition, and entanglement, which challenge classical physics and enable advancements in technology such as semiconductors, lasers, and quantum computing.
Quantum Physics Formulas
Quantum physics involves several fundamental formulas that describe the behavior of particles at the atomic and subatomic levels. Here are some of the key formulas:
Planck’s Equation
Where E is the energy of a photon, h is Planck’s constant (6.626×10−34 Js), and ν(nu) is the frequency of the photon.
Heisenberg’s Uncertainty Principle
Where Δx is the uncertainty in position, Δp is the uncertainty in momentum, and h is Planck’s constant.
Schrödinger Equation
Where i is the imaginary unit, ℏ is the reduced Planck’s constant (h/2π), ψ is the wave function, t is time, and H^ is the Hamiltonian operator.
De Broglie Wavelength
Where λ is the wavelength, h is Planck’s constant, and p is the momentum of the particle.
Energy Levels in a Hydrogen Atom
Where En is the energy of the nth level, and n is the principal quantum number.
Examples of Quantum Physics
Quantum physics governs many phenomena in the microscopic world, with practical implications and observable effects in various technologies and natural phenomena. Here are some notable examples:
- Photoelectric Effect : Light shining on a metal surface ejects electrons, demonstrating the particle nature of light.
- Quantum Tunneling : Particles pass through barriers they classically shouldn’t, enabling phenomena like nuclear fusion in stars.
- Superposition and Quantum Entanglement : Particles exist in multiple states simultaneously (superposition) and can instantly affect each other’s state over distances (entanglement).
- Double-Slit Experiment : Electrons create an interference pattern when passed through two slits, showing their wave-like behavior.
- Quantum Computing : Uses qubits that can exist in multiple states to perform complex calculations more efficiently than classical computers.
- Quantum Dot : refers to a nanoscale particle that has quantum mechanical properties, leading to unique optical and electronic behaviors.
- Heisenberg’s Uncertainty Principle : States that one cannot simultaneously know the exact position and momentum of a particle
- Bose-Einstein Condensate : A state of matter formed at temperatures close to absolute zero, where particles occupy the same quantum state and behave as a single quantum entity. Used in precision measurements and studying quantum phenomena at macroscopic scales.
- Quantum Teleportation : Transfer of quantum information from one particle to another over a distance without physical transmission of the particle itself.
- Quantum Harmonic Oscillator : A model that describes particles in a potential well, fundamental to understanding vibrational modes in molecules. Basis for spectroscopy and the study of molecular vibrations.
- Casimir Effect : A physical force arising from the quantum vacuum fluctuations between two closely spaced conducting plates. Relevant in nanotechnology and the development of microelectromechanical systems (MEMS).
- Quantum Zeno Effect : A phenomenon where frequent observation of a quantum system can inhibit its evolution. Potential use in controlling quantum systems and in quantum computing for error correction.
Quantum Physics Laws
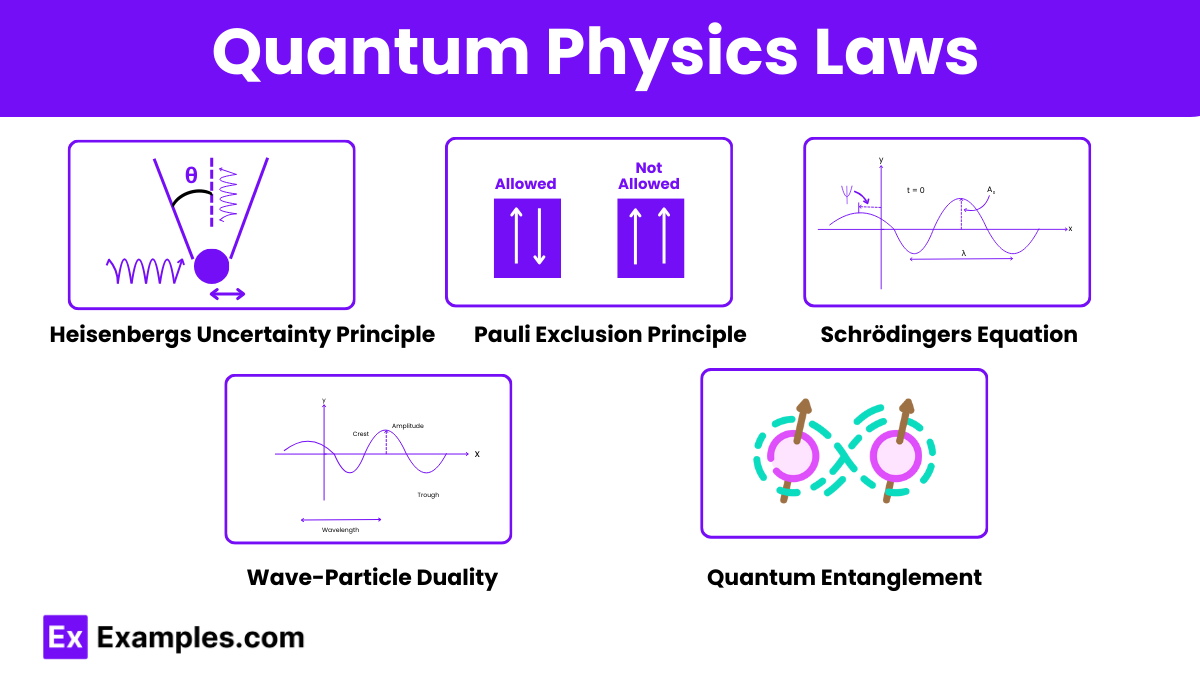
Quantum physics is governed by several fundamental laws and principles that describe the behavior of particles at the atomic and subatomic levels. Here are some of the key laws:
1. Planck’s Law
Energy is quantized and can be emitted or absorbed in discrete units called quanta. Planck’s law introduced the concept of quantized energy levels, which was pivotal in the development of quantum theory.
2. Heisenberg’s Uncertainty Principle
It is impossible to simultaneously know both the exact position and exact momentum of a particle. This principle implies a fundamental limit to the precision with which certain pairs of physical properties can be known, reflecting the inherent probabilistic nature of quantum mechanics.
3. Pauli Exclusion Principle
No two fermions (e.g., electrons) can occupy the same quantum state simultaneously within an atom. This principle explains the structure of electron shells in atoms and the behavior of electrons in solids, and it is crucial for understanding the properties of matter.
4. Wave-Particle Duality
Particles exhibit both wave-like and particle-like properties. Demonstrated through experiments like the double-slit experiment, this principle is fundamental to understanding quantum behavior. Electrons, for example, can produce interference patterns (a wave property) but also exhibit particle-like collisions.
5. Schrödinger Equation
Describes how the quantum state of a physical system changes over time. The Schrödinger equation is a fundamental equation of quantum mechanics, providing a way to predict the behavior of particles in a quantum system over time.
6. Quantum Entanglement
Particles can become entangled such that the state of one particle is dependent on the state of another, no matter the distance between them. This non-local property challenges classical notions of separability and has profound implications for quantum communication and computing.
Quantum Mechanics vs Quantum Physics
| Aspect | Quantum Mechanics | Quantum Physics |
|---|---|---|
| Definition | A branch of physics that deals specifically with the mathematical framework for describing the behavior of particles at the quantum level. | A broader term that encompasses the entire field studying the behavior and interactions of particles at the atomic and subatomic levels. |
| Scope | Focuses on the mathematical formulations and principles that describe the quantum state of systems. | Includes quantum mechanics as well as quantum field theory, quantum statistics, and other subfields. |
| Key Concepts | Wave functions, Schrödinger equation, Heisenberg uncertainty principle, quantum entanglement, superposition. | Includes all concepts from quantum mechanics plus additional topics like quantum chromodynamics and quantum electrodynamics. |
| Applications | Primarily theoretical, providing the foundation for understanding atomic and subatomic particles’ behavior. | Practical applications in technology (e.g., semiconductors, lasers, quantum computing), as well as theoretical advancements. |
| Mathematical Rigor | Highly mathematical, involving complex equations and models to predict quantum states and behaviors. | Can be less mathematically rigorous when discussing general principles and experimental observations. |
| Historical Development | Developed through the early 20th century by scientists like Planck, Einstein, Schrödinger, Heisenberg, and Dirac. | Encompasses the historical development of quantum mechanics and the subsequent advancements in understanding quantum phenomena. |
Classical Physics vs Quantum Physics
| Aspect | Classical Physics | Quantum Physics |
|---|---|---|
| Scope | Describes the behavior of macroscopic objects and systems, such as planets, cars, and projectiles. | Describes the behavior of microscopic particles, such as electrons, protons, and photons. |
| Key Theories | Newtonian mechanics, Maxwell’s equations, thermodynamics, classical electromagnetism. | Schrödinger equation, Heisenberg’s uncertainty principle, quantum field theory, quantum electrodynamics. |
| Determinism vs Probability | Deterministic; given initial conditions, future states can be precisely predicted. | Probabilistic; only the probabilities of outcomes can be predicted, not exact outcomes. |
| Wave-Particle Duality | Objects are treated either as particles or waves, but not both simultaneously. | Particles exhibit both wave-like and particle-like properties simultaneously. |
| Energy Levels | Energy can vary continuously. | Energy is quantized; particles exist in discrete energy states. |
| Superposition | Objects exist in a single, definite state at any given time. | Particles can exist in multiple states (superpositions) simultaneously until measured. |
| Measurement Effect | Measurement does not affect the state of the system. | Measurement affects the state of the system, collapsing it from a superposition to a definite state. |
| Non-Locality | Interactions are local; objects are only influenced by their immediate surroundings. | Entangled particles exhibit non-local correlations, affecting each other instantaneously over any distance. |
| Applications | Everyday phenomena, engineering, classical mechanics, electromagnetism. | Modern technology, such as semiconductors, lasers, MRI, quantum computing. |
FAQ’s
What is a quantum particle?
A quantum particle is a particle, like an electron or photon, that exhibits both wave-like and particle-like properties.
What is superposition?
Superposition is the principle where a quantum system can exist in multiple states simultaneously until measured.
What is entanglement?
Entanglement is a phenomenon where quantum particles become interconnected, such that the state of one instantly influences the state of another, regardless of distance.
What is the Heisenberg Uncertainty Principle?
The Heisenberg Uncertainty Principle states that it is impossible to simultaneously know both the exact position and momentum of a particle.
What is a quantum leap?
A quantum leap is the sudden change of an electron within an atom from one energy level to another.
What is a wave function?
A wave function is a mathematical description of the quantum state of a particle or system, containing information about probabilities of outcomes.
What is Schrödinger’s cat?
Schrödinger’s cat is a thought experiment illustrating superposition, where a cat in a box can be both alive and dead until observed.
What are qubits?
Qubits are the basic units of quantum information, capable of representing both 0 and 1 simultaneously due to superposition.
How does quantum physics differ from classical physics?
Quantum physics explains phenomena at atomic and subatomic levels, while classical physics describes macroscopic phenomena.
What is quantum decoherence?
Quantum decoherence is the process by which a quantum system loses its quantum behavior and transitions to classical behavior due to interactions with its environment.
Share :
Practice Test
Which quantum number determines the shape of an electron's orbital?
Principal Quantum Number
Magnetic Quantum Number
Azimuthal Quantum Number
Spin Quantum Number
of 10
What is the name of the equation that describes how the quantum state of a physical system changes over time?
Maxwell's Equations
Schrödinger Equation
Dirac Equation
Klein-Gordon Equation
of 10
In quantum mechanics, what is the term used to describe the discrete energy levels of an atom?
Energy Bands
Energy States
Quantum States
Quantum Levels
of 10
What phenomenon is explained by the photoelectric effect?
Particle-wave duality
Electron transitions between energy levels
Emission of electrons when light shines on a material
Interference of light waves
of 10
Which constant is fundamental to quantum mechanics and relates to the energy of a photon?
Gravitational Constant
Boltzmann Constant
Planck Constant
Speed of Light
of 10
What does the Pauli Exclusion Principle state about fermions?
No two fermions can occupy the same quantum state simultaneously
Fermions have no intrinsic angular momentum
Fermions always obey the Schrödinger Equation
Fermions can only exist in discrete energy levels
of 10
Which quantum number specifies the orientation of an orbital in space?
Principal Quantum Number
Azimuthal Quantum Number
Magnetic Quantum Number
Spin Quantum Number
of 10
What is the key feature of a quantum superposition state?
A particle can be in multiple states at once
Particles have zero energy
Quantum states are always deterministic
Particles are always in a single state
of 10
What principle explains why electrons in an atom occupy the lowest energy orbitals first?
Pauli Exclusion Principle
Hund's Rule
Aufbau Principle
Heisenberg Uncertainty Principle
of 10