What is the general formula for stress?
σ = F/A
σ = F*d
σ = F/m
σ = F/v
of 10
Stress Formula – Formula, Applications, example Problems
Stress Formula – Formula, Applications, example Problems
What is Stress Formula?
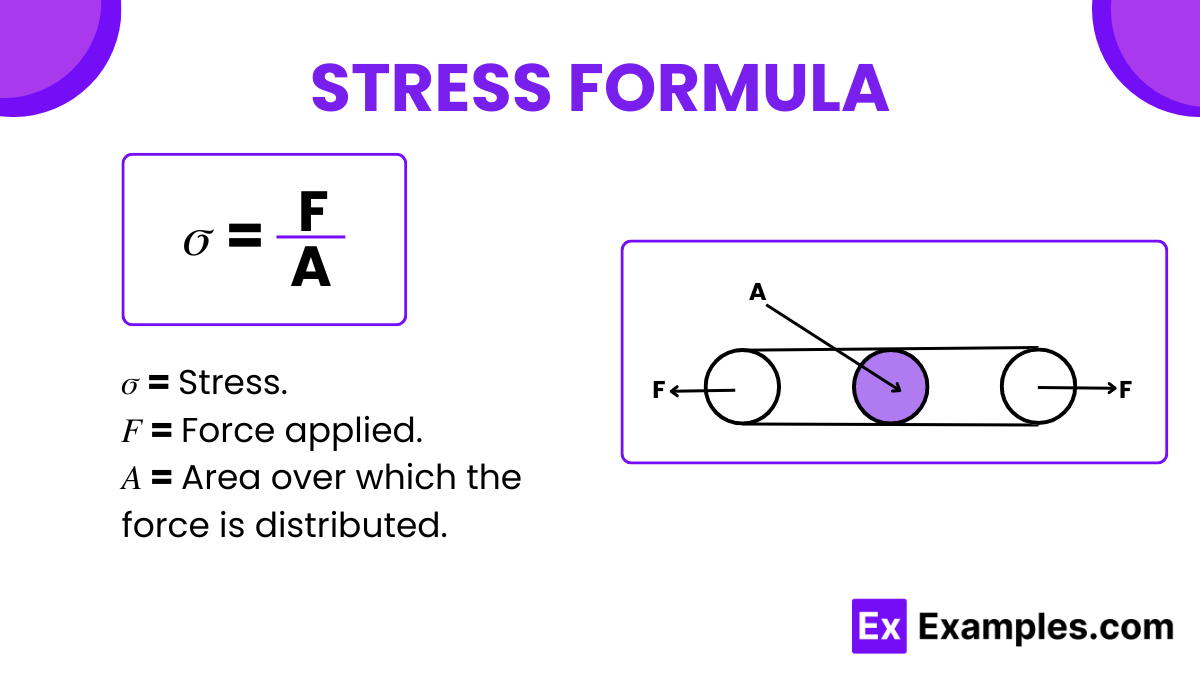
The stress formula in physics quantifies the amount of internal force exerted per unit area within a material. This formula is essential for understanding how materials deform under various forces, a fundamental concept in the field of physics and engineering. we express the formula for stress as
- 𝜎 represents the stress.
- 𝐹 is the force applied.
- 𝐴 is the area over which the force is distributed.
This concept was developed by Thomas Young, an English polymath, who first described it in the early 19th century. Young’s work laid the foundation for modern material science, particularly in understanding elasticity and structural integrity. The stress formula helps engineers and physicists predict how materials will behave when subjected to different forces, which is critical in designing safe and effective structures, from bridges to airplanes.
Applications of Stress Formula
- Building and Construction: Engineers calculate the stress on structural components like beams and columns to ensure they can support expected loads without failure.
- Material Science: Researchers use the stress formula to determine the breaking point of different materials, aiding in the development of stronger and more durable materials.
- Manufacturing: In industries such as automotive and aerospace manufacturing, the stress formula helps in designing parts that must withstand high forces without deforming.
- Safety Testing: Companies perform stress tests on products to simulate how they will perform under extreme conditions, ensuring they are safe for public use.
- Education and Training: Physics educators use the stress formula to teach students about the properties of materials and the principles of force and pressure.
Example Problems on Stress Formula
Problem 1: Calculating Stress in a Beam
Question: A force of 5000 Newtons is applied to a rectangular beam with a cross-sectional area of 0.1 square meters. Calculate the stress on the beam.
Solution: 𝜎 = 𝐹 / 𝐴 = 5000 N / 0.1 m² = 50000 Pa
The stress on the beam is 50000 Pascals.
Problem 2: Stress on a Wire
Question: A thin steel wire with a diameter of 0.5 mm is subjected to a tension force of 300 Newtons. Calculate the stress experienced by the wire.
Solution: First, calculate the cross-sectional area 𝐴 of the wire using the formula for the area of a circle, 𝐴=𝜋𝑟², Where r is the radius.
𝑟=0.5 mm / 2 =0.25 mm=0.00025 m
𝐴 = 𝜋(0.00025 m)² ≈ 1.963×10⁻⁷ m²
Now, apply the stress formula:
𝜎 = 𝐹𝐴 = 300 N / 1.963×10⁻⁷ m² ≈ 1.528 × 10⁹ Pa
The stress on the wire is approximately 1.528 GigaPascals (GPa).
Problem 3: Comparing Stress in Two Columns
Question: Two columns, one with a circular cross-section of radius 10 cm and another with a square cross-section of side 20 cm, each bear a load of 10000 Newtons. Which column experiences more stress?
Solution: Calculate the area for each column:
Circular column area 𝐴₁ = 𝜋𝑟²
𝑟=0.1 m
𝐴₁ = 𝜋(0.1 m)² = 0.0314 m²
Square column area 𝐴₂ = 𝑠²
𝑠 = 0.2 m
𝐴₂ = (0.2 m)² = 0.04 m²
Now, calculate the stress for each column:
Circular column: 𝜎₁ = 10000 N / 0.0314 m² ≈ 318471 Pa
Square column: 𝜎₂ = 10000 N / 0.04 m²=250000 Pa
The circular column experiences more stress than the square column because it has a smaller cross-sectional area.
FAQs
What is Stress and Its Unit?
Stress measures internal force per unit area within materials, quantified in Pascals (Pa).
What is the Formula for Total Stress?
To calculate total stress, use the formula 𝜎=𝐹𝐴, where 𝐹 represents the force and 𝐴 represents the area.
How Do You Calculate the Stress?
Calculate stress by dividing the force applied (𝐹) by the area (A): 𝜎=𝐹𝐴.
Share :
Practice Test
In the stress formula σ = F/A, what does F represent?
Force
Area
Area
Length
of 10
What is the unit of stress in the International System of Units (SI)?
Pascal (Pa)
Newton (N)
Joule (J)
Watt (W)
of 10
How does increasing the cross-sectional area (A) affect the stress (σ) for a given force (F)?
Stress increases
Stress decreases
Stress remains the same
Stress becomes zero
of 10
Which type of stress occurs when a material is stretched?
Compressive stress
Tensile stress
Shear stress
Thermal stress
of 10
What is compressive stress?
Stress that acts parallel to the surface
Stress that acts perpendicular to the surface and compresses the material
Stress that acts perpendicular to the surface and stretches the material
Stress that acts due to temperature changes
of 10
How is shear stress different from tensile stress?
Shear stress acts parallel to the surface, while tensile stress acts perpendicular
Shear stress acts perpendicular to the surface, while tensile stress acts parallel
Shear stress increases volume, while tensile stress reduces volume
Shear stress acts due to temperature, while tensile stress acts due to force
of 10
In which unit is shear stress typically measured?
Pascal (Pa)
Newton (N)
Joule (J)
Watt (W)
of 10
What happens to the stress in a material if the applied force is doubled while the cross-sectional area remains constant?
Stress is halved
Stress remains the same
Stress doubles
Stress becomes zero
of 10
What type of stress is experienced by a material when it is twisted?
Compressive stress
Tensile stress
Shear stress
Torsional stress
of 10