What is the SI unit of electrical resistance?
Ampere
Volt
Ohm
Coulomb
of 10
Units of Resistance – Examples, Definition, Units, Conversion Chart
Units of Resistance – Examples, Definition, Units, Conversion Chart
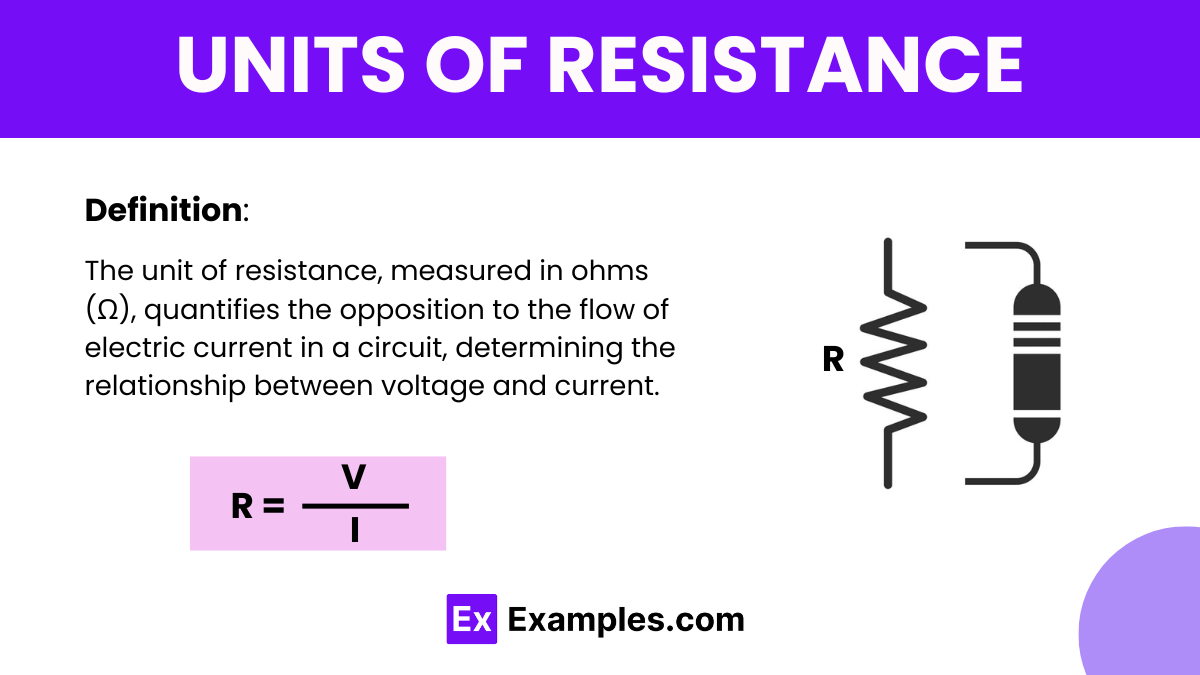
Resistance, a fundamental concept in physics, is the measure of an object’s opposition to the flow of electric current. In electrical circuits, it is represented by the symbol R and measured in ohms (Ω). Understanding units of resistance is crucial in designing and analyzing electronic circuits, as well as in various engineering and scientific applications.
What is Units of Resistance?
Units of Resistance Formula
Where:
- R is the resistance in ohms (Ω).
- V is the voltage in volts (V).
- I is the current in amperes (A).
SI Units of Resistance
The SI unit of resistance is the ohm (Ω). It is named after the German physicist Georg Simon Ohm and is represented by the symbol Ω. In electrical circuits, resistance is measured in ohms and indicates the degree to which a material opposes the flow of electric current. The ohm is a fundamental unit in electrical engineering, electronics, and physics, and is used extensively in analyzing and designing circuits and electrical systems.
CGS Unit of Resistance
In the CGS (centimeter-gram-second) system, the unit of resistance is called the “abohm” (symbol: abΩ). One abohm is equivalent to 10⁹ ohms in the SI system. The CGS system is less commonly used in modern science and engineering compared to the SI system, but the abohm remains a part of historical context in some areas of study.
List of Resistance Units
| Unit | Symbol | Equivalent to | Description |
|---|---|---|---|
| Kilohm (kΩ) | kΩ | 103 Ω | Equal to 1,000 ohms. |
| Megohm (MΩ) | MΩ | 106 Ω | Equal to 1,000,000 ohms. |
| Gigohm (GΩ) | GΩ | 109 Ω | Equal to 1,000,000,000 ohms. |
| Milliohm (mΩ) | mΩ | 10−3 Ω | Equal to 0.001 ohms. |
| Microohm (µΩ) | µΩ | 10−6 Ω | Equal to 0.000001 ohms. |
Kilohm (kΩ)
- The kilohm is equal to 1,000 ohms.
- It is commonly used to represent resistance values in electronic circuits where the resistance is relatively moderate.
Megohm (MΩ)
- The megohm is equal to 1,000,000 ohms.
- It represents very high resistance values, often encountered in high-impedance circuits, insulation resistance measurements, and biomedical applications.
Gigohm (GΩ)
- The gigohm is equal to 1,000,000,000 ohms.
- It represents extremely high resistance values and is used in specialized applications such as high-voltage insulation testing and electrostatic discharge (ESD) protection.
Milliohm (mΩ)
- The milliohm is equal to one-thousandth (0.001) of an ohm.
- It represents very low resistance values and is commonly used in power electronics, where minimizing resistance losses is critical.
Microohm (µΩ)
- The microohm is equal to one-millionth (0.000001) of an ohm.
- It represents extremely low resistance values, often encountered in high-current applications, such as power transmission lines and electrical contacts.
Conversion of Resistance Units
| To Unit | Conversion Factor | Example |
|---|---|---|
| Ohm (Ω) to Kilohm (kΩ) | 1 Ω = 10⁻³ kΩ | 10 Ω = 0.01 kΩ |
| Ohm (Ω) to Megohm (MΩ) | 1 Ω = 10⁻⁶ MΩ | 10 Ω = 0.00001 MΩ |
| Ohm (Ω) to Gigohm (GΩ) | 1 Ω = 10⁻⁹ GΩ | 10 Ω = 0.00000001 GΩ |
| Ohm (Ω) to Milliohm (mΩ) | 1 Ω = 10³ mΩ | 10 Ω = 10,000 mΩ |
| Ohm (Ω) to Microohm (μΩ) | 1 Ω = 10⁶ μΩ | 10 Ω = 10,000,000 μΩ |
Ohm (Ω) to Kilohm (kΩ)
- A kilohm is equal to 1,000 ohms.
- It is commonly used to represent resistance values in electronic circuits, where the resistance is relatively moderate.
- For example, a 10 ohm resistor is equivalent to 0.01 kilohms (10 Ω = 0.01 kΩ).
Ohm (Ω) to Megohm (MΩ)
- A megohm is equal to 1,000,000 ohms.
- It represents very high resistance values, often encountered in high-impedance circuits, insulation resistance measurements, and biomedical applications.
- For example, a 10 ohm resistor is equivalent to 0.00001 megohms (10 Ω = 0.00001 MΩ).
Ohm (Ω) to Gigohm (GΩ)
- A gigohm is equal to 1,000,000,000 ohms.
- It represents extremely high resistance values and is used in specialized applications such as high-voltage insulation testing and electrostatic discharge (ESD) protection.
- For example, a 10 ohm resistor is equivalent to 0.00000001 gigohms (10 Ω = 0.00000001 GΩ).
Ohm (Ω) to Milliohm (mΩ)
- A milliohm is equal to one-thousandth (0.001) of an ohm.
- It represents very low resistance values and is commonly used in power electronics, where minimizing resistance losses is critical.
- For example, a 10 ohm resistor is equivalent to 10,000 milliohms (10 Ω = 10,000 mΩ).
Ohm (Ω) to Microohm (μΩ)
- A microohm is equal to one-millionth (0.000001) of an ohm.
- It represents extremely low resistance values, often encountered in high-current applications such as power transmission lines and electrical contacts.
- For example, a 10 ohm resistor is equivalent to 10,000,000 microohms (10 Ω = 10,000,000 μΩ).
FAQs
What is the significance of milliohms and microohms?
Milliohms and microohms are important for measuring very low resistance values, such as those found in power electronics, electrical contacts, and high-current applications.
Why are different units of resistance necessary?
Different units of resistance provide flexibility in expressing resistance values across a wide range of scales, from small electronic components to high-voltage systems.
What are some practical applications of resistance units?
Resistance units are used in various electronic and electrical applications, such as designing circuits, calculating power dissipation, measuring sensor values, and determining the quality of conductive materials.
Share :
Practice Test
Which symbol represents the unit of resistance?
Ω
V
A
W
of 10
What is the resistance of a conductor if the voltage is 10 volts and the current is 2 amperes?
5 ohms
10 ohms
20 ohms
2 ohms
of 10
If a resistor has a resistance of 10 ohms, what is the current through it when a voltage of 20 volts is applied?
1 ampere
2 amperes
5 amperes
10 amperes
of 10
Which of the following materials typically has the highest electrical resistance?
Copper
Aluminum
Silver
Rubber
of 10
What is the unit of conductance, the reciprocal of resistance?
Henry
Farad
Siemens
Tesla
of 10
How is resistance measured?
Using a voltmeter
Using an ammeter
Using an ohmmeter
Using a thermometer
of 10
What happens to the resistance of a conductor if its length is doubled?
It remains the same
It is halved
It is doubled
It becomes zero
of 10
If the cross-sectional area of a conductor is doubled, what happens to its resistance?
It remains the same
It is halved
It is doubled
It becomes zero
of 10
What unit is used to express resistivity?
Ohm per meter
Ohm per square meter
Ohm per cubic meter
Ohm-meter
of 10