What is the formula to calculate the volume of a cube with side length \'a\'?
V = a²
V = 4/3πa³
V = a³
V = πa²

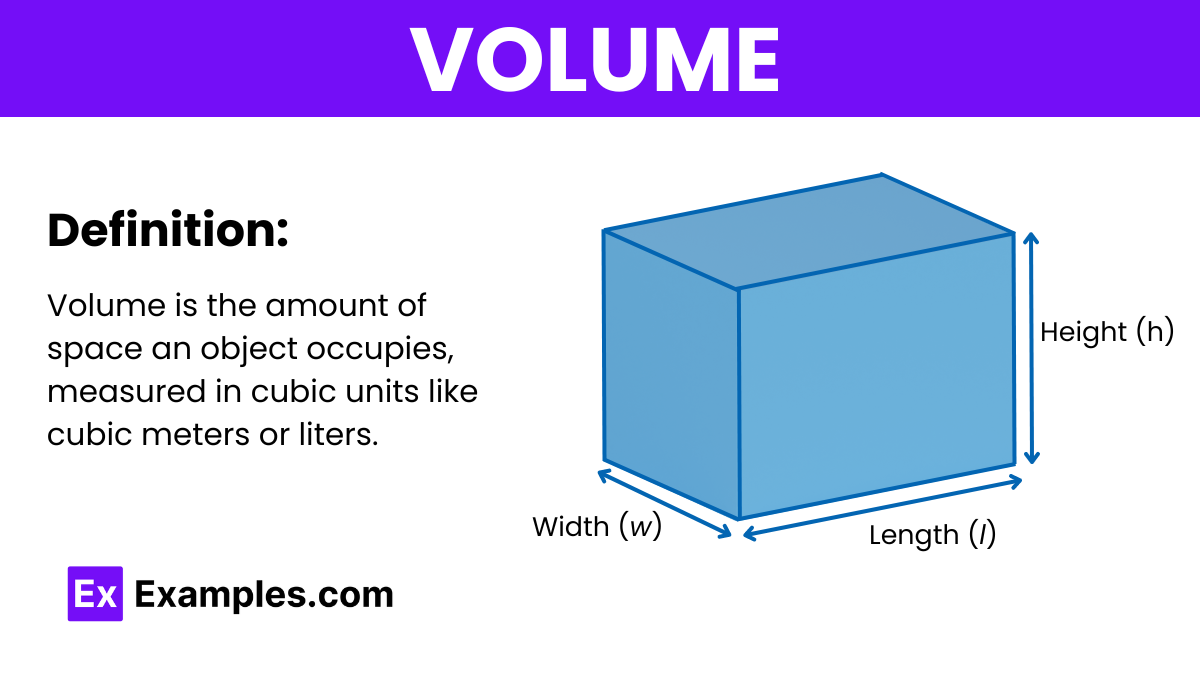
The volume of a solid object is measured in cubic units. This means that if you measure the dimensions (like length, width, and height) of an object in meters, then the volume will be expressed in cubic meters. Cubic meters are the standard unit of volume in the International System of Units (SI), which is used worldwide.
| Shape | Description | Volume Formula | Example |
|---|---|---|---|
| Cube | A solid with six equal square faces. | V = side³ | Side = 3m, V=27 m³ |
| Rectangular Prism | A box shape with six rectangular faces. | V = length × width × height | Length = 10m, Width = 4m, Height = 2m, V=80 m³ |
| Sphere | A perfectly round 3D object. | V = 4/3πradius³ | Radius = 2m, V≈33.51 m³ |
| Cylinder | A shape with two parallel circular bases. | V = πradius² × height | Radius = 1m, Height = 3m, V≈9.42 m³ |
| Cone | A shape with a circular base tapering to a point. | V = 1/3πradius² × height | Radius = 0.5m, Height = 2m, V≈0.52 m³ |
| Pyramid | A solid with a polygon base and triangular sides. | V = 1/3 × base area × height | Base side = 4m, Height = 9m, V=48 m³ |
Let’s say you want to find the volume of a right circular cylinder with a radius of 15 meters and a height of 3 meters. Use π = 3.14 for this calculation.
Solution:
| Unit | Description | Equivalent |
|---|---|---|
| Cubic Meter (m³) | Standard SI unit for volume, used in scientific and construction contexts. | 1 m³ = 1,000 liters |
| Liter (L) | Commonly used for liquids in everyday contexts. | 1 L = 1,000 milliliters (mL) |
| Milliliter (mL) | Often used in cooking and in laboratories. | 1 mL = 0.001 liters |
| Cubic Centimeter (cm³) | Common in medical and automotive applications. | 1 cm³ = 1 mL |
| Gallon (US) | Used in the United States for measuring larger quantities of liquids. | 1 US gallon ≈ 3.785 liters |
| Quart (US) | Smaller than a gallon, used for various liquid measurements in the US. | 1 quart = 0.25 US gallons |
| Pint (US) | Smaller unit used in cooking and some industries in the US. | 1 pint = 0.5 quarts |
| Cubic Inch (in³) | Used in manufacturing and shipping in the United States. | 1 in³ ≈ 16.387 cm³ |
| Cubic Foot (ft³) | Used in shipping, refrigeration, and architectural measurements. | 1 ft³ ≈ 28.317 liters |
| Barrel (oil) | Specifically for measuring volumes of crude oil. | 1 barrel ≈ 159 liters |
This table helps provide a clear and quick reference for converting and understanding different
The SI unit of volume is the cubic meter (m³). Smaller volumes may be measured in cubic centimeters (cm³) or liters.
Volume is typically calculated by the formula: Length × Width × Height for rectangular prisms. Different shapes have specific formulas based on their geometry.
Mass is not directly calculated from volume. However, mass can be determined by multiplying volume by density: Mass = Density × Volume.
Three common methods are: measuring dimensions and applying geometric formulas, displacement of water for irregular objects, and calculation through mass and density.

Volume is the amount of three-dimensional space occupied by an object, substance, or enclosed within a container. Commonly used in various fields such as science, engineering, and cooking. Volume is typically measured in cubic units like cubic meters (m³), liters (L), or cubic feet, depending on the context.
The volume of a solid object is measured in cubic units. This means that if you measure the dimensions (like length, width, and height) of an object in meters, then the volume will be expressed in cubic meters. Cubic meters are the standard unit of volume in the International System of Units (SI), which is used worldwide.
Shape Description: A cube is a solid with six equal square faces.
Volume Formula: Volume = side length × side length × side length
Example: If a cube has a side length of 3 meters, the volume would be 3 m×3 m×3 m = 27 m³. This could be a large storage box, where knowing the volume helps determine how much it can hold.
Shape Description: A rectangular prism is a box-shaped figure with six rectangular faces, where opposite faces are equal.
Volume Formula: Volume = length × width × height
Example: For a rectangular tank measuring 10 meters in length, 4 meters in width, and 2 meters in height, the volume is 10 m×4 m×2 m = 80 m³. This could represent the capacity of a water tank.
Shape Description: A sphere is a perfectly round geometrical object in three-dimensional space, like a ball.
Volume Formula: Volume = 4/3πradius³
Example: If a spherical balloon has a radius of 2 meters, its volume is 4/3 × π × 23 ≈ 33.51 m³. This indicates the amount of air needed to inflate the balloon.
Shape Description: A cylinder has two parallel circular bases connected by a curved surface at a right angle to the bases.
Volume Formula: Volume = π × radius² × height
Example: A cylindrical can with a radius of 1 meter and a height of 3 meters has a volume of π × 1² × 3 ≈ 9.42 m³, useful for calculating storage capacity.
Shape Description: A cone has a circular base connected by a curved surface to a single vertex.
Volume Formula: Volume = 1/3 π radius² height
Example: A cone-shaped party hat with a radius of 0.5 meters and a height of 2 meters has a volume of 1/3 × π × 0.52 × 2 ≈ 0.52 m³. This helps determine the amount of material needed to make the hat.
Shape Description: A pyramid has a polygon base and triangular sides that converge to a single point (the apex).
Volume Formula: Volume = 1/3 × base area × height
Example: If a pyramid has a square base with a side length of 4 meters and a height of 9 meters, assuming the area of the base is 4 m×4 m=16 m², the volume is 1/3 × 16 m² × 9 m = 48 m³. This can apply to architectural projects where understanding volume is critical for stability and material estimates.
Shape | Description | Volume Formula | Example |
|---|---|---|---|
Cube | A solid with six equal square faces. | V = side³ | Side = 3m, V=27 m³ |
Rectangular Prism | A box shape with six rectangular faces. | V = length × width × height | Length = 10m, Width = 4m, Height = 2m, V=80 m³ |
Sphere | A perfectly round 3D object. | V = 4/3πradius³ | Radius = 2m, V≈33.51 m³ |
Cylinder | A shape with two parallel circular bases. | V = πradius² × height | Radius = 1m, Height = 3m, V≈9.42 m³ |
Cone | A shape with a circular base tapering to a point. | V = 1/3πradius² × height | Radius = 0.5m, Height = 2m, V≈0.52 m³ |
Pyramid | A solid with a polygon base and triangular sides. | V = 1/3 × base area × height | Base side = 4m, Height = 9m, V=48 m³ |
Identify Necessary Parameters: First, find all the measurements you need for the formula, like radius (r), height (h), diameter, or slant height.
Check Units: Make sure all the measurements are in the same units (meters, centimeters, etc.).
Use the Formula: Put the measurements into the formula for the shape you’re calculating.
Write the Answer in Cubic Units: The result should be in cubic units, like cubic meters (m³).
Let’s say you want to find the volume of a right circular cylinder with a radius of 15 meters and a height of 3 meters. Use π = 3.14 for this calculation.
Solution:
Radius of the cylinder (r): 15 meters
Height of the cylinder (h): 3 meters
Volume formula for a cylinder: V=πr²h
Substitute the values: V=3.14×(15²)×3
Calculate 15²=225, so V=3.14×225×3=2119.5 m³
Result: The volume of the cylinder is 2119.5 cubic meters.
Unit | Description | Equivalent |
|---|---|---|
Cubic Meter (m³) | Standard SI unit for volume, used in scientific and construction contexts. | 1 m³ = 1,000 liters |
Liter (L) | Commonly used for liquids in everyday contexts. | 1 L = 1,000 milliliters (mL) |
Milliliter (mL) | Often used in cooking and in laboratories. | 1 mL = 0.001 liters |
Cubic Centimeter (cm³) | Common in medical and automotive applications. | 1 cm³ = 1 mL |
Gallon (US) | Used in the United States for measuring larger quantities of liquids. | 1 US gallon ≈ 3.785 liters |
Quart (US) | Smaller than a gallon, used for various liquid measurements in the US. | 1 quart = 0.25 US gallons |
Pint (US) | Smaller unit used in cooking and some industries in the US. | 1 pint = 0.5 quarts |
Cubic Inch (in³) | Used in manufacturing and shipping in the United States. | 1 in³ ≈ 16.387 cm³ |
Cubic Foot (ft³) | Used in shipping, refrigeration, and architectural measurements. | 1 ft³ ≈ 28.317 liters |
Barrel (oil) | Specifically for measuring volumes of crude oil. | 1 barrel ≈ 159 liters |
This table helps provide a clear and quick reference for converting and understanding different
The SI unit of volume is the cubic meter (m³). Smaller volumes may be measured in cubic centimeters (cm³) or liters.
Volume is typically calculated by the formula: Length × Width × Height for rectangular prisms. Different shapes have specific formulas based on their geometry.
Mass is not directly calculated from volume. However, mass can be determined by multiplying volume by density: Mass = Density × Volume.
Three common methods are: measuring dimensions and applying geometric formulas, displacement of water for irregular objects, and calculation through mass and density.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What is the formula to calculate the volume of a cube with side length \'a\'?
V = a²
V = 4/3πa³
V = a³
V = πa²
Which unit is commonly used to measure volume in the metric system?
Meters
Kilograms
Liters
Newtons
What is the volume of a rectangular prism with length 5 cm, width 3 cm, and height 4 cm?
60 cm³
12 cm³
20 cm³
30 cm³
How do you calculate the volume of a sphere with radius 'r'?
V = 4/3π
V = 4/3πr³
V = πr³
V = 2/3πr³
What is the volume of a cylinder with radius 2 cm and height 5 cm?
20π cm³
40π cm³
10π cm³
30π cm³
Which geometric shape has a volume calculated by the formula V = πr²h?
Sphere
Cube
Cone
Cylinder
What is the volume of a cone with base radius 3 cm and height 9 cm?
27π cm³
54π cm³
81π cm³
36π cm³
How is the volume of a rectangular tank with a length of 10 m, a width of 4 m, and a height of 2 m determined?
40 m³
80 m³
20 m³
60 m³
What is the volume of a pyramid with a base area of 16 m² and height 9 m?
48 m³
72 m³
144 m³
54 m³
How is the volume of a liquid typically measured in a laboratory?
Kilograms
Liters
Newtons
Meters
Before you leave, take our quick quiz to enhance your learning!

