Wiens Displacement Law

Wien’s Displacement Law describes the relationship between the temperature of a blackbody (an idealized object that absorbs all incident radiation) and the wavelength at which it emits radiation most strongly. In essence, as the temperature of the blackbody increases, the peak wavelength at which it emits light decreases, meaning that the color of the emitted light shifts to shorter wavelengths.

What is Wiens Displacement Law?

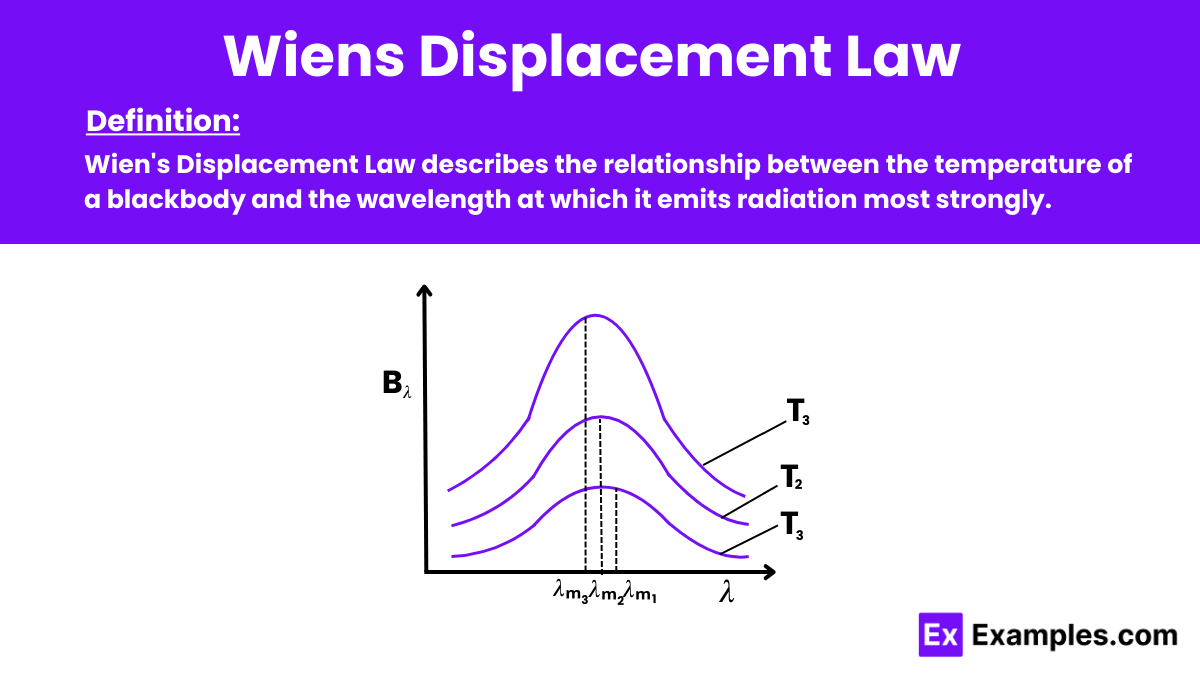
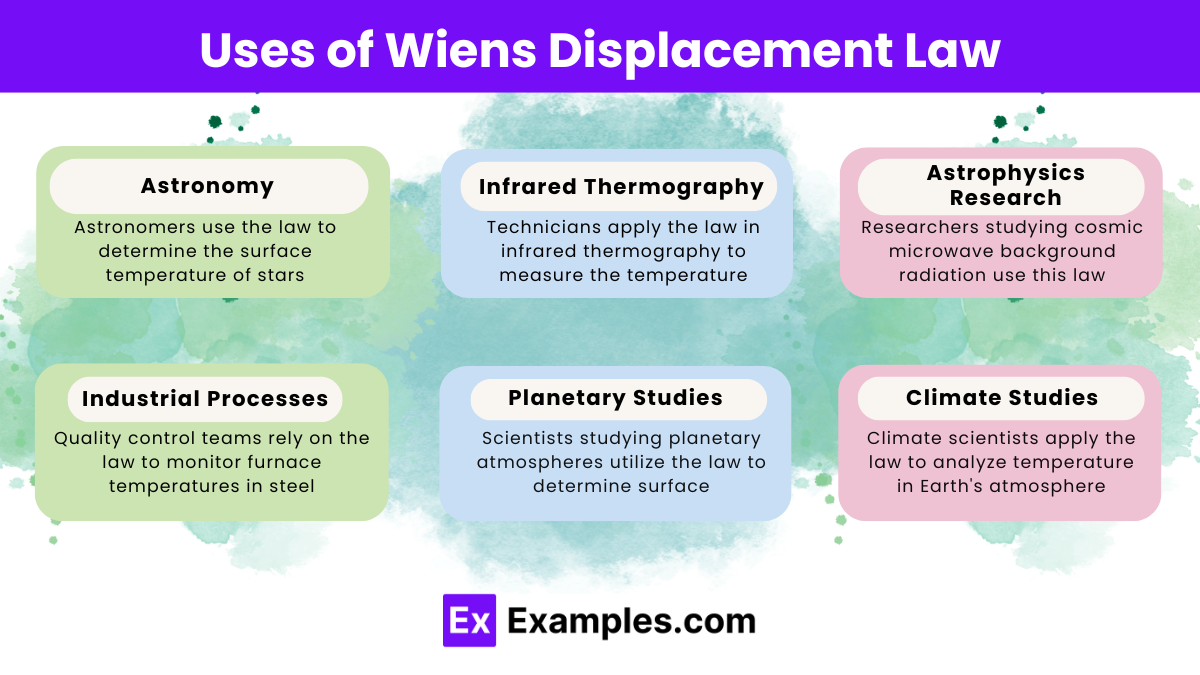
Wien’s Displacement Law is a principle in physics that describes the relationship between the temperature of a blackbody and the wavelength at which it emits the most radiation.

Wiens Displacement Law Formula

The formula for Wien’s Displacement Law is:

𝜆ₘₐₓ=𝑏𝑇​

where:

• 𝜆ₘₐₓ is the wavelength at which the radiation emitted by a blackbody is at its peak (in meters),
• 𝑇 is the absolute temperature of the blackbody (in kelvins),
• 𝑏 is Wien’s constant, approximately 2.897×10⁻³ m K.

This formula indicates that the peak wavelength decreases as the temperature increases, meaning that hotter objects emit light at shorter wavelengths.

Wien’s Displacement Constant

Wien’s Displacement Constant (often denoted as 𝑏) is a physical constant used in Wien’s Displacement Law to describe the relationship between the temperature of a blackbody and the wavelength at which it emits radiation most strongly. Its approximate value is:

𝑏=2.897×10⁻³ m K

This constant is used in the formula: 𝜆ₘₐₓ=𝑏𝑇​

Alternate ways of Wiens Displacement formula

Wien’s Displacement Law is primarily expressed as:

𝜆ₘₐₓ=𝑏𝑇​

• 𝜆ₘₐₓ is the peak wavelength,
• 𝑇 is the absolute temperature,
• 𝑏 is Wien’s constant (2.897×10⁻³ m K).

There are alternative ways to express this relationship:

In Terms of Frequency: Since frequency (𝑓) and wavelength (𝜆) are related through the speed of light (c), we can derive a formula involving the peak frequency (𝑓ₘₐₓ​):

𝑓ₘₐₓ = 𝑐/𝜆ₘₐₓ = 𝑐𝑇/𝑏​

• where:
• c is the speed of light (3×108 m/s)

In Energy Terms: Using Planck’s equation that relates the energy (𝐸) of a photon to its frequency:

𝐸ₘₐₓ=ℎ⋅𝑓ₘₐₓ=ℎ𝑐𝑇/𝑏

• ​where:
• ℎ is Planck’s constant (6.626×10⁻³⁴ J s),
• 𝑏 is Wien’s constant.

These alternate expressions allow the relationship to be examined in different ways, such as in terms of energy or frequency rather than wavelength.

Uses of Wiens Displacement Law

Wien’s Displacement Law plays a crucial role in several scientific and practical applications:

1. Astronomy: Astronomers use the law to determine the surface temperature of stars. By observing the wavelength at which a star emits the most light, they can estimate its temperature.
2. Infrared Thermography: Engineers and technicians apply the law in infrared thermography to measure the temperature of various surfaces. By analyzing the peak wavelength of the emitted infrared radiation, they can infer the surface temperature.
3. Astrophysics Research: Researchers studying cosmic microwave background radiation use Wien’s Displacement Law to better understand the universe’s temperature distribution.
4. Industrial Processes: Quality control teams rely on the law to monitor furnace temperatures in steel and glass manufacturing, ensuring consistent quality during production.
5. Planetary Studies: Scientists studying planetary atmospheres utilize the law to determine surface temperatures of planets and moons by analyzing the emitted radiation.
6. Climate Studies: Climate scientists apply the law to analyze temperature changes in Earth’s atmosphere. They use satellites to measure the peak infrared wavelengths emitted and determine global temperature patterns.

Examples for Wiens Displacement Law

1. Stars: When astronomers observe stars, they identify that hotter stars appear blue or white due to their shorter peak wavelengths. Conversely, cooler stars appear red, clearly illustrating the shift to longer wavelengths.
2. Sun’s Surface Temperature: The sun emits most of its radiation around a wavelength of 500 nm, which corresponds to visible light. Thus, applying Wien’s Displacement Law to this wavelength reveals a surface temperature of about 5,800 K.
3. Infrared Cameras: Infrared cameras detect thermal radiation from objects and identify their peak wavelengths. Consequently, by using Wien’s Displacement Law, they estimate the temperatures of these objects.
4. Furnaces: Industrial furnaces emit most of their radiation at infrared wavelengths due to their high temperatures. Therefore, engineers use the law to monitor furnace temperatures, ensuring optimal operation.
5. Cosmic Microwave Background: The cosmic microwave background radiation peaks at a wavelength of around 1.9 mm. Thus, scientists use the law to calculate the universe’s background temperature, which is about 2.7 K.

FAQ’S

Is Wien’s law accurate?

Wien’s Law is accurate for determining the peak wavelength of blackbody radiation at high temperatures. However, it is less accurate at lower temperatures, where Planck’s Law is more precise.

Why did Wien’s law fail?

Wien’s Law fails at lower temperatures because it doesn’t account for longer-wavelength radiation. Planck’s Law, which includes all wavelengths, better models the full spectrum in these cases.

How do astronomers use Wien’s law?

Astronomers use Wien’s Law to estimate the temperature of stars by analyzing the peak wavelength of their emitted light, revealing stellar temperatures from color shifts.