What is the formula for the power generated by wind energy?
P = 0.5ρAV³
P = 0.5ρAV²
P = 0.5ρA²V
P = 0.5ρV³
of 10
Wind Energy Formula – Formulas, Applications, Example Problems
Wind Energy Formula – Formulas, Applications, Example Problems
What is Wind Energy Formula?
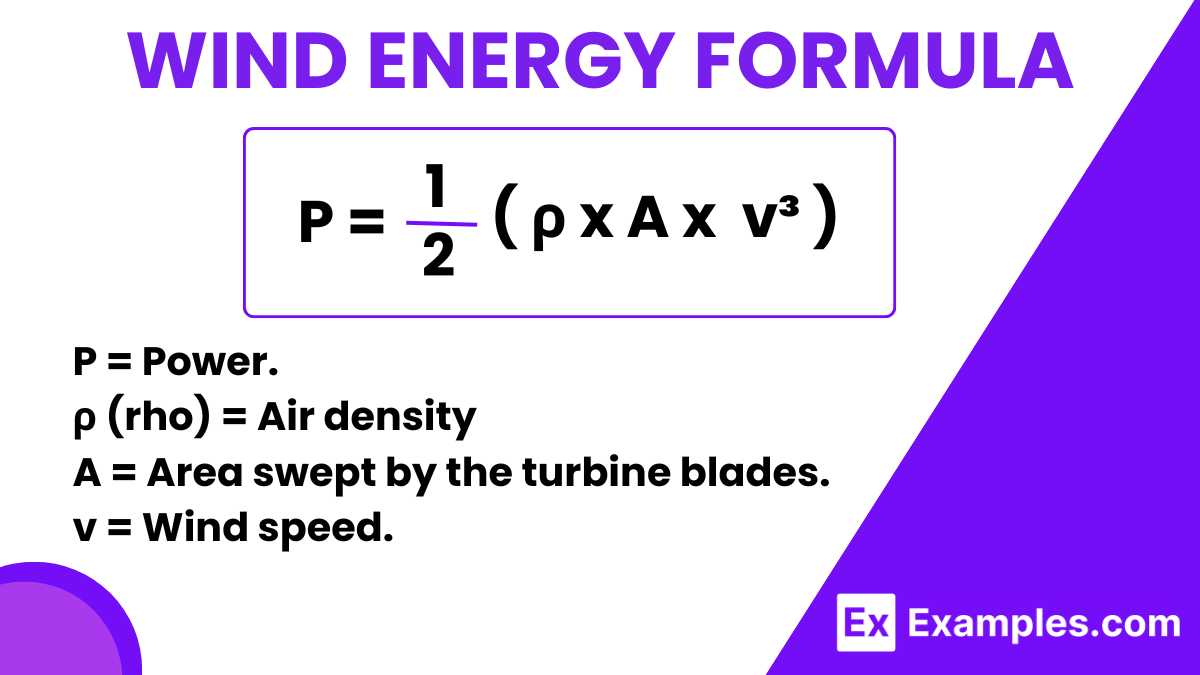
Wind energy is a vital renewable resource harnessed to generate electricity. At the core of understanding how wind power is converted into electrical power is the Wind Energy Formula. This formula is crucial in the field of physics as it helps predict how much power a wind turbine can generate based on certain environmental conditions. The formula for wind energy, derived from the principles of physics, is given by:
- ‘P’ stands for the power generated in watts.
- ‘ρ’ (rho) represents the air density in kilograms per cubic meter.
- ‘A’ is the area swept by the turbine blades in square meters.
- ‘v’ is the wind speed in meters per second.
This formula shows that the power produced by a wind turbine is dependent not only on wind speed but also on the density of the air and the size of the turbine blades.
The development of this formula is attributed to the work of physicist Albert Betz in 1919, who introduced Betz’s Law which limits the maximum achievable extraction of wind power by a turbine to 59.3%. Betz’s research significantly contributed to the optimization of turbine designs, enhancing the efficiency of wind energy conversion. The Wind Energy Formula is integral in the planning and development of wind farms by allowing engineers and scientists to estimate potential energy output, making it a cornerstone in the ongoing shift towards sustainable energy solutions.
Applications of Wind Energy Formula
- Designing Wind Turbines: Engineers use the formula to calculate the optimal size of the turbine blades and the tower height to maximize energy output based on local wind conditions.
- Evaluating Wind Farm Locations: Before establishing a wind farm, scientists apply the formula to assess the potential power output from different locations. This helps in selecting the most efficient sites for wind farms.
- Optimizing Turbine Performance: The formula assists in fine-tuning the operation of wind turbines, ensuring they operate efficiently at different wind speeds and air densities.
- Forecasting Energy Production: Energy companies use the formula to predict how much power their wind farms will produce. This is vital for planning the energy supply and integrating wind power with other energy sources.
- Educational Purposes: In academics, the formula is used to teach students about renewable energy technologies and the physics of energy conversion.
- Financial Analysis: Developers use the formula to predict the return on investment for wind energy projects by estimating potential energy generation.
Example Problems of Wind Energy Formula
Problem 1: Calculating Power Output with Basic Parameters
Given:
- Wind speed (v) = 10 m/s
- Blade area (A) = 30 m²
- Air density (ρ) = 1.225 kg/m³
Question: Calculate the power output of a wind turbine under these conditions using the Wind Energy Formula.
Solution: Use the formula P = ½ ρ A v³.
P = 0.5 × 1.225 kg/m³ × 30 m² × (10 m/s)³
P = 0.5 × 1.225 × 30 × 1000
P = 18375 Watts or 18.375 kW
Problem 2: Impact of Increasing Wind Speed
Given:
- Wind speed (v) = 15 m/s
- Blade area (A) = 30 m²
- Air density (ρ) = 1.225 kg/m³
Question: Calculate how much power is generated when the wind speed increases to 15 m/s.
Solution:
P = 0.5 × 1.225 kg/m³ × 30 m² × (15 m/s)³
P = 0.5 × 1.225 × 30 × 3375
P = 61875 Watts or 61.875 kW
Problem 3: Effect of Air Density on Power Output
Given:
- Wind speed (v) = 12 m/s
- Blade area (A) = 20 m²
- Air density (ρ) = 1.1 kg/m³
Question: How does a decrease in air density to 1.1 kg/m³ affect the power output?
Solution:
P = 0.5 × 1.1 kg/m³ × 20 m² × (12 m/s)³
P = 0.5 × 1.1 × 20 × 1728
P = 19008 Watts or 19.008 kW
FAQs
What is the Formula for Wind Power Energy?
The formula for wind power energy is P = ½ ρ A v³, where P is power, ρ is air density, A is blade area, and v is wind speed.
How to Calculate the Kinetic Energy of Wind?
Calculate wind’s kinetic energy using KE = ½ mv², where m is mass of air passing through per second and v is wind speed.
How to Measure Wind Energy?
Measure wind energy by assessing wind speed, air density, and turbine area to apply in the Wind Energy Formula.
Share :
Practice Test
What does the symbol ρ represent in the wind energy power formula?
Wind speed
Air density
Swept area
Power coefficient
of 10
If the wind speed doubles, how does the power generated by a wind turbine change?
It remains the same
It doubles
It quadruples
It increases eightfold
of 10
How does the swept area of a wind turbine affect the power generated?
It has no effect
It is inversely proportional to the power
It is directly proportional to the power
It is proportional to the square of the power
of 10
What is the typical range of air density (ρ) used in wind energy calculations?
0.1 to 0.2 kg/m³
1.0 to 1.2 kg/m³
10 to 12 kg/m³
100 to 120 kg/m³
of 10
What is the Betz limit in wind energy?
The maximum efficiency of a wind turbine
The minimum wind speed for power generation
The maximum wind speed a turbine can handle
The minimum swept area for power generation
of 10
What is the formula for calculating the swept area (A) of a wind turbine with radius (r)?
A = 2πr
A = πr²
A = 2πr²
A = πr³
of 10
If the radius of a wind turbine's blades is tripled, how does the swept area change?
It remains the same
It triples
It increases ninefold
It doubles
of 10
How does the power coefficient (Cp) affect the power output of a wind turbine?
It has no effect
It is inversely proportional to the power
It is directly proportional to the power
It reduces the power
of 10
If a wind turbine has a power coefficient (Cp) of 0.4, how efficient is the turbine?
40%
50%
60%
70%
of 10